Statement-I : A particle moves in a straight line with constant acceleration. The average velocity of this particle cannot be zero in any time interval.
Statement-II : For a particle moving in straight line with constant acceleration, the average velocity in a time interval is u+v2, where u and v are initial and final velocity of the particle of the given time interval.
Statement-I is true, Statement-II is true, Statement-II is correct explanation for Statement-I
Statement-I is true, Statement-II is true, Statement-II is NOT a correct explanation for Statement-I
Statement-I is true, Statement-II is false
Statement-I is false, Statement-II is true.
The correct Answer is:D
If displacement is zero in given time interval then its average velocity also will be zero. E.g. particle projects vertically upwards.
Topper's Solved these Questions
- KINEMATICSBOOK - ALLENCHAPTER - KINEMATICSEXERCISE - Comprehension#1
3 Videos
- KINEMATICSBOOK - ALLENCHAPTER - KINEMATICSEXERCISE - Comprehension#2
5 Videos
- KINEMATICSBOOK - ALLENCHAPTER - KINEMATICSEXERCISE - EXERCISE-03
6 Videos
- ERROR AND MEASUREMENTBOOK - ALLENCHAPTER - ERROR AND MEASUREMENTEXERCISE - Part-2(Exercise-2)(B)
22 Videos
- KINEMATICS (MOTION ALONG A STRAIGHT LINE AND MOTION IN A PLANE)BOOK - ALLENCHAPTER - KINEMATICS (MOTION ALONG A STRAIGHT LINE AND MOTION IN A PLANE)EXERCISE - BEGINNER S BOX-7
8 Videos
Similar Questions
A particle moves rectilinearly with initial velocity u and constant acceleration a. Find the average velocity of the particle in a time interval from t=0 to t=t second of its motion.
View SolutionA particle moves in a straight line with a uniform acceleration a. Initial velocity of the particle is zero. Find the average velocity of the particle in first 's' distance.
View Solution
Knowledge Check
If average velocity of particle moving on a straight line is zero in a time interval, then
AAcceleration of particle may be zeroBVelocity of particle must be zero at an instantCVelocity of particle may be never zero in the intervalDAverage speed of particle may be zero in the intervalAverage velocity of a particle moving in a straight line, with constant acceleration a and initial velocity u in first t seconds is.
Au+12atBu+atCu+at2Du2A particle is moving on a straight line and its average velocity is found to be zero in an interval of time.
AAverage speed of the particle may also be zero for a given interval of time.BVelocity of the particle can never be zero in given interval of time.CVelocity of the particle must be zero at a particular instant.DAcceleration of particle may be zero.
A particle moves in straight line. Acceleration of particle changes with velocity as showns in graph:
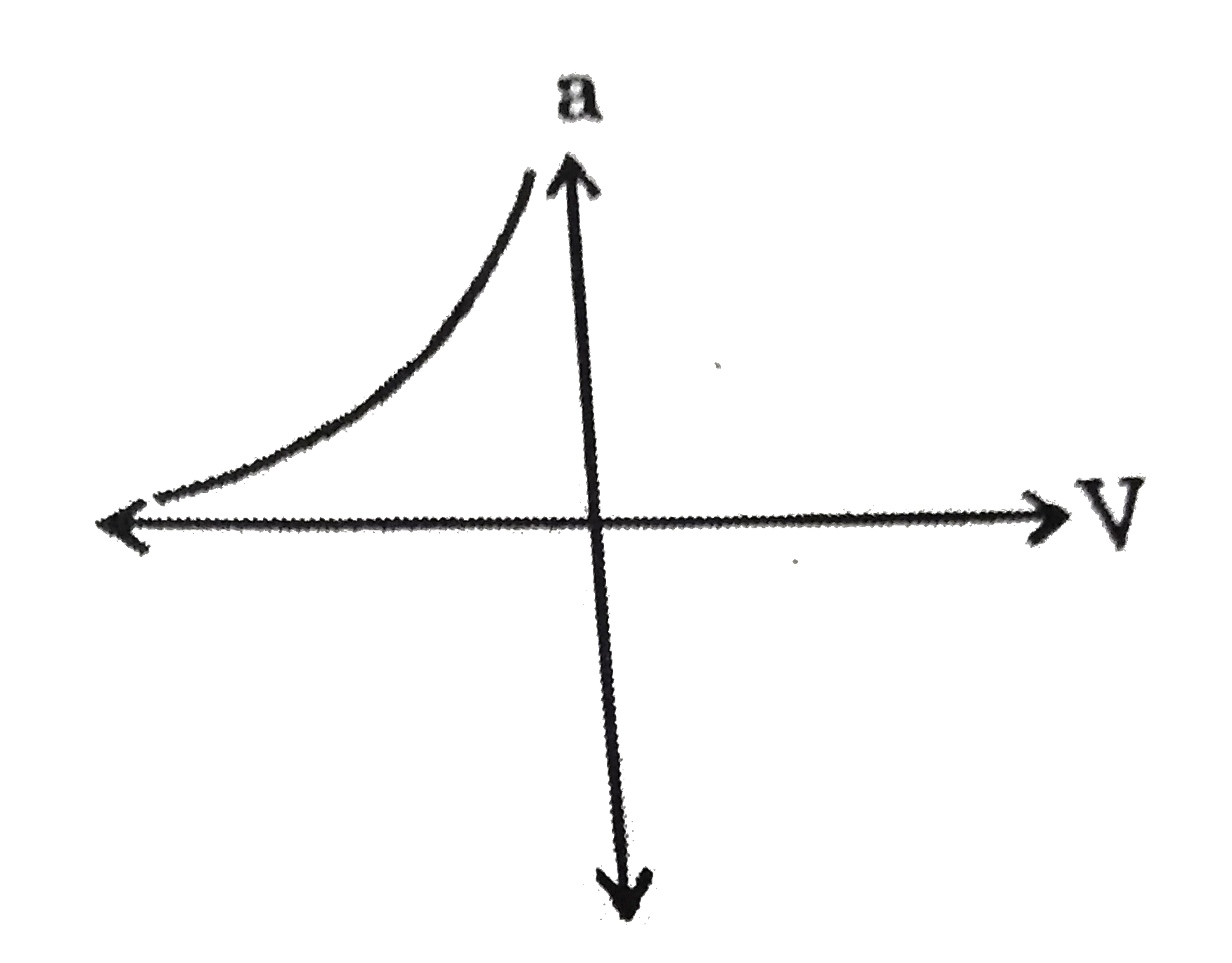
View SolutionA particle moves in a straight line with constant speed of 4m/s for 2s, then with 6m/s for 3s. Find the average speed of the particle in the given time interval.
View SolutionA particle of mass m is moving along the line y-b with constant acceleration a. The areal velocity of the position vector of the particle at time t is (u=0)
View SolutionThe velocity-time graph of a particle is not a straight line. Its acceleration is
View SolutionThe velocity time plot for a particle moving on straight line is shown in the figure.

View Solution
