Two identical small elastic balls have been suspended using two strings of different length (see fig (a)). Pendulum A is pulled to left by a small angle θ0 and released. It hits ball B head on which swings to angle 2θ0 from the vertical. Calculate the time period of oscillation of A if its length is known to be L.
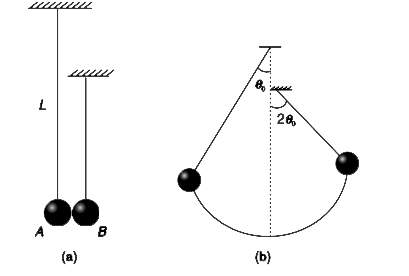
Step by step video, text & image solution for Two identical small elastic balls have been suspended using two strings of different length (see fig (a)). Pendulum A is pulled to left by a small angle theta_(0) and released. It hits ball B head on which swings to angle 2 theta _(0) from the vertical. Calculate the time period of oscillation of A if its length is known to be L. by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- SIMPLE HARMONIC MOTIONBOOK - ARIHANTCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Level 3
9 Videos
- SIMPLE HARMONIC MOTIONBOOK - ARIHANTCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Level 3
9 Videos
- ROTATIONAL MOTIONBOOK - ARIHANTCHAPTER - ROTATIONAL MOTIONEXERCISE - PHYSICS
159 Videos
- SURFACE TENSIONBOOK - ARIHANTCHAPTER - SURFACE TENSIONEXERCISE - Surface tension
33 Videos
Similar Questions
A simple pendulum of length 20cm and of mass 0.1kg is pulled to one side through an angle θ and released.If its maximum velocity is 1.4ms−1,the value of θ is ?
View SolutionTwo indentical pith balls, each carrying charge q, are suspended from a common point by two strings of equal length l. Find the mass of each ball if the angle between the strings is 2θ in equilibrium.
View Solution
Knowledge Check
A pendulum consists of a small mass m at the end of a string of length L. The pendulum is pulled aside making an angle θ with the vertical and released. Taking the suspension point as the axis, at the instant of release
Atorque on the pendulum is mgL cosθBtorque on the pendulum is mgL sinθCangular acceleration of pendulum is gcosθLDangular acceleration of pendulum is gsinθLA heavy & big sphere is hang with a string of length l. This sphere move in a horizontal circular path making an angle θ with vertical then its time period is
AT=2π√lgBT=2π√lsinθgCT=2π√lcosθgDT=2π√lgcosθA simple pendulum of length l is set in motion such that the bob, of mass m, moves along a horizontal circualar path , and the string makes a constant angle θ with the vertical. The time period of rotation of the bob is l and the tension in the thread is T
At=2π√l/gBt=2π√lcosθ/gCT=4π2mlt2DThe bob is in equilibrium
A coical pendulum consists of a string of length L whose upper end is fixed and another end is tied to a bob. The bob is moving in horizontal circle with constant angular speed ω such that the string makes a constant angle θ with the verticle. calculate time period T0 of revolution of bob in terms of L,g and θ.
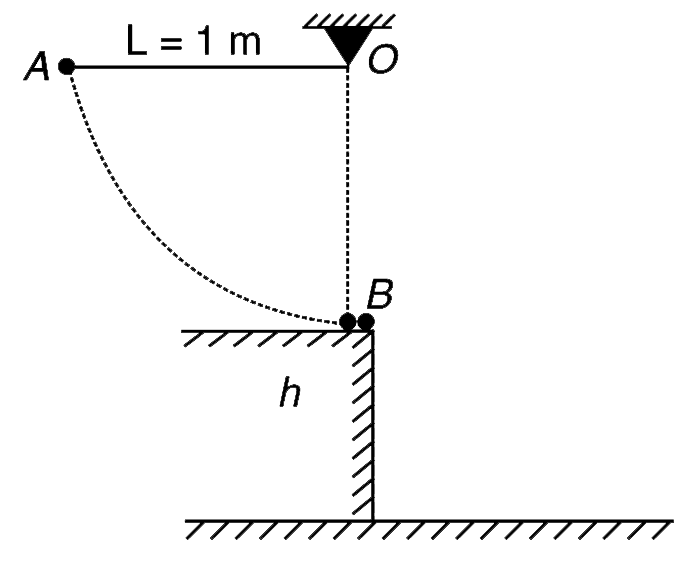
View Solution(i) A small steel ball (B) is at rest on the edge of a table of height h. Another identical steel ball (A) is tied to a light string of length L =1.0 m and is released from the position shown so that it swings like a pendulum. At the lowest position of its path it hits the ball B which is at rest. Ball B flies off the table and hits the ground in time t. After collision the ball A keeps moving for a time t before coming to rest for the first time. Find the value of h if t = t. Collision between the balls is head on and coefficient of restitution is e = 0.995.
(ii) A pendulum has a particle of mass m attached to a massless rod of length L. The rod is released from a position where it makes an angle θ0(>π2) with the vertical. The time period of oscillation is observed to be T0. Another similar pendulum has a rod of length 2L. Time period of this pendulum when released from position θ0 is T. Which is larger T or T0 ?
View SolutionTwo identical balls each having a density ρ are suspended from as common point by two insulating strings of equal length. Both the balls have equal mass and charge. In equilibrium each string makes an angle θ with vertical. Now, both the balls are immersed in a liquid. As a result the angle θ does not change. The density of the liquid is σ. Find the dielectric constant of the liquid.
View SolutionTwo identical spheres of mass m and charge q are suspended from a common point by two threads of the same length l and it is found that threads make an angle θ with the vertical when the spheres are in equilibrium . Calculate the value of q.
View SolutionA heavy small sized sphere is suspended by a string of length l . The sphere rotates uniformly in a horizontal circle with the string making an angle θ with the vertical. Then the time period of this conical pendulum is-
View Solution
