Two boys support by the ends a uniform rod of mass M and length 2L. The rod is horizontal. The two boys decided to change the ends of the rod by throwing the rod into air and catching it. The boys do not move from their position and the rod remained horizontal throughout its flight. Find the minimum impulse applied by each boy on the rod when it was thrown
The correct Answer is:M2√πLg3
To solve the problem of finding the minimum impulse applied by each boy on the rod when it is thrown, we can follow these steps:
Step 1: Understand the System
We have a uniform rod of mass M and length 2L held horizontally by two boys at its ends. When they throw the rod into the air, they apply impulses that will give the rod both upward velocity and angular velocity.
Hint: Visualize the rod and the forces acting on it. Draw a diagram to help understand the setup.
Step 2: Define Impulses
Let JH be the horizontal component of the impulse and JV be the vertical component of the impulse. The total impulse J can be expressed as:
J2=J2H+J2V
Hint: Remember that impulses are vector quantities; thus, they can be combined using the Pythagorean theorem.
Step 3: Relate Impulses to Motion
The vertical component JV will provide the upward velocity U of the center of mass (CM) of the rod, and the horizontal component JH will provide the angular speed ω of the rod.
From the equations of motion, we can relate these components:
- The time of flight T of the rod can be expressed as:
T=2Ug
- The angular displacement during this time is π radians, which gives:
ωT=π⟹ω=πg2U
Hint: Use the relationship between angular displacement, angular velocity, and time to find ω.
Step 4: Calculate Impulses
Using the moment of inertia ICM of the rod about its center of mass:
ICM=13M(2L)2=4ML23
The horizontal impulse JH can be related to the angular momentum:
JH=ICMω
Substituting for ω:
JH=4ML23⋅πg2U=2ML2πg3U
Hint: Recall the formula for the moment of inertia and how it relates to angular momentum.
Step 5: Express JV
From the vertical impulse, we have:
JV=MU
Hint: The vertical impulse directly relates to the change in momentum of the rod.
Step 6: Substitute and Minimize
Now substitute JH and JV into the equation for total impulse:
J2=(2ML2πg3U)2+(MU)2
To minimize J, we take the derivative with respect to U and set it to zero.
Hint: Use calculus to find the minimum value of a function by setting its derivative to zero.
Step 7: Solve for U
After differentiating and simplifying, we find:
U4=L2π2g236⟹U2=Lπg6
Hint: Keep track of your algebraic manipulations carefully to avoid mistakes.
Step 8: Calculate Minimum Impulse
Substituting U2 back into the impulse equation gives us:
J2min=M2L2π2g2144⋅Lπg6+M2Lπg4⋅6
After simplification, we find:
Jmin=MπLg6
Final Answer
The minimum impulse applied by each boy on the rod when it was thrown is:
Jmin=MπLg6
---
We have a uniform rod of mass M and length 2L held horizontally by two boys at its ends. When they throw the rod into the air, they apply impulses that will give the rod both upward velocity and angular velocity.
Let JH be the horizontal component of the impulse and JV be the vertical component of the impulse. The total impulse J can be expressed as:
J2=J2H+J2V
The vertical component JV will provide the upward velocity U of the center of mass (CM) of the rod, and the horizontal component JH will provide the angular speed ω of the rod.
- The time of flight T of the rod can be expressed as:
T=2Ug
- The angular displacement during this time is π radians, which gives:
ωT=π⟹ω=πg2U
Using the moment of inertia ICM of the rod about its center of mass:
ICM=13M(2L)2=4ML23
The horizontal impulse JH can be related to the angular momentum:
JH=ICMω
Substituting for ω:
JH=4ML23⋅πg2U=2ML2πg3U
From the vertical impulse, we have:
JV=MU
Now substitute JH and JV into the equation for total impulse:
J2=(2ML2πg3U)2+(MU)2
To minimize J, we take the derivative with respect to U and set it to zero.
After differentiating and simplifying, we find:
U4=L2π2g236⟹U2=Lπg6
Substituting U2 back into the impulse equation gives us:
J2min=M2L2π2g2144⋅Lπg6+M2Lπg4⋅6
After simplification, we find:
Jmin=MπLg6
The minimum impulse applied by each boy on the rod when it was thrown is:
Jmin=MπLg6
Topper's Solved these Questions
Similar Questions
A uniform rod AB of mass m and length l is at rest on a smooth horizontal surface. An impulse J is applied to the end B perpendicular to the rod in horizontal direction. Speed of the point A of the rod after giving impulse is:
View SolutionA uniform rod of mass m and length l rests on a smooth horizontal surface. One of the ends of the rod is struck in a horizontal direction at right angles to the rod. As a result the rod obtains velocity v0. Find the force with which one-half of the rod will act ont he other in the process of motion.
View Solution
Knowledge Check
A uniform rod AB of mass m and length l at rest on a smooth horizontal surface. An impulse P is applied to the end B. The time taken by the rod to turn through a right angle is :
 A2πmlPBπml3PCπml12PD2πml3P
A2πmlPBπml3PCπml12PD2πml3PA uniform rod AB of mass m and length l is at rest on a smooth horizontal surface. An impulse p is applied to the end B. The time taken by the rod to turn through a right angle is
A(2πmlpB(2π)(pmlCπml12pDπpmlA uniform rod AB of mass m and length l at rest on a smooth horizontal surface . An impulse P is applied to the end B. The time taken by the rod to turn through a right angle is :
 A2πmlPBπml3PCπml12PD2πml3P
A2πmlPBπml3PCπml12PD2πml3P
A rod AB of mass M and length L is shown in figure. End A of rod is hinged and end B is lying on the ground. Find the
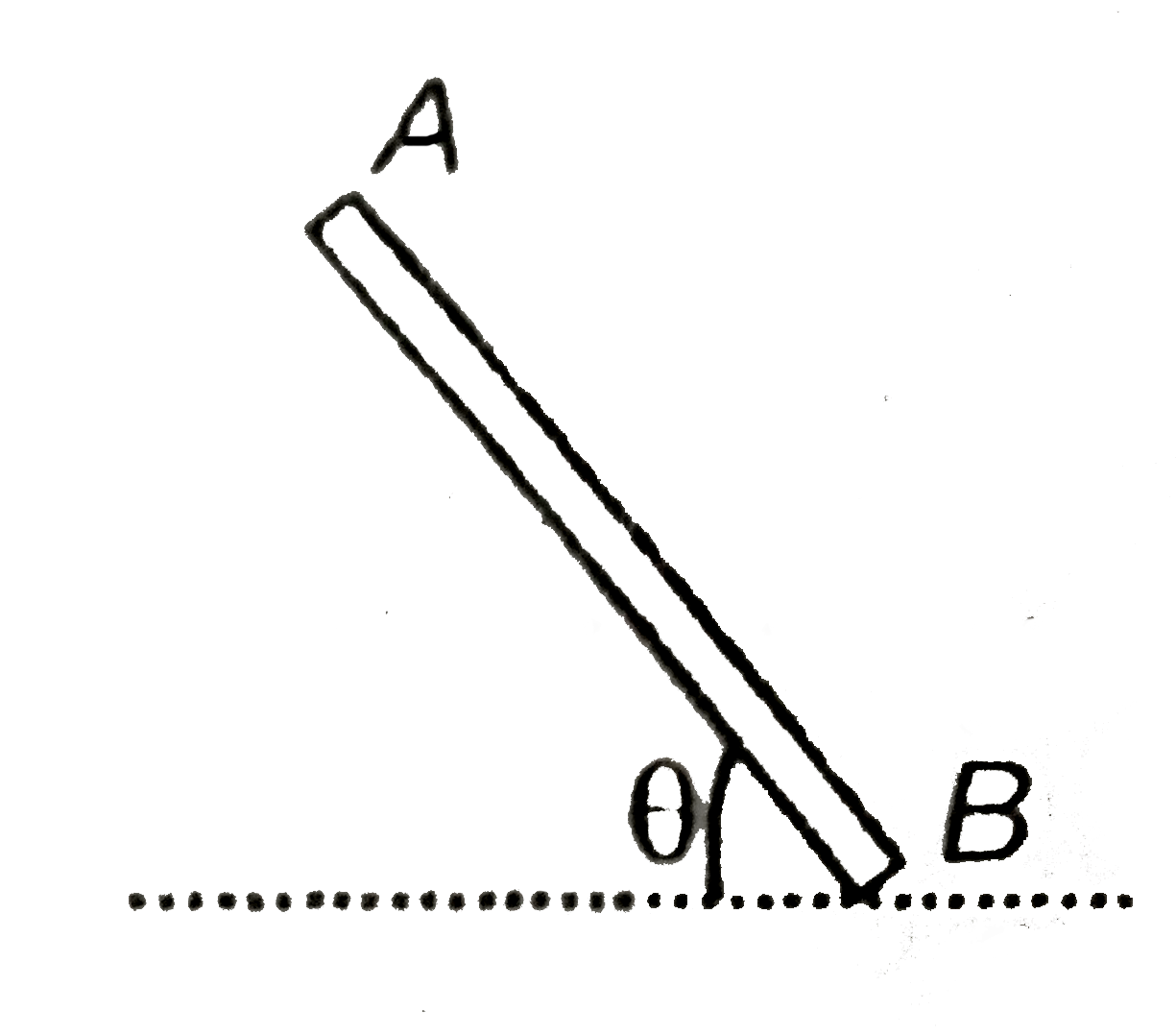
Normal reaction by ground on the rod
View SolutionA uniform rod of mass m and length l is on the smooth horizontal surface. When a constant force F is applied at one end of the rod for a small time t as shown in the figure. Find the angular velocity of the rod about its centre of mass.
View SolutionA uniform rod of mass M and length L is hinged at its end. The rod is released from its vertical position by slightly pushing it. What is the reaction at the hinge when the rod becomes horizontal, again vertical.
View SolutionA rod AB of mass m and length L is rotating in horizontal plane about one of its ends which is pivoted. The constant angular speed with which the rod is rotating is ω. The force applied on the rod by the pivot is
View SolutionA uniform rod of mass 20 Kg and length 1.6 m is piovted at its end and swings freely in the vertical plane. Angular acceleration of the rod just after the rod is relased from rest in the horizontal position is

View Solution
