Two liquid A & B having densities 2ρ and ρ respectively, are kept in a cylindrical container separated by a partition as shown in figure. The height of each liquid in the container is h and area of cross section of the container is A. Now the partition is removed. Calculate change in gravitational potential energy (ΔU) of the system (a) assuming that the two liquids mix uniformly. (b) Assuming that the two liquids are immiscible. What do you conclude from the sign of ΔU in the above two cases?
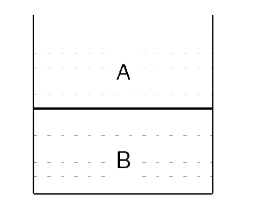
The correct Answer is:(a)12ρAh2g
(b) −ρAh2g
The positive sign of ΔU means external work will be required to mix the two liquids uniformly. ΔU is negative in second case which means the heavier liquid will automatically move to lower side.
Step by step video, text & image solution for Two liquid A & B having densities 2rho and rho respectively, are kept in a cylindrical container separated by a partition as shown in figure. The height of each liquid in the container is h and area of cross section of the container is A. Now the partition is removed. Calculate change in gravitational potential energy (DeltaU) of the system (a) assuming that the two liquids mix uniformly. (b) Assuming that the two liquids are immiscible. What do you conclude from the sign of DeltaU in the above two cases? by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
Two liquids of densities ρ and 3rho having volumes 3V and V are mixed together. Find density of the mixture.
View SolutionEqual volume of a liquid is poured into containers A and B where area of cross-section of container A is double the area of cross-section of container B. If PAandPB are pressures exerted at the bottom of the containers, then find PA:PB.
View Solution
Knowledge Check
The liquid inside the container has density ρ. Choose the correct option.
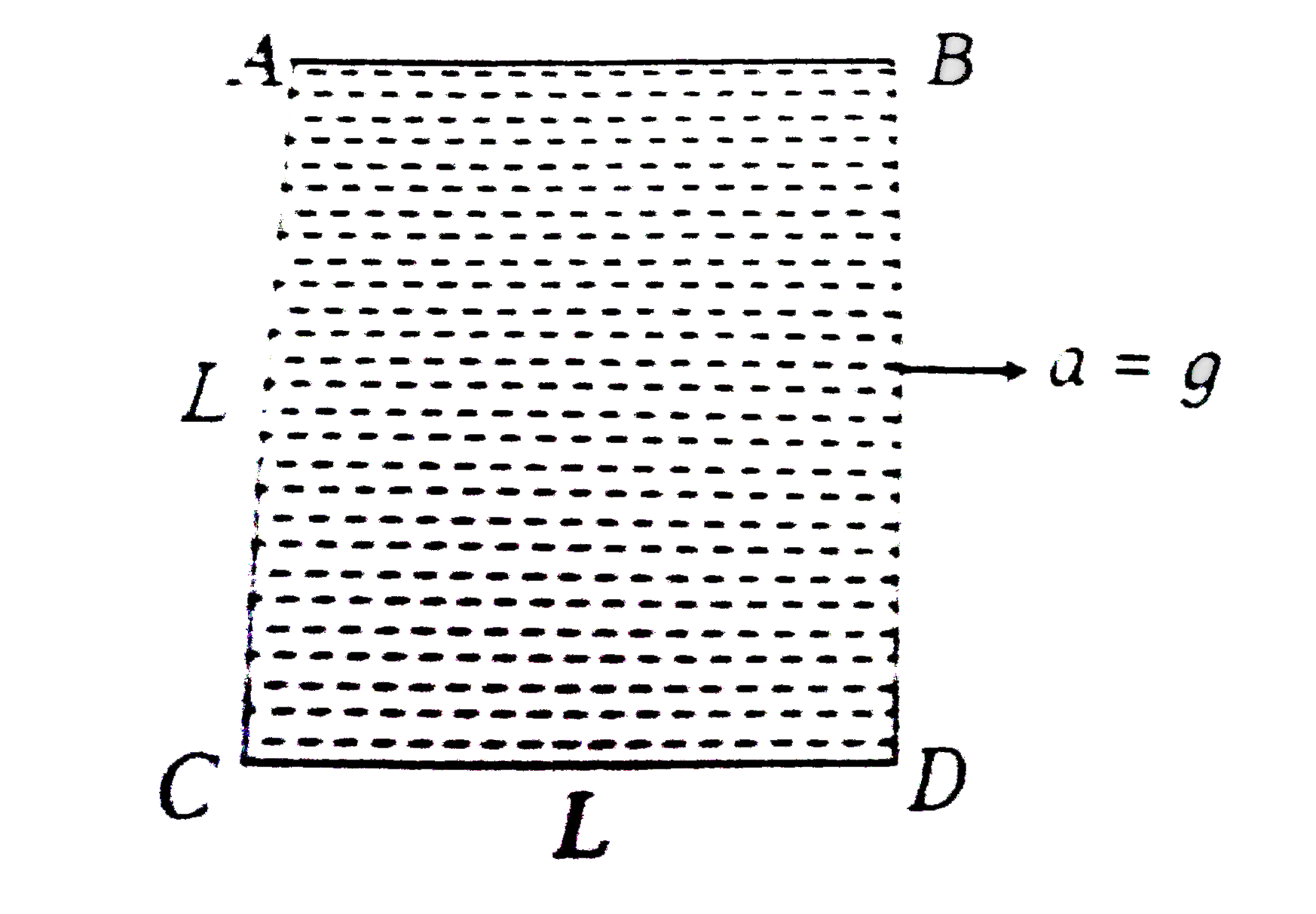 ApA−pC=2ρgLBpC−pB=√2ρgLCpC−pD=ρgLDpA−pD=0ρgL
ApA−pC=2ρgLBpC−pB=√2ρgLCpC−pD=ρgLDpA−pD=0ρgLIf two liquids of same mass but densities ρ1 and ρ2 respectively are mixed, then the density of the mixture is:
Aρ=ρ1+ρ22Bρ=ρ1+ρ22ρ1ρ2Cρ=2ρ1ρ2ρ1+ρ2Dρ=ρ1ρ2ρ1+ρ2If two liquids of same masses but densities ρ1andρ2 respectively are mixed, then the density of mixture is given by
Aρ=ρ1+ρ22Bρ=ρ1+ρ22ρ1ρ2Cρ=2ρ1ρ2ρ1+ρ2Dρ=ρ1ρ2ρ1+ρ2
If two liquids of same masses but densities ρ1andρ2, respectively are mixed, then the density of mixture is given by
View SolutionA cylindrical vessel contains a liquid of density ρ up to height h. The liquid is closed by a piston of mass m and area of cross section A. There is a small hole at the bottom of the vessel. The speed v with which the liquid comes out of the hole is

View SolutionLiquid of density ρ in a container exerts pressure P given by (height of liquid h and area A)
View SolutionA cylindrical vessel contains a liquid of density ρ up to height h. The liquid is closed by a piston of mass m and area of cross section A. There is a small hole at the bottom of the vessel. The speed v with which the liquid comes out of the hole is

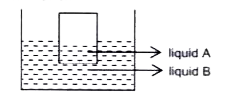
View SolutionA container filled with a liquid A is floating on the surface of another liquid 'B' as shown in the figure, then volume of the liquid displaced by the container
View Solution
