One mole of helium gas follow cycle 1−2−3−1 shown in the diagram. During process 3−1, the internal energy (U) of the gas depends on its volume (V) as U=bV2, where b is a positive constant. If gas releases the amount of heat Q during process 3−1 and gas absorbs the amount of heat Q2 during process 1→2→3. 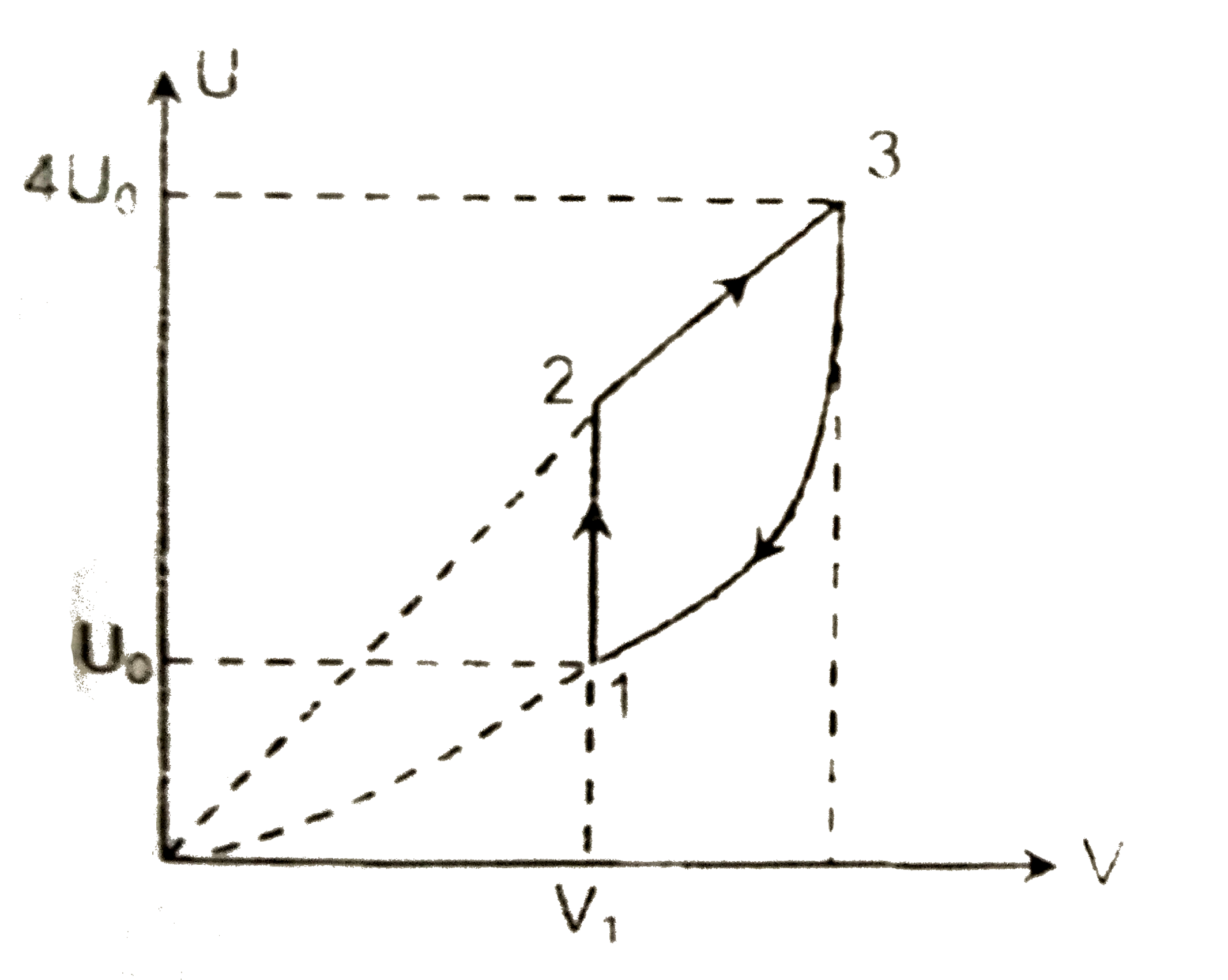
The value of ratio of volume of gas in state −3(V3) to that of gas in state −1(V1) is:
12
21
13
None of these.
The correct Answer is:B
Step by step video, text & image solution for One mole of helium gas follow cycle 1-2-3-1 shown in the diagram. During process 3-1, the internal energy (U) of the gas depends on its volume (V) as U=bV^(2), where b is a positive constant. If gas releases the amount of heat Q during process 3-1 and gas absorbs the amount of heat Q_(2) during process 1rarr2rarr3. The value of ratio of volume of gas in state -3(V_(3)) to that of gas in state -1(V_(1)) is: by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- TEST PAPERSBOOK - RESONANCECHAPTER - TEST PAPERSEXERCISE - PT-01
30 Videos
- TEST PAPERSBOOK - RESONANCECHAPTER - TEST PAPERSEXERCISE - Pt-02
30 Videos
- TEST PAPERSBOOK - RESONANCECHAPTER - TEST PAPERSEXERCISE - PART - II PHYSICS
107 Videos
- SIMPLE HARMONIC MOTION BOOK - RESONANCECHAPTER - SIMPLE HARMONIC MOTION EXERCISE - Advanced Level Problems
13 Videos
- TEST SERIESBOOK - RESONANCECHAPTER - TEST SERIESEXERCISE - PHYSICS
127 Videos
Similar Questions
An ideal gas undergoes a thermodynamic process in which internal energy of the gas depends on pressure of the gas as U= ap!, where a is a positive constant. Assuming gas to be monoatomic, the molar heat capacity of the gas for given process will be
View SolutionAn ideal gas undergoes a thermodynamic process in which internal energy of the gas depends on pressure of the gas as U=ap4, where a is a positive constant. Assuming gas to be monoatomic, the molar heat capacity of the gas for given process will be
View Solution
Knowledge Check
One mole of helium gas follows the cycle 1-2-3-1 shown in the diagram. During process 3-1, the internal energy (U) of the gas depends on its volume (V) as U=bV2, where b is a positive constant. If gas releases the amount of heat Q1 during process 3-1 and gas absorbs th amount of heat Q2 during process 1→2→3 them:
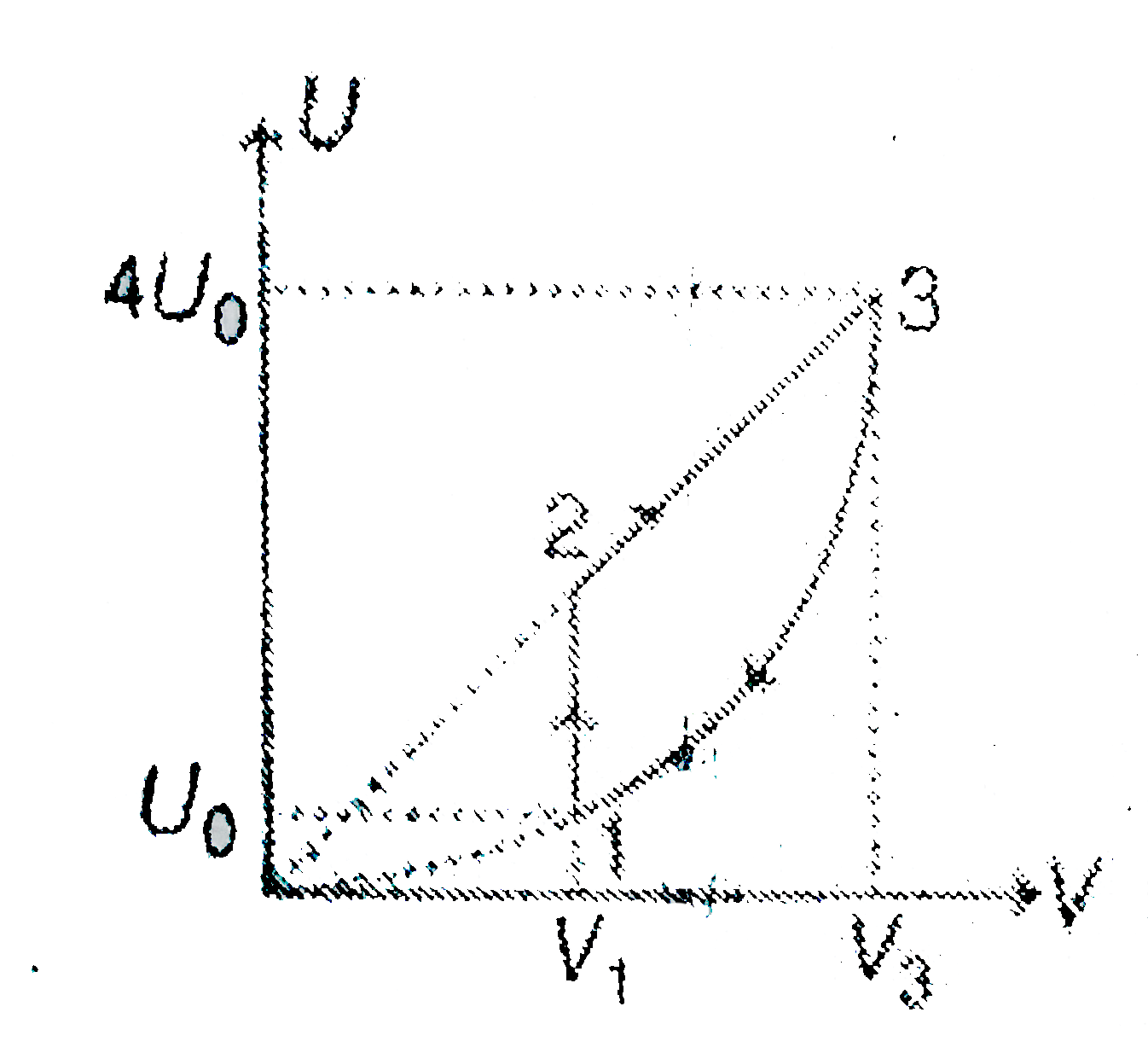
The value of O1/Q2 isA89B98C1213D1312One mole of helium gas follows the cycle 1-2-3-1 shown in the diagram. During process 3-1, the internal energy (U) of the gas depends on its volume (V) as U=bV2, where b is a positive constant. If gas releases the amount of heat Q1 during process 3-1 and gas absorbs th amount of heat Q2 during process 1→2→3 them:
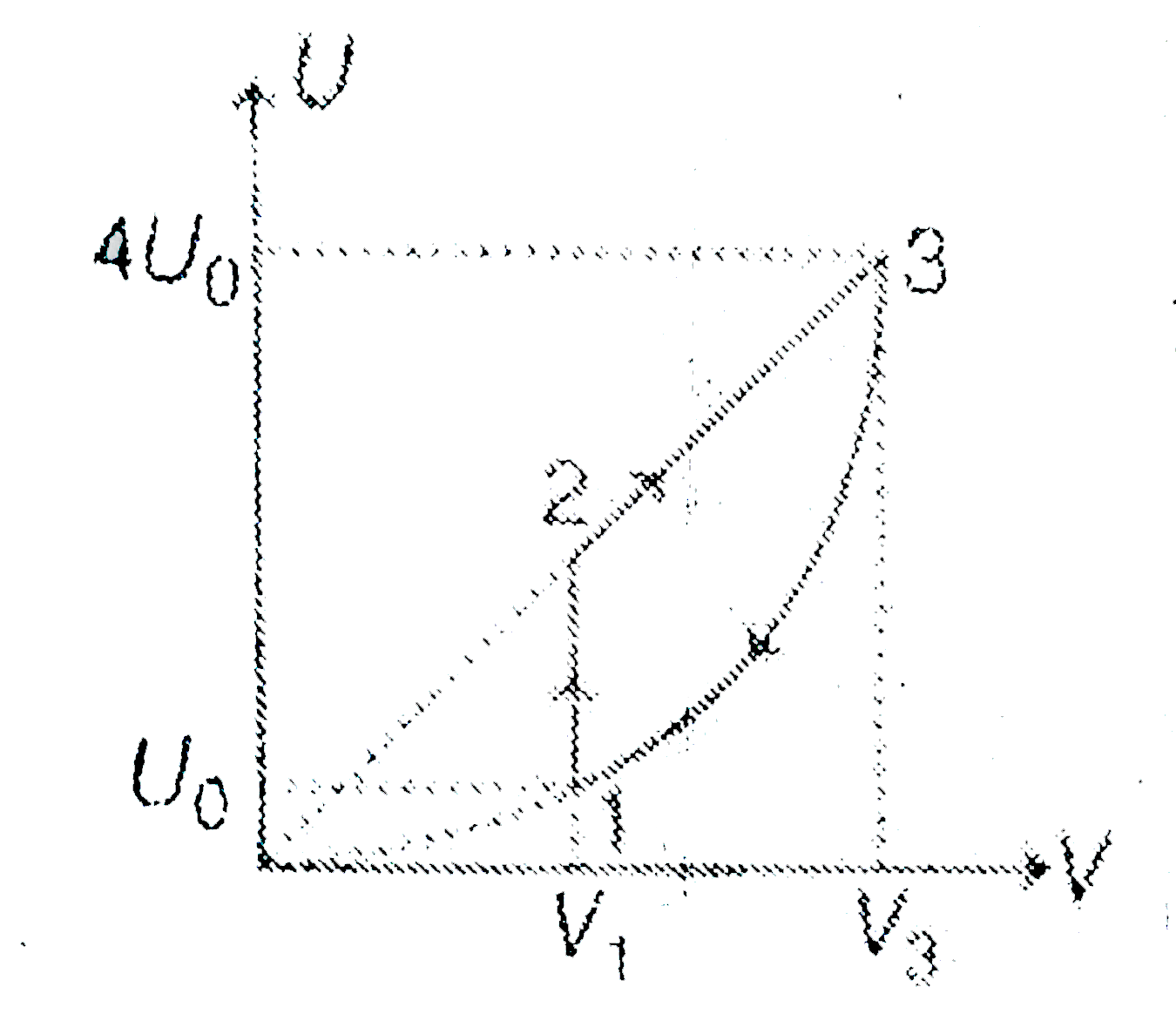
V3V1 isA43B21C32D41The temperature (T) of one mole of an ideal gas varies with its volume (V) as T=−αV3+βV2, where αandβ are positive constants. The maximum pressure of gas during this process is
Aαβ2RBβ2R4αC(α+β)R2β2Dα2R2β
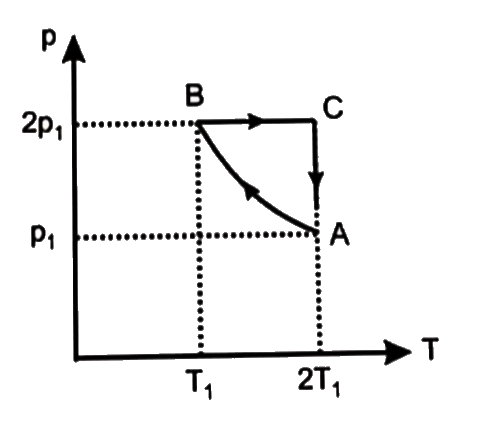
Two moles of an ideal monoatomic gas is taken through a cycle ABCA as shown in the p - T diagram. During the process AB, the pressure and temperature of the gas vary such that pT = constant. If T1=300K and the amount of heat released by the gas during the process AB is Q=−xR, then the value of x is
View SolutionAn ideal gas undergoes a four step cycle as shown in the P-V diagram below. During this cycle heat is absorbed by the gas in
View SolutionOne mole of a monoatomic ideal gas is expanded by a process described by PV3 = C where C is a constant. The heat capacity of the gas during the process is given by (R is the gas constant)
View SolutionTwo moles of an ideal gas are undergone a cyclic process 1-2-3-1. If net heat exchange in the process is 300J, the work done by the gas in the process 2-3 is

View SolutionDuring the adiabatic expansion of 2 moles of a gas, the internal energy of the gas is found to decrease by 2 joules , the work done during the process on the gas will be equal to
View Solution
