Question

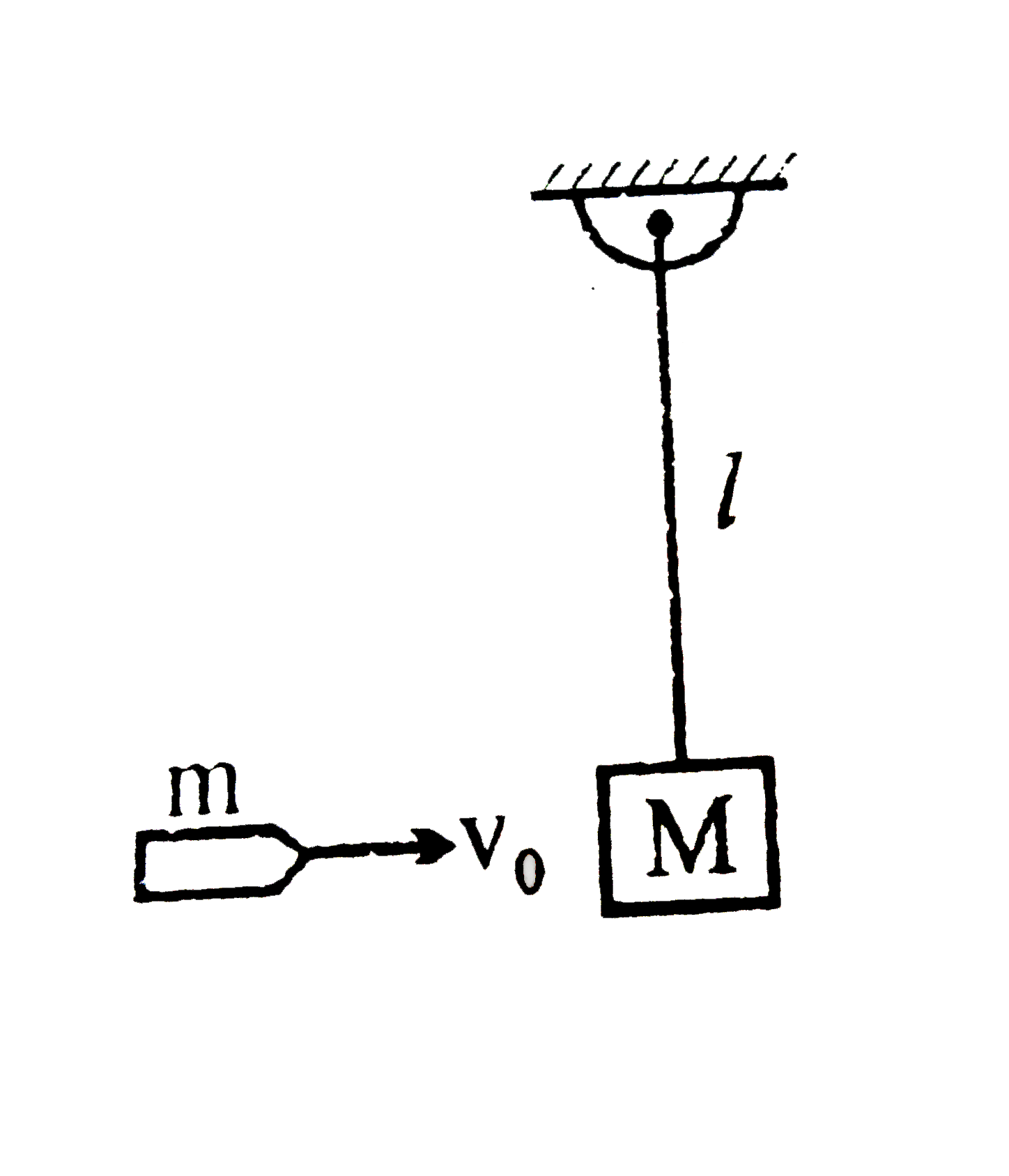
A bullet of mass m=50 gm strikes $(\Delta t\approx 0)$a sand bag of mass M =5 kg hanging from a fixed point, with a horizontal velocity ${\overrightarrow{v}}_p$. If bullet sticks to the sand bag then the ratio of final & initial kinetic energy of the bullet is

Options

A. `10^(-2)`
B. `10^(-3)`
C. `10^(-6)`
D. `10^(-4)`

Answer

D

Explanation

To solve the problem, we need to determine the ratio of the final kinetic energy to the initial kinetic energy of the bullet after it strikes the sandbag and sticks to it.

1. Identify the masses and initial conditions:
- Mass of the bullet, \( m = 50 \, \text{g} = 0.050 \, \text{kg} \)
- Mass of the sandbag, \( M = 5 \, \text{kg} \)
- Initial velocity of the bullet, \( v_p \) (horizontal velocity)
- Initial velocity of the sandbag, \( v_s = 0 \) (since it is hanging and at rest)

2. Apply the conservation of momentum:
Since the bullet sticks to the sandbag, we can use the conservation of momentum before and after the collision:
\( m v_p + M v_s = (m + M) v_f \)
where \( v_f \) is the final velocity of the bullet and sandbag together.

Plugging in the values:
\( 0.050 \, v_p + 5 \cdot 0 = (0.050 + 5) v_f \)
Simplifying:
\( 0.050 \, v_p = 5.050 \, v_f \)
Thus, we can solve for \( v_f \):
\( v_f = \frac{0.050 \, v_p}{5.050} \)

3. Calculate the initial and final kinetic energies:
- Initial kinetic energy of the bullet:
\( KE_{\text{initial}} = \frac{1}{2} m v_p^2 = \frac{1}{2} (0.050) v_p^2 = 0.025 v_p^2 \)
- Final kinetic energy of the bullet and sandbag together:
\( KE_{\text{final}} = \frac{1}{2} (m + M) v_f^2 = \frac{1}{2} (5.050) v_f^2 \)
Substituting \( v_f \):
\( KE_{\text{final}} = \frac{1}{2} (5.050) \left(\frac{0.050 \, v_p}{5.050}\right)^2 \)
Simplifying:
\( KE_{\text{final}} = \frac{1}{2} (5.050) \left(\frac{0.0025 \, v_p^2}{25.5025}\right) = \frac{0.050 \, v_p^2}{2 \cdot 5.050} \)

4. Calculate the ratio of final to initial kinetic energy:
\( \text{Ratio} = \frac{KE_{\text{final}}}{KE_{\text{initial}}} = \frac{\frac{0.050 \, v_p^2}{2 \cdot 5.050}}{0.025 v_p^2} \)
Simplifying this ratio:
\( = \frac{0.050}{2 \cdot 5.050 \cdot 0.025} \)
\( = \frac{0.050}{0.2525} = \frac{50}{252.5} = \frac{100}{505} \approx 0.198 \)

5. Final result:
The ratio of final to initial kinetic energy is approximately \( 0.198 \).