A thin uniform spherical shell of mass $m = 2 k g$ and radius $R = 10 c m$ is placed on a rough horizontal surface with a coefficient of friction $μ = 0.4$ . A horizontal force 'F' is applied to the spherical shell at a height $R / 3$ ablove the centre as shown. The maximum magnitude of the applied force 'F' for which the spherical shell will be rolling without slipping is (taking $g = 10 m / a^{2}$ )

A

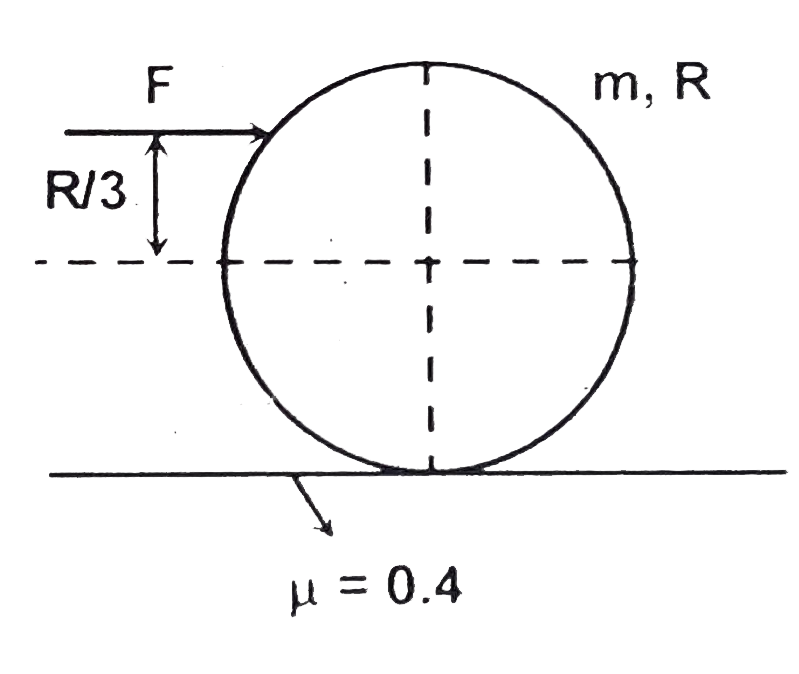
$20 N$

B

$30 N$

C

$40 N$

D

$60 N$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:C

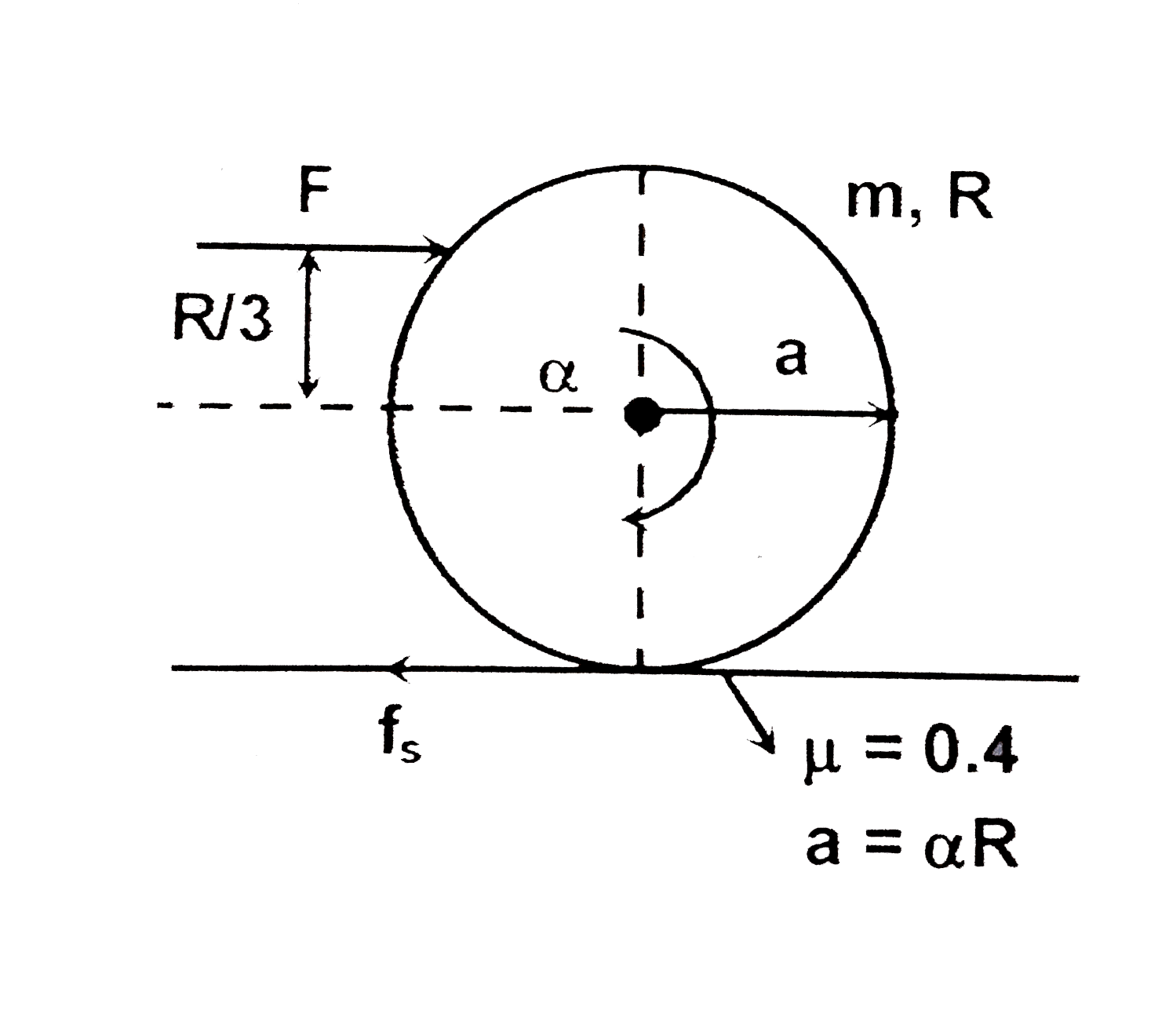
$F - f_{s} = m a$ …..(i)
$\frac{F R}{3} + f_{s} R = \frac{2}{3} m R^{2} α$
$\frac{F}{3} + f_{s} = \frac{2}{3} m a$ [since, $a = R α$ ] …..(i)
Solving (i) and (ii), we get
$\frac{4 F}{3} = \frac{5}{3} m a$
$a = \frac{4 F}{5 m}$
Now, from equation (i)
$f_{s} = F - m a = F - \frac{4 F}{5} = \frac{F}{5}$
Hence, $f_{s} \leq μ N$
$F / 5 \leq μ N$
$F \leq 5 μ m g$
$∴ F_{max} = 5 \times 0.4 \times 20 = 40 N$

|

Updated on:21/07/2023

Class 12 PHYSICS QUESTION BANK

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
A block of mass $m = 5 k g$ is resting on a rough horizontal surface for which the coefficient of friction is $0.2$ . When a force $F = 40 N$ is applied, the acceleration of the block will be $(g = 10 m / s^{2})$ .

A $5.73 m / {sec}^{2}$
B $8.0 m / {sec}^{2}$
C $3.17 m / {sec}^{2}$
D $10.0 m / {sec}^{2}$
Question 2 - Select One or More

A solid sphere of mass ma nd radis R is placed on a rough horizontal surface A horizontal force F is applied to sphere at a height h, $(0 \leq h \leq R)$ from centre. If sphere rolls slipping then,
AThe maximum possible acceleration of sphere is $\frac{F}{M}$
BThe maximum possible acceleration of sphere is $\frac{10 F}{7 M}$
CThe magnitude of friction force acting on the sphere is zero if $h = \frac{2 R}{5}$
DThe magnitude of friction force acting on the sphere is zero $h = \frac{3 R}{4}$ .
Question 3 - Select One or More
A block of mass m is placed on a rough horizontal surface. The coefficent of friction between them is $μ$ . An external horizontal force is applied to the block and its magnitude is gradually increased. The force exerted by the block on the surface is R.
AThe magnitude of R gradually increase.
B $R \leq m g \sqrt{μ^{2} + 1}$ .
CThe angle made by R with the vertical will gradually increase.
DThe angle made by R with the vertical $\leq {tan}^{- 1} μ$ .

FIITJEE-TEST PAPERS-PHYSICS

Home
Ask Doubt
Profile