In the arrangement shown in figure , the mass of the body A is 4 m and that of body B is m . The height h=0⋅2 m . If the body B is released , then till what maximum height the body B will go up ? (g = 10m/s2)
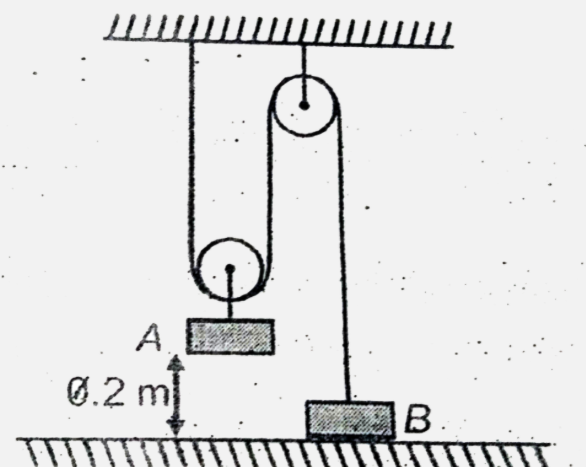
Let the body A go down with acceleration a1 and the body B go up with acceleration a2 ( just after release ) , then a2=2a2a1
4mg−2T=4ma1 …(i)
T−mg=ma2 ….(ii)
Multiplying equation (ii) by 2 and adding it to equation (i)
2mg=4ma1+2ma2
a1=2mg8m=g4
So, a2=2a1=g2
The time till the body A reaches the floor, both bodies will be moving with above mentioned accelerations. Just after the body A strikes the floor, all strings will get slack , ie tension in acceleration will be g downwards .
From the constraint reletions , the body B will be at height 0.4 m from floor, when A strikes the floor. As the body B starts from rest , so we can write
v2=u20+2as=0+2a2(0.4)
v2=2(g2)0.4=4
or ⇒v=2m/s
After this body B will retard and at highest point velocity of body B will again become zero
so , v2=u2+2as
0=4−2(10h)⇒h=0.2m
So height from ground is 0.4+0.2+0.6m

Topper's Solved these Questions
- LAWS OF MOTIONBOOK - AAKASH INSTITUTECHAPTER - LAWS OF MOTIONEXERCISE - Illustration
5 Videos
- LAWS OF MOTIONBOOK - AAKASH INSTITUTECHAPTER - LAWS OF MOTIONEXERCISE - TRY YOURSELF
67 Videos
- KINETIC THEORYBOOK - AAKASH INSTITUTECHAPTER - KINETIC THEORYEXERCISE - EXERCISE (ASSIGNMENT) SECTION - D Assertion - Reason Type Questions
10 Videos
- MAGNETISM AND MATTER BOOK - AAKASH INSTITUTECHAPTER - MAGNETISM AND MATTER EXERCISE - ASSIGNMENT (SECTION D)
26 Videos
Similar Questions
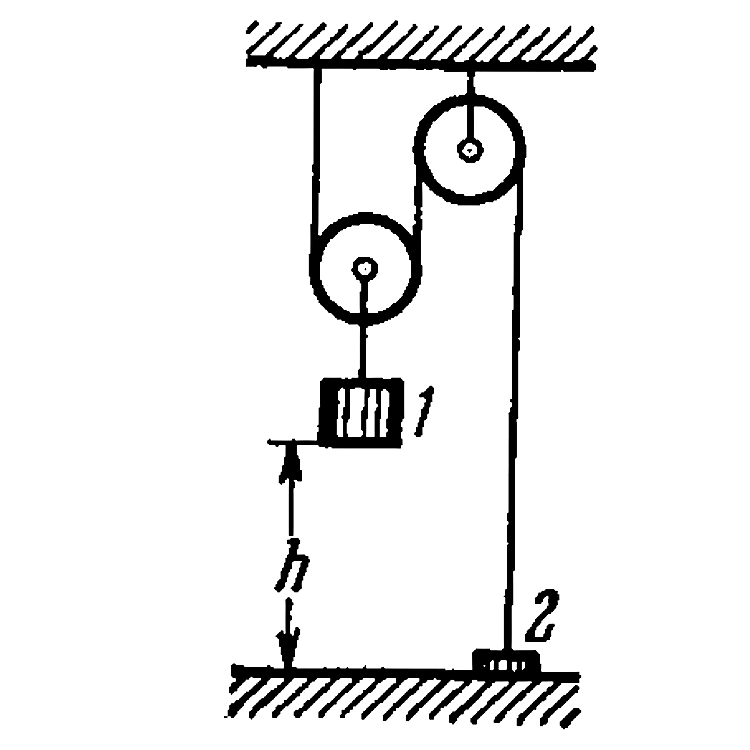
In the arrangement shown in figure the mass of body 1 is η=4.0 times as great as that of body 2. The height h=20cm. The masses of the pulleys and the threads, as well as the friction, are negligible. At a certain moment body 2 is released and the arrangement set in motion. What is the maximum height that body 2 will go up to?
View SolutionIn the arrangement show in figure, the track is frictionless until the body reaches the higher level. A frictional force stops the body in a distance d from A. The initial speed of the body v0 is 6 m/s the height h is 1 m and the coefficient of kinetic friction is 0.2.
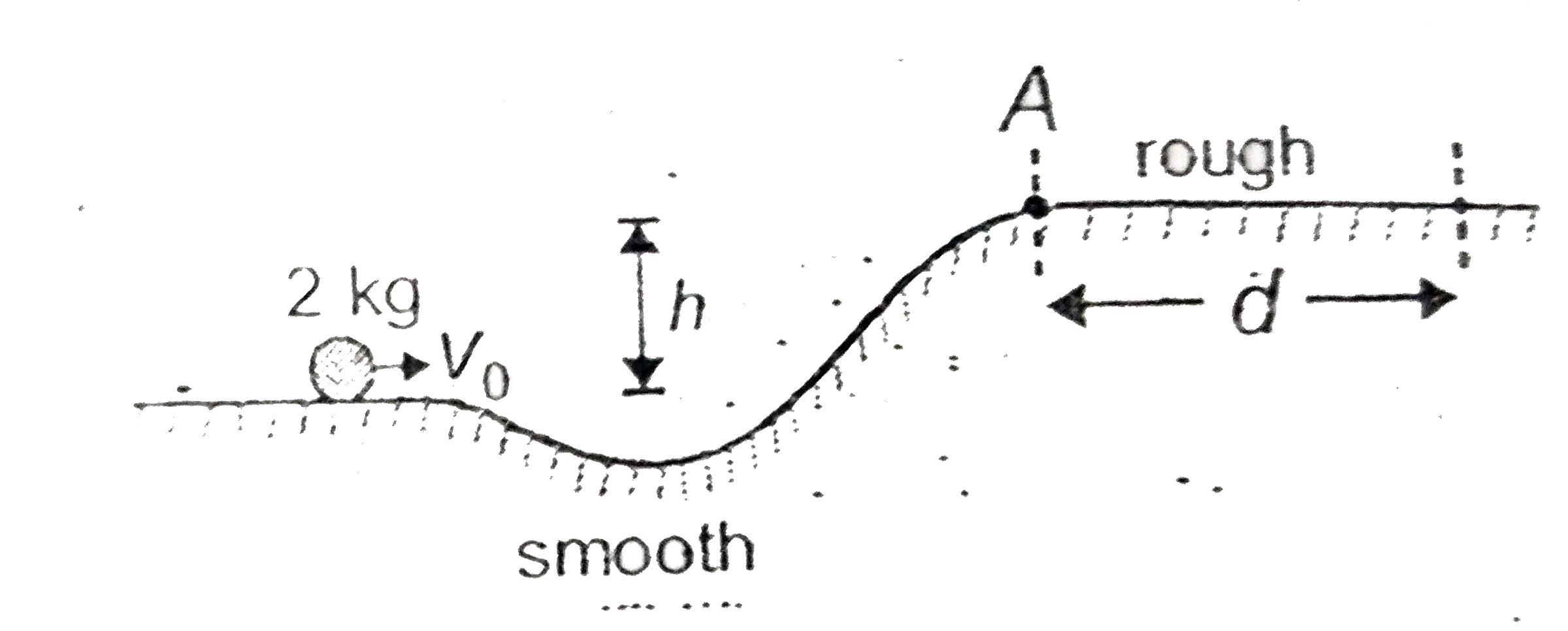
The speed of body at the higher level A is
View Solution
Knowledge Check
If a body is thrown up with the velocity of 15 m/s then maximum height attained by the body is (g=10m/s)
A11.25mB16.2mC24.5mD7.62mThe kinetic energy of a body of mass 5 kg is 100 J. When it is thrown up then the height to which it goes up, is(g=10m/s2)
A5mB1mC2mD10mA body is dropped from a height of 40m. After it crosses half distance, the acceleration due to gravity ceases to act. The body will hit ground with velocity (Takeg=10m/s2) :
A20m/sB10m/sC2m/sD15m/s
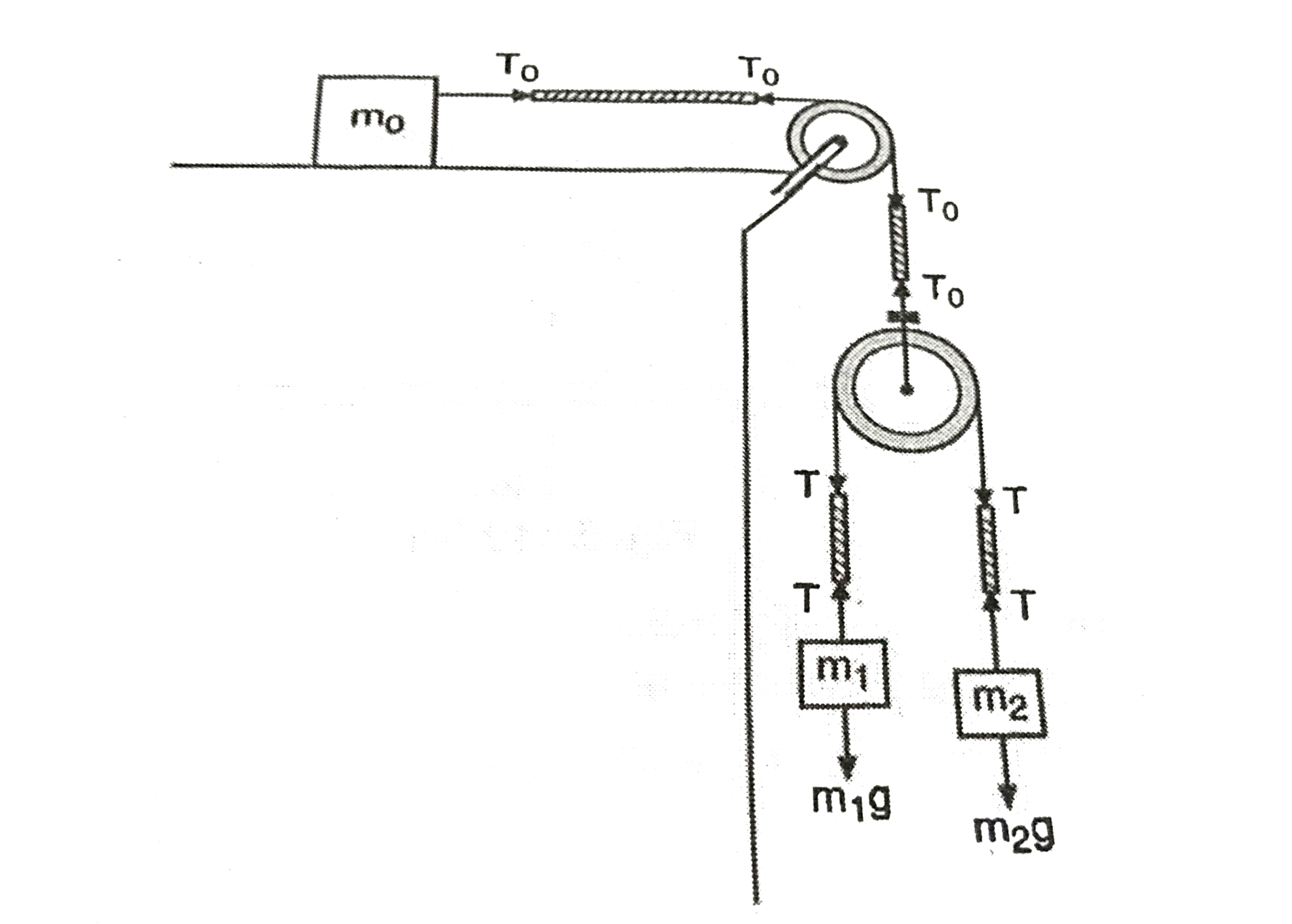
In the arrangement shown in fig the bodies have masses m0,m1andm2 the friction is absent the masses of pulleys and the threads are negligible find the acceleration of the body m1
View SolutionA body is released from the top of a tower of height 50 m. Find the velocity with which the body hits the ground. (g=9.8m/s2)
View SolutionWhat is the gravitational potential energy of a body of mass m at a height h ?
View SolutionWhat is the work done to fits to lift a body of mass 5 kg to a height of 50 m from the ground (in J)? (g = 10 m s−2)
View SolutionA body is thrown vertically upward from a point A 125 m above the ground. It goes up to a maximum height of 250 m above the ground and passes through A on its downward journey. The velocity of the body when it is at a height of 70 m above the ground is (g=10m/s2)
View Solution
