Two equal masses each in are hung from a balance whose scale pans differ in vertical height by h. The error in weighing in terms of density of the earth ρ is
_S01_008_S01.png)
gh=[1−2hRe],W2,W1=mg2−mg1=2mg[h1Re−h2Re]=2mGMR2e×hRe[∵g=GMR2e&h1−h2=h]
Error in weighing =W2−W1=2mG43πR3eρhR3e=8π3Gmρh
Due to depth
Assuming density of earth remains same throughout
At earth surface, g=43πGReρ ...(i) at depth d inside the earth
For point P only mass of the inner sphere is effective gd=GM'r2
gd=Gr2×Mr2R2e=GMR2e×rRe=GMR2e×Re−dRe
Mass of sphere of radius r=M'
M'=43πr3ρ=43πr3×M4/3πR3=M'=MR3r3
gd=g[1−dRe] valid for any depth.
Topper's Solved these Questions
- GRAVITATIONBOOK - ALLENCHAPTER - GRAVITATIONEXERCISE - Exercise 1 (Check your Grasp)
28 Videos
- GRAVITATIONBOOK - ALLENCHAPTER - GRAVITATIONEXERCISE - Exercise 2 (Brain Teasers)
27 Videos
- GEOMETRICAL OPTICSBOOK - ALLENCHAPTER - GEOMETRICAL OPTICSEXERCISE - subjective
14 Videos
- KINEMATICS-2DBOOK - ALLENCHAPTER - KINEMATICS-2DEXERCISE - Exercise (O-2)
48 Videos
Similar Questions
Two objects of equal mass rest on the opposite pans of an arm balance. Does the scale remain balance when it is accelerated up or down in a life ?
View SolutionOn the opposite sides of vertical vessel filled with water, two identical holes are opened. If area of cross-section for each is a and difference of height of these two is h and p is density of water, then force on the vessel is
View Solution
Knowledge Check
Two equal masses each m are hung from a balance whose scale pans differ in vertical height by h. The error in weighing is
AπGρmhB13GρmhC83πGρmhD43πGρmhTwo equal masses each in are hung from a balance whose scale pans differ in vertical height by h. The error in weighing in terms of density of the earth ρ is
AπGρmhB13πGρmhC83πGρmhD43πGρmhTwo identical blocks of mass m are suspended from a beam balance whose scale pans differ in vertical height by h(<<R), if R and ρ are the radius and density of the earth, then the error in weighing is
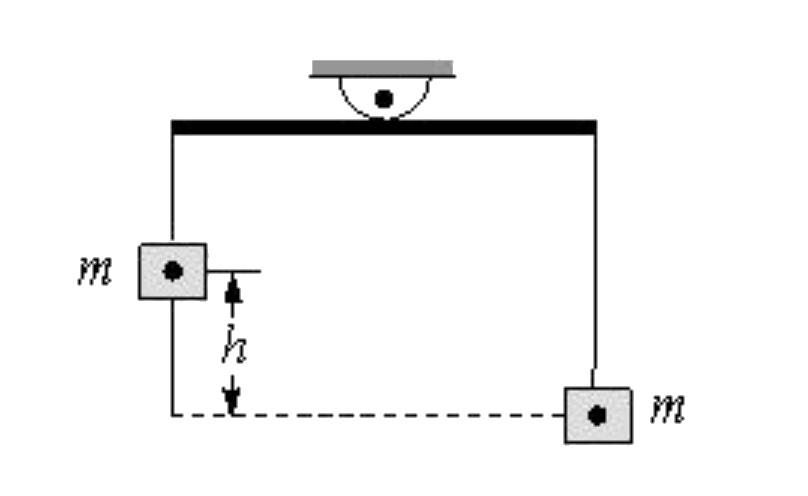 A23πρR3GmB83πρGmhC83πρR3GmD43πρGm2h
A23πρR3GmB83πρGmhC83πρR3GmD43πρGm2h
Two identical blocks of mass m are suspended from a beam balance whose scale pans differ in vertical height by h(h<<R), if R and ρ are the radius and density of the earth, then the error in weighing is

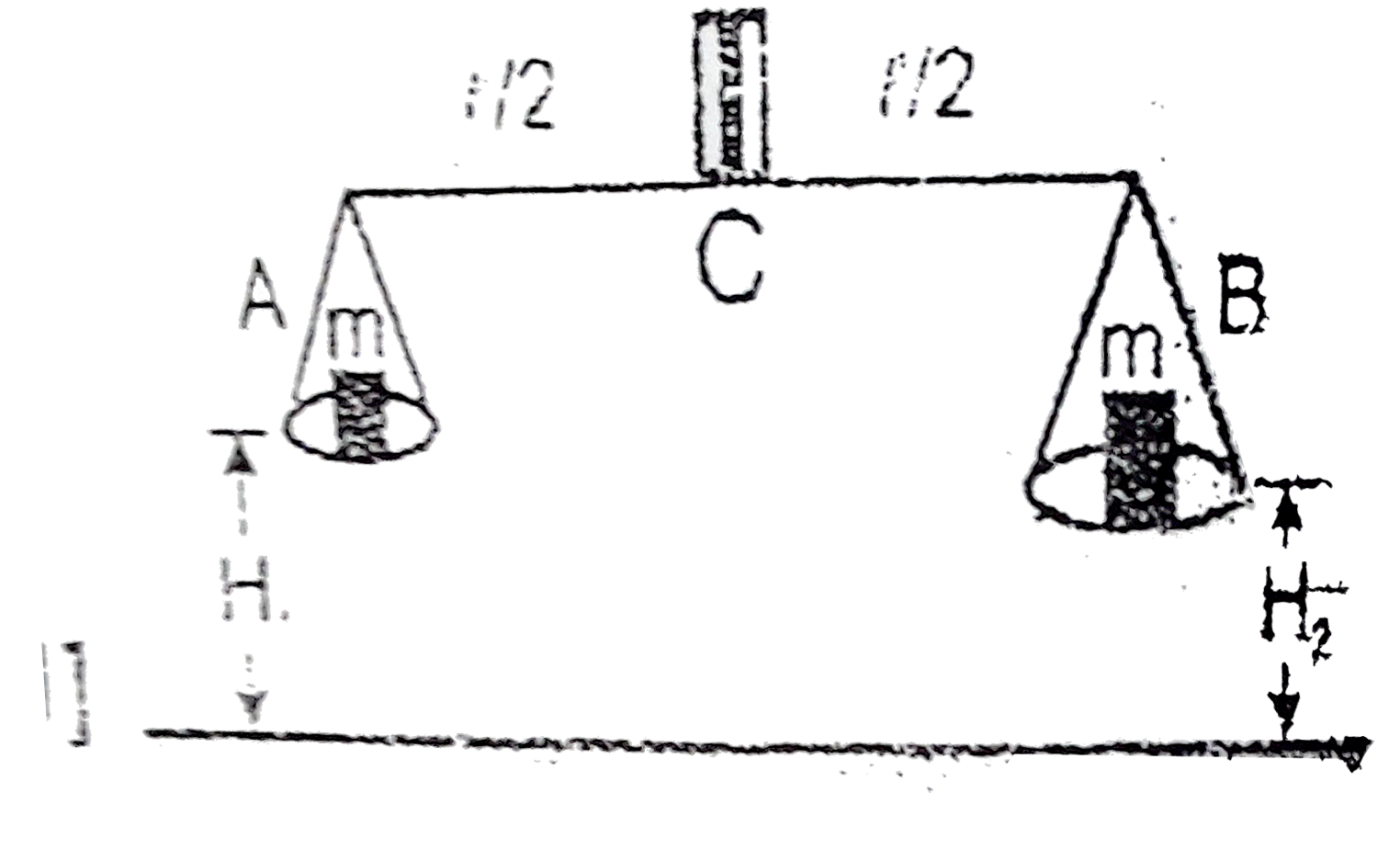
View SolutionTwo blocks of masses m each are hung from a balances as shown in the figure. The scale pan A is at height H1 whereas scale pan B is at height H2 Net torque of weights acting on the system about point C will be (length of the rod is l and H1 & H2 are lt ltR ) (H1>H2)
 .
.
View SolutionTwo vertical plates submerged partially in a wetting liquid form a wedge with a very small angle δφ. The edge of this wedge is vertical. The density of the liquid is ρ, its surface tension is α, the contact angle is θ. Find the height h, to which the liquid rises, as a function of the distance x from the edge.
View SolutionThere are two bodies A and B of same mass. A is placed near equator of earth and B is placed at a height h above the pole of earth. if both the bodies weighs equally. Find h in terms of radius R of earth, angular speed ω of earth and g acceleration due to gravity close to earth.
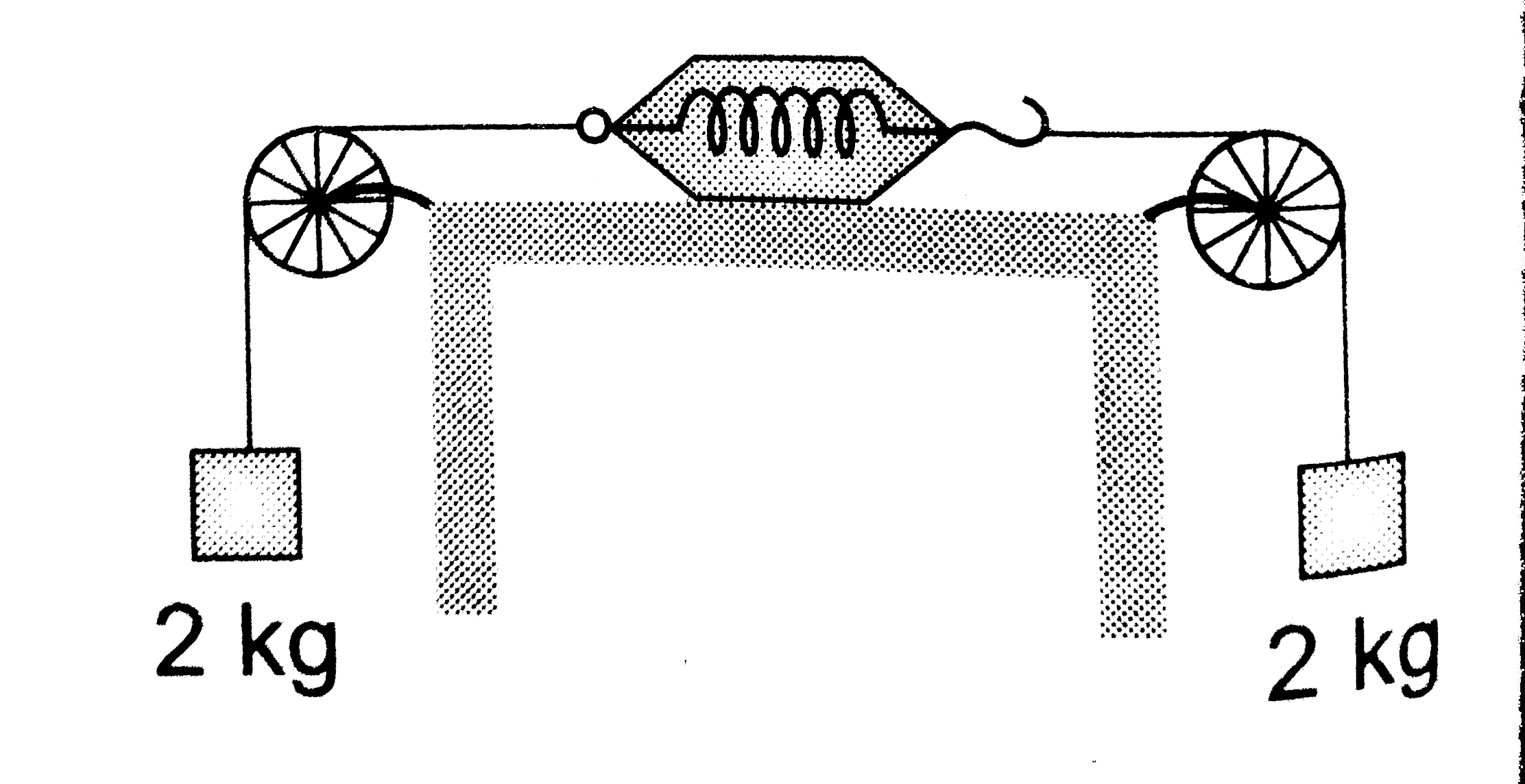
View SolutionAs shown in the figure, two equal masses each of 2kg are suspended from a spring balance. The reading of the spring balance will be.
View Solution
