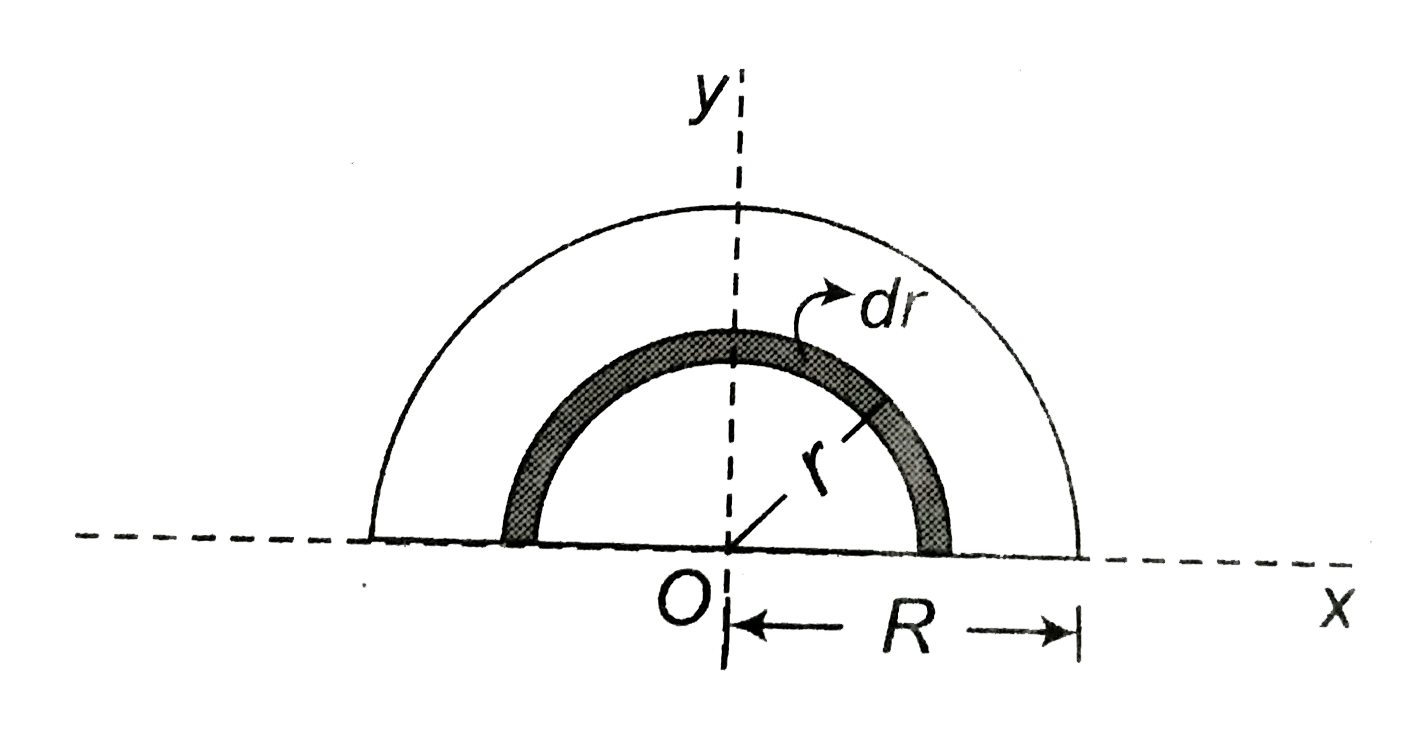
Locate c.m. of thin , uniform semicircular plate.
Video Solution
Text Solution
Mass/area=MπR2/2=2MπR2
Here , the elements is semicircular ring of radius and thickness for
dA=πrdr
dm=2MπR2×πrdr=2MR2rdr
The coordinates of the c.m. of element , y=2rπ
yc.m.=∫ydm∫dm=∫R02rπ2MrdrR2M
=4πR2∫R0r2dr=4πR2r3(3R0
=4πR2(R33)=4R3π
|
Topper's Solved these Questions
Similar Questions
Knowledge Check
A folded plate OABCDEFO made of materials such that part OABO (say part(i), BCDOB hs mass 4m,m and m respectively. Part (i) is a uniform semicircular plate of radius R/2 and in on the xy plane. Part (ii) and (iii) each is a uniform quarter circular plate of radius R on xy and xz plane respectively. The whole system is free to rotate about y-axis A particle P of mass m moving with velocity v0 hits to a point located at the circumcentre of the part (iii) and sticks to it. The point is at a distance R/2 from x -axis as shown in the figure. Angular velocity of the combined system just after the collision is
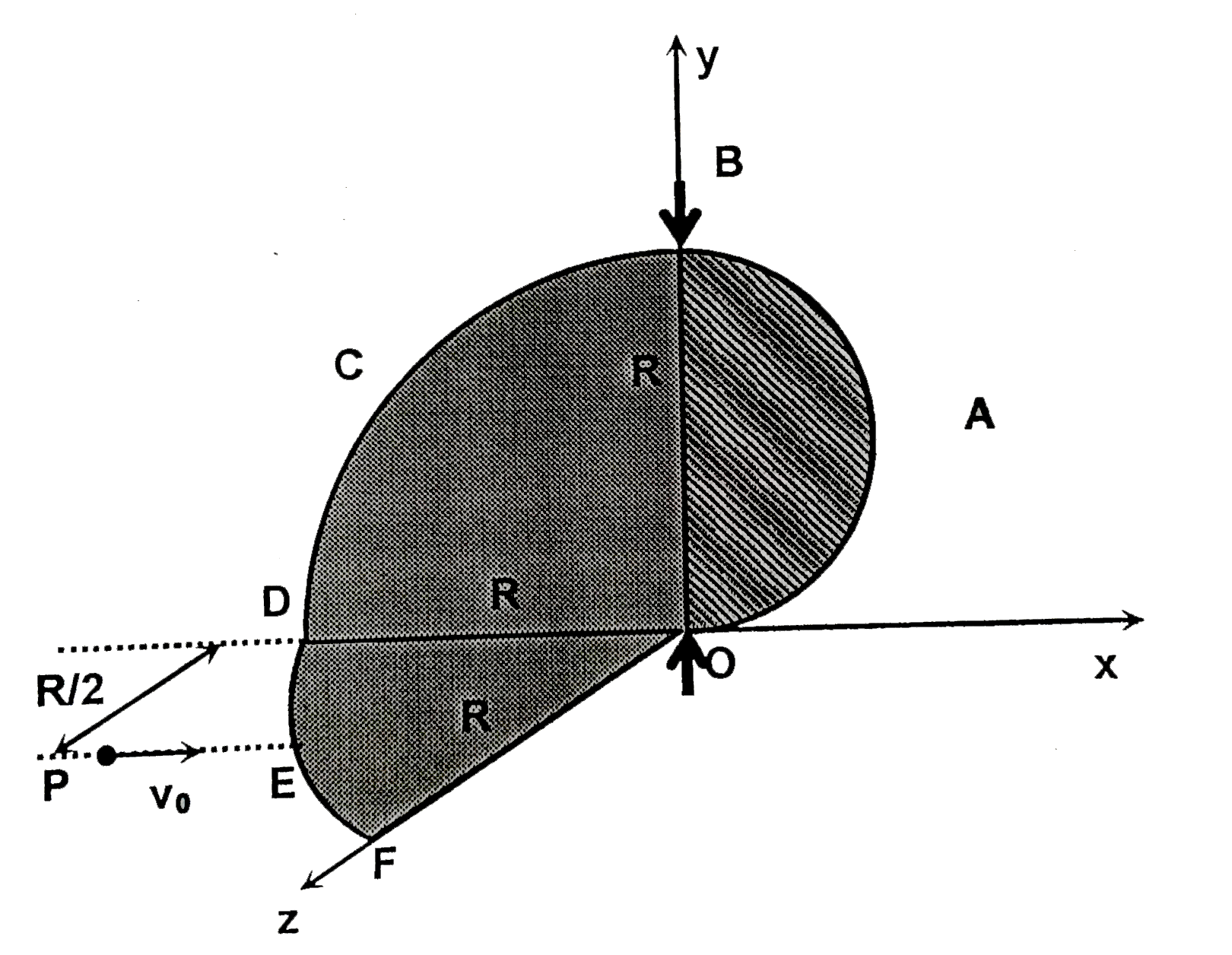 Av04RB2v05RC3v05RDv02R
Av04RB2v05RC3v05RDv02RThe moment of inertia of a uniform semicircular disc of mass M and radius r about a line perpendicular to the plane of the disc through the center is
A12Mr2BMt2C25Mr2D14Mr2The moment of inertia of a uniform semicircular disc of mass disc through the centre is
A25Mr2B14Mr2C12Mr2DMr2
Find coordinates of mass center of a uniform semicircular plate of radius r placed symmetric to the y-axis of a Cartesian coordinate system, with centre at origin.
View SolutionMass Center of a sector of a uniform circular plate
Find location of mass center of a sector of a thin uniform plate.
View SolutionFind the coordination of the centre of mass of a uniform semicircular wire of radius R and mass M.
View SolutionWhat is the moment of inertia of the thin uniform rectangular plate of mass m about the diagonal axis ×' ?

View SolutionFind the centre of mass of a uniform semicircular ring of radius R and mass M.
View Solution
