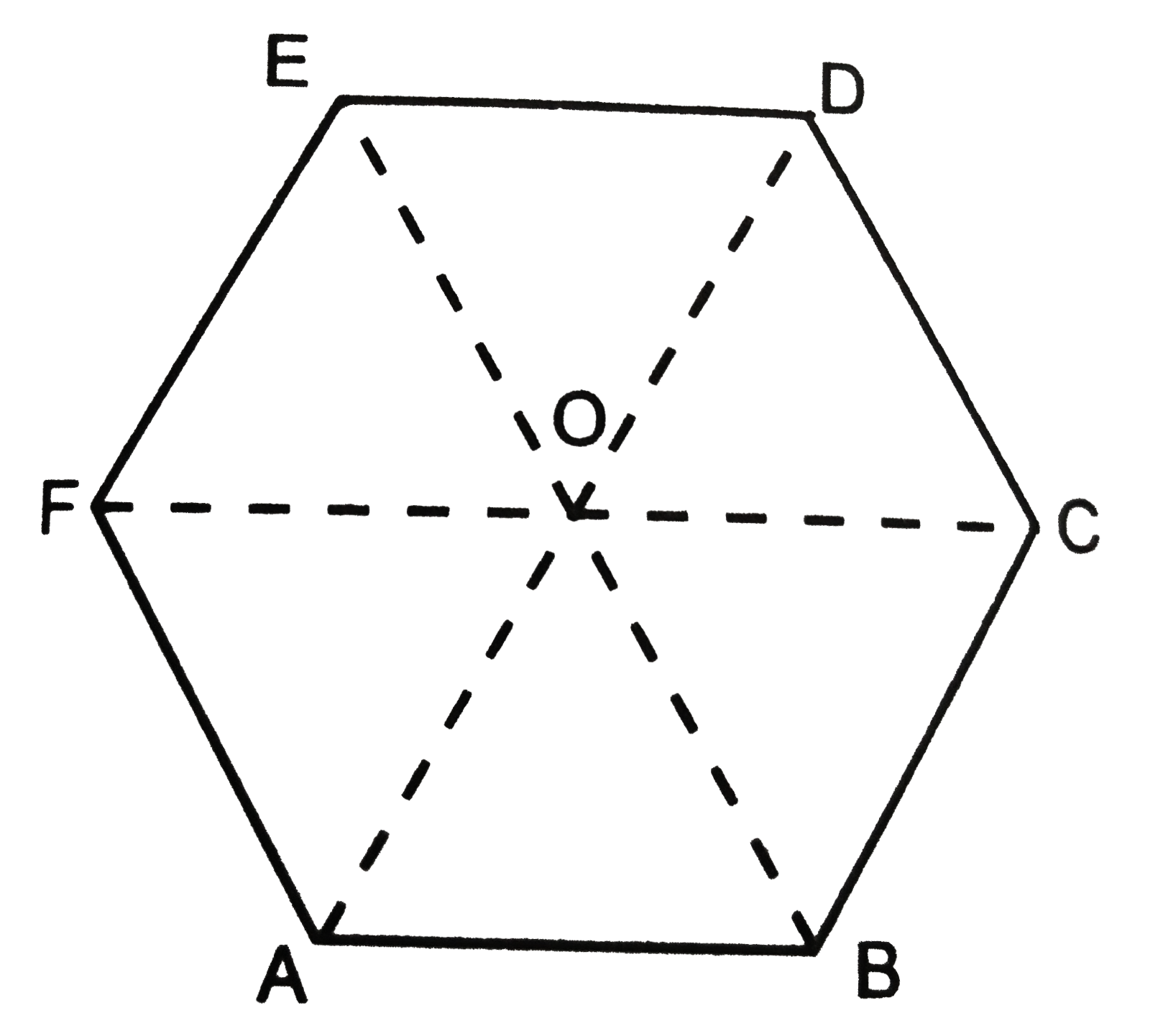
ABCDEF is a regular hexagon with point O as centre. The value of $- - \to A B + - - \to A C + - - \to A D + - - \to A E + - - \to A F$ is

Video Solution

Text Solution

Verified by Experts

From the diagram $- - \to A B = - --- \to - D E, - - \to B C = - -- \to - E F$
$- - \to A B + - - \to A C + - - \to A D + - - \to A E + - - \to A F$ .
$= - - \to A B + (- - \to A B + - - \to B C) + - - \to A D + (- - \to A D + - - \to D E) + (- - \to A D + - - \to D E + - - \to E F)$
$= - -- \to 3 A D = 3 (- -- \to 2 A O) = 6 - -- \to (A O)$

|

Updated on:21/07/2023

Class 11 PHYSICS PROJECTILE MOTION

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
Consider the ragular hexagon ABCDEF with centre at O (origin).
Five forces $- - \to A B, - - \to A C, - - \to A D, - - \to A E, - - \to A F$ act at the vertex A of a regular hexagon ABCDEF. Then their resultant is
A $3 - - \to A O$
B $2 - - \to A O$
C $4 - - \to A O$
D $6 - - \to A O$
Question 2 - Select One
Let O be the centre of a regular pentagon ABCDE and $- - \to O A = \to a$ , then $- - \to A B + - -- \to 2 B C + - -- \to 3 C D + - -- \to 4 D E + - -- \to 5 E A$ is equals:
A0
B $4 \to a$
C $5 \to a$
D $6 \to a$
Question 3 - Select One
Let O be the centre of a regular pentagon ABCDE and $- - \to O A = \to a$ , then $- - \to A B + - -- \to 2 B C + - -- \to 3 C D + - -- \to 4 D E + - -- \to 5 E A$ is equals:
A $6 \to a$
B $5 \to a$
C $4 \to a$
D $\to 0$

NARAYNA-MOTION IN A PLANE-Level-II(H.W)

Home
Ask Doubt
Profile