A ray of light undergoes deviation of 30∘ when incident on an equilateral prism of refractive index √2. what is the angle subtended by the ray inside the prism with the base of the prism?
δ=30∘, A=60∘, μ=√2
Let us first calculate δm
μ=sin(A+δm2)sin(A/2)
√2=sin(60∘+δm2)sin30∘=1/2
sin(60∘+δm2)=1√2=sin45∘
60∘+δm2=45∘
δm=30∘=δ
Since δ=δm, i.e. ray inside the prism is parallel to the base of the prism.
Topper's Solved these Questions
- REFRACTION OF LIGHT BY PLANE SURFACESBOOK - CP SINGHCHAPTER - REFRACTION OF LIGHT BY PLANE SURFACESEXERCISE - Exercises
110 Videos
- REFRACTION AT SPHERICAL SURFACESBOOK - CP SINGHCHAPTER - REFRACTION AT SPHERICAL SURFACESEXERCISE - EXERCISES
105 Videos
- SEMICONDUCTORSBOOK - CP SINGHCHAPTER - SEMICONDUCTORSEXERCISE - Exercises
140 Videos
Similar Questions
A ray of light undergoes a deviation of 30∘ when incident on an equilateral prism of refractive index √2.
What is the angle subtended by the ray inside the prism with the base of the prism?
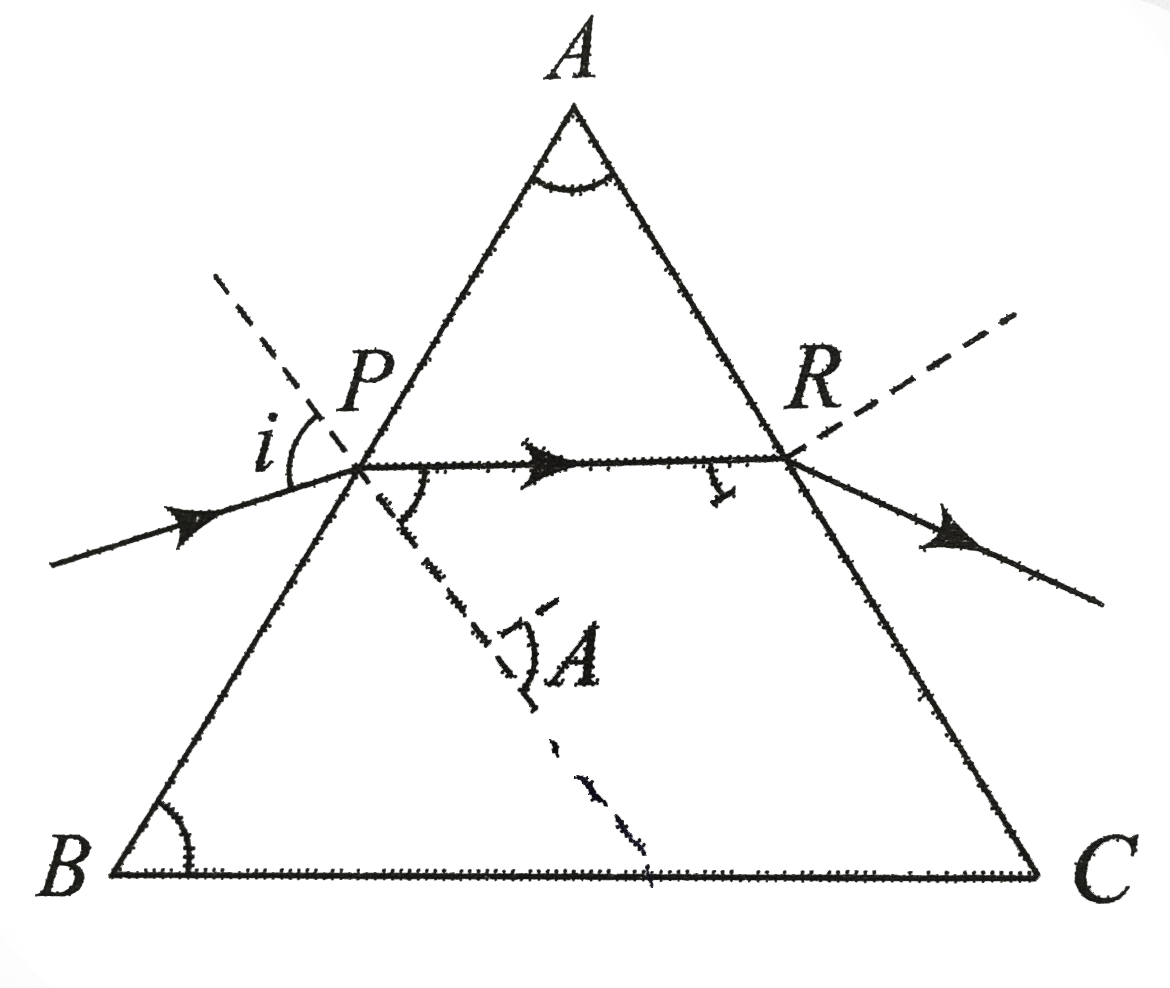
View SolutionA ray of light undergoes deviation of 30∘ when incident on a n equilateral prism of refractive index √2. The and made by the ray inside the prism with the base of the prism is ________________.
View Solution
Knowledge Check
A ray of light undergoes deviation of 30∘ when incident on an equilateral prism of refractive index √2. The angle made by the ray inside the prism with the base of the prism is
A15∘B0∘C45∘D30∘A ray of light suffers minimum deviation when incident on an equilateral prism of refractive index √2 . What is the angle of incidence ?
A30∘B45∘C60∘D90∘A ray of light suffers a minimum deviation when incident on an equilateral prism of refractive index √2 The angle of incidence is
A30∘B45∘C60∘D50∘
A ray of light undergoes deviation of 30degree when incident on an equilateral prism of refractive index √2. The angle made by the ray inside the prism with the base of the prism is …..
View SolutionA ray of light suffers a minimum deviation when incident on equilateral prism of refractive index √2 The angle of deviation is
View SolutionWhat is the angle of incidence for an equilateral prism of refractive index √3 so that the ray si parallel to the base inside the prism?
View SolutionFor an angle of incidence θ on an equilateral prism of refractive index √3, the ray refracted is parallel to the base inside the prism. The value of θis
View SolutionWhen a ray of light is incident normally on one refracting surface of an equilateral prism of refractive index 1.5, then
View Solution
