A vessel having perfectly reflecting plane botton is filled with water (μ=4/3) to depth d. A point source of light is placed at a height h above the surface of water. Find the distance of final image from water surface.

Shift =d(1−1/μ)
Mirror will be shifted towards object by d(1−1/μ)
Distance of object from virtual mirror
M′M′0=(h+d)−d(1−1/μ)=h+dμ
Distance of image I from virtual mirror =h+d/μ
Distance of I from real mirror
MM0=h+dμ−d(1−1/μ)
=h−d+2dμ
Distance from final image from surface of water
=(h−d+2dμ)+d
=h+2dμ=h+2d4/3
=h+3d2
Topper's Solved these Questions
- REFRACTION OF LIGHT BY PLANE SURFACESBOOK - CP SINGHCHAPTER - REFRACTION OF LIGHT BY PLANE SURFACESEXERCISE - Exercises
110 Videos
- REFRACTION AT SPHERICAL SURFACESBOOK - CP SINGHCHAPTER - REFRACTION AT SPHERICAL SURFACESEXERCISE - EXERCISES
105 Videos
- SEMICONDUCTORSBOOK - CP SINGHCHAPTER - SEMICONDUCTORSEXERCISE - Exercises
140 Videos
Similar Questions
A point source of light is placed on the bed of a pond 80 cm deep. Find the diameter of the liuminatded circle on the surface of water.
View SolutionA point source is placed at a depth h below the surface of water (refractive index =μ). The medium above the surface of water is air from water.
View Solution
Knowledge Check
A bird is flying 3m above the surface of water. To a fish underwater, the height of the bird from the water surface appears to be
A2.25mB3.33mC4mD4/3mA cylinderical vessel is filled with water up to height H. A hole is bored in the wall at a depth h from the free surface of water. For maximum range h is equal to
AH/4BH/2C3H/4DHA point source of light is placed at a depth h=0.5 m below the surface of a liquid (μ=54) , Then , the fraction of light energy that escape directly from the liquid surface is
A0.1B0.2C0.3D0.4
A point source of light is placed at distance h below the surface of a large and deep lake. What fraction of light will escape through the surface of water?
View SolutionA vessel, whose bottom is flat and perfectly reflecting, is filled with water (∈dex=4/3) upto a height = 40 cm . A point object in air above is moving towards the water surface with a constant speed =4m/s What is the relative speed of its final image (∈m/s), as seen by the object itself, at a moment when the object is 30 cm above the water surface?
View SolutionA point source of light is 80.0cm below the surface of a body of water. Find the diameter of the circle at the surface through which light emerges from the water.
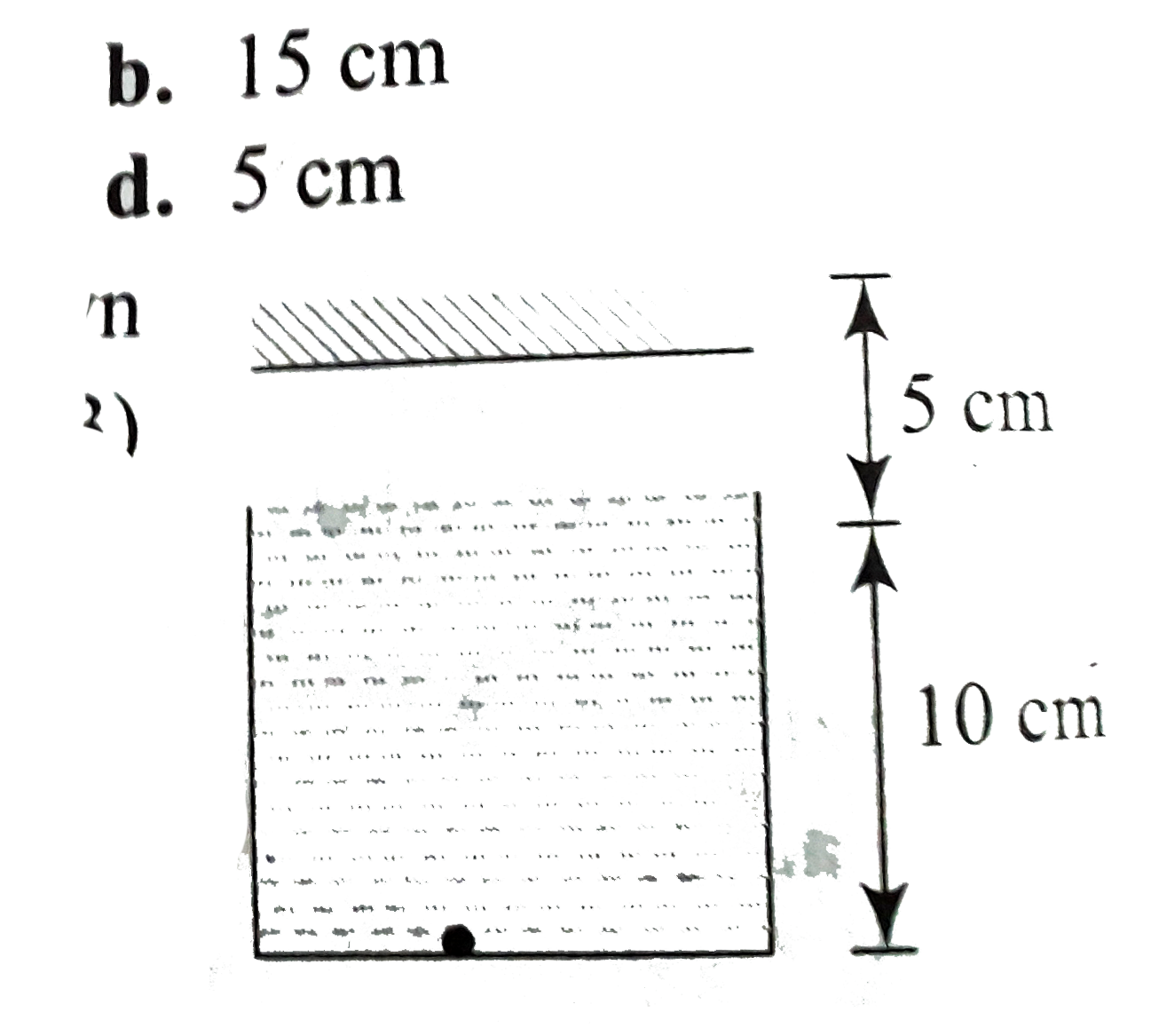
View SolutionConsider the situation shown in figure. Water (μ=4/3) is filled in a beaker up to a height of 10cm.
A plane mirror is fixed at a height of 5 cm from the surface of water. Distance of image from the mirror after reflection from it of an object O at the bottom of the beaker is
View SolutionA tank is filled with water up to a height H. Water is allowed to come out of a hole P in one of the walls at a depth D below the surface of water. Express the horizontal distance x in terms of H and D
 .
.
View Solution
