The central fringe of the interference pattern produced by the light o

The central fringe of the interference pattern produced by light of wavelength 6000A is found to shift to the position of 4th bright fringe after a glass plate of refraction index 1.5 is introduced in front of one slit in young's experiment. The thickness of the glass plate will be
A $4.8 μ m$
B $8.23 μ m$
C $14.98 μ m$
D $3.78 μ m$
To make the central fringe at the center $O$ , mica sheet of refractive index $1.5$ is introduced Choose the corect statement(S).

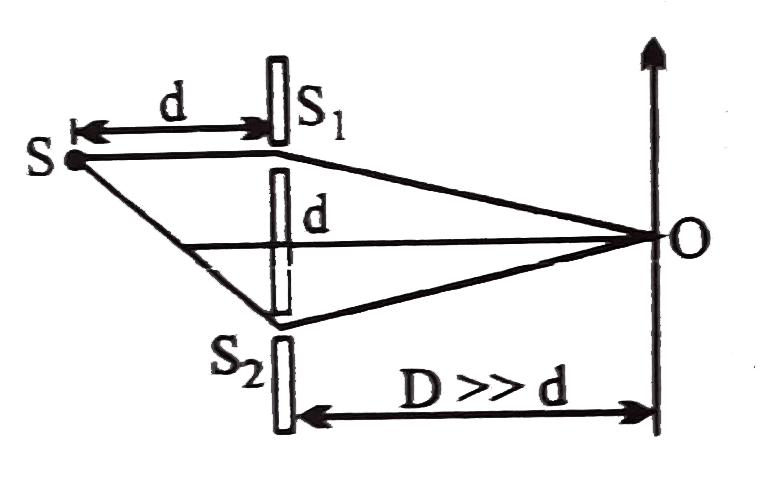AThe thickness of sheet is $2 (\sqrt{2} - 1) d$ infornt of $S_{1}$
BThe thickness of sheet is $(\sqrt{2} - 1) d$ infornt of $S_{2}$
CThe thickness of sheet is $2 \sqrt{2} d$ infornt of $S_{1}$
DThe thickness of sheet is $(2 \sqrt{2} - 1) d$ infornt of $S_{1}$
The central fringe shifts to the position of fifth bright fringe, if a thin film of refractive index 1.5 is introduced in the path of light of wavelength $5000 Å$ . The thickness of the glass plate is
A $1 μ m$
B $5 μ m$
C $3 μ m$
D $4 μ m$

The central fringe of the interference pattern produced by the light of wavelength 6000 Å is found to shift to the position of 4th dark fringe after a glass sheet of refractive index 1.5 is introduced. The thickness of glass sheet would be