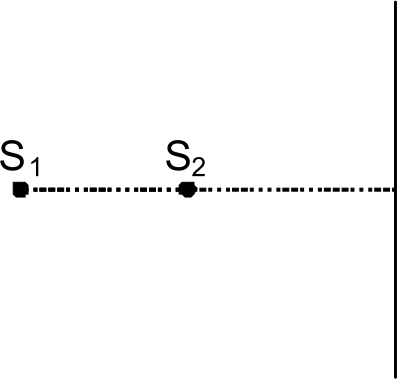
Two source S1 and S2 of intensity I1 and I2 are placed in front of a screen [Figure a]. The pattern of intensity distribution see in the central portion is given by Figure b. In this case which of the following statement are true.
![Two source S(1) and S(2) of intensity I(1) and I(2) are placed in front of a screen [Figure a]. The pattern of intensity distribution see in the central portion is given by Figure b. In this case which of the following statement are true.](https://d10lpgp6xz60nq.cloudfront.net/physics_images/NAR_PHY_V02_XII_C01_E01_237_Q01.png)

S1 and S2 have the same intensities.
S1 and S2 have a constant phase difference
S1 and S2 have thesamephase.
S1 and S2 have the same wavelength.
The correct Answer is:A, B, D
The resultant intensity of two identical coherent lights when superposed is
I=4I0cos2(θ2)
for two sources to be coherent phase difference must be constant and should have same wavelength
Topper's Solved these Questions
- BOOK - NARAYNACHAPTER - WAVE OPTICSEXERCISE - LEVEL - V37videos
WAVE OPTICS
- BOOK - NARAYNACHAPTER - WAVE OPTICSEXERCISE - LEVEL - VI40videos
WAVE OPTICS
- BOOK - NARAYNACHAPTER - WAVE OPTICSEXERCISE - LEVEL - III30videos
WAVE OPTICS
- BOOK - NARAYNACHAPTER - SEMICONDUCTOR ELECTRONICSEXERCISE - ADDITIONAL EXERCISE (ASSERTION AND REASON TYPE QUESTIONS :)19videos
SEMICONDUCTOR ELECTRONICS
Similar Questions
If I1 and I2 are the intensities of two waves then ratio of maximum and minimum intensities is
View SolutionTwo identical sources of sound S1andS2 produce intensity I0 at a point P equidistant from each source .
(i) Determine the intensity of each at the point P.
(ii) If the power of S1 is reduced to 64% and phase difference between the two sources is varied continuously , then determine the maximum and minimum intensities at the point P.
(iii) If the power of S1 is reduced by 64% , then determine the maximum and minimum intensities at the point P.View Solution
Knowledge Check
- Question 1 - Select One
Two coherent sources of intensities I1 and I2 produce an interference pattern. The maximum intensity in the interference pattern will be
A(a) I1+I2B(b) I21+I22C(c) (I1+I2)2D(d) (√I1+√I2)2 - Question 2 - Select One
A sphere of radius R is exposed to a parallel beam of radiation of intensity I as shown in figure. Choose the correct option (s) of the following.
AIf the surface of the sphere is completely reflecting, radiation force in the sphere is 2IπR2cBIf surface of the sphere is completely absorbing, radiation force on the sphere is IπR2cCIf surface of the sphere is completely reflecting, radiation force on the sphere is IπR22cDIf surface of the sphere is partially reflecting with reflection coefficent 0.3 and absorbing coefficent 0.7, the radiation force in the sphere is 1.71πR2c - Question 3 - Select One
A point source of light S is placed in front of a perfectly reflecting mirror as shown in Figure. Σ is a screen. The intensity at the center of screen is found to be I.
If the mirror is removed, then the intensity at the center of screen would be
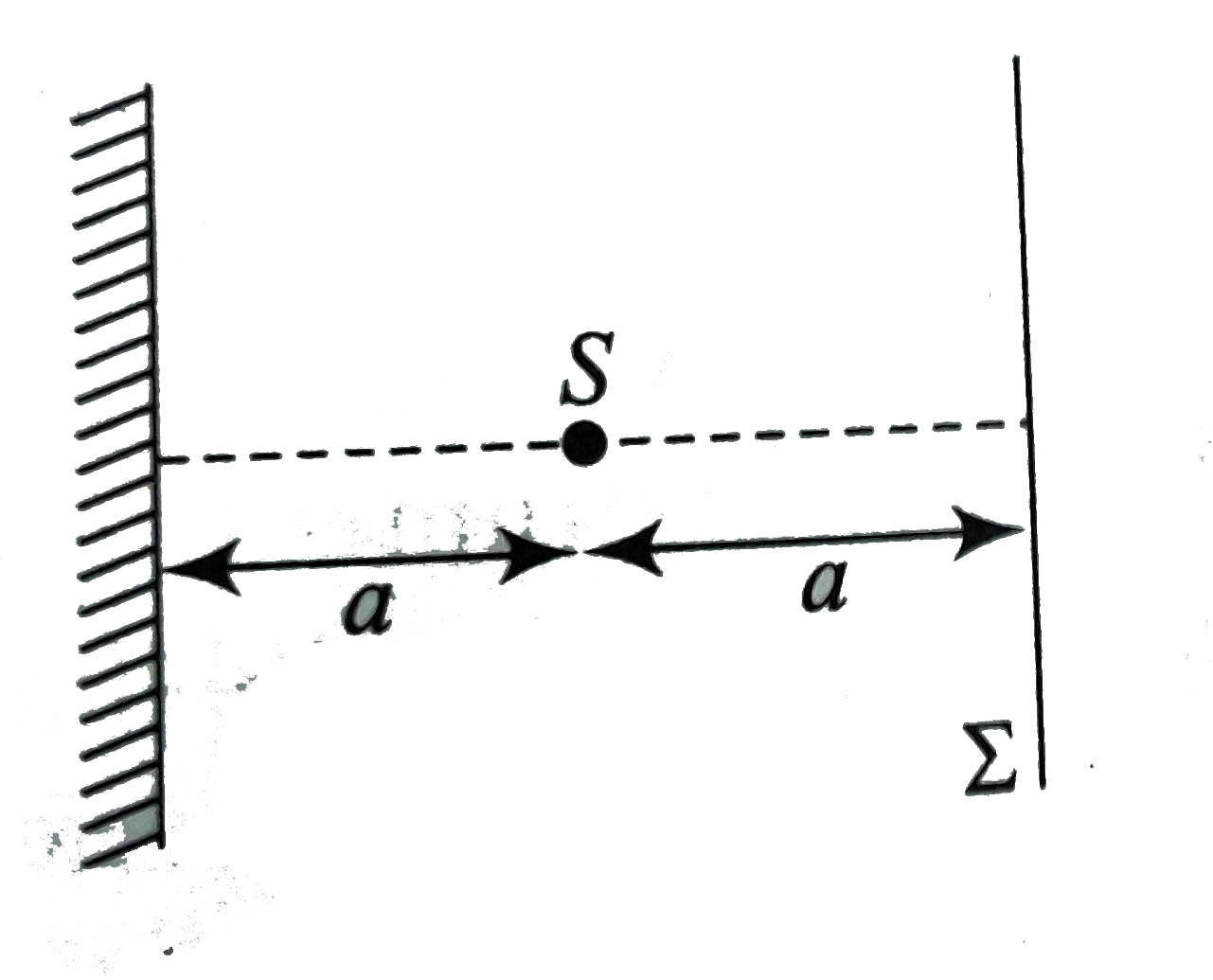 AIB10I/9C9I/10D2I
AIB10I/9C9I/10D2I
Three coherent sourcesS1,S2 andS3 can throw light on a screen. With S1 switched on intensity at a point P on the screen was observed to be I. With onlyS2 on, intensity at P was 2I and when all three are switched on the intensity at P becomes zero. Intensity at P is I when S1 andS2 are kept on. Find the phase difference between the waves reaching at P from sources S1andS3.
View SolutionTwo coherent monochromatic point sources S1 and S2 are placed in front of an infinite screen as shown in figure. Wavelength of the light emitted by both the sources is zero. Initial phase difference between the sources is zero.
Initially S1S2=2.5λ and the number of bright circular rings on the screen in n1. if the distance S1S2 is increased and made 5.7λ, the number of bright circular rings becomes n2. the difference n2−n1 isView SolutionTwo sources of intensity 2I and 8I are used in an interference experiment. The intensity at a point where the waves from two sources superimpose with a phase difference of (a) zero (b) π/2and(c)π is
View SolutionA point source of lightS is placed in front of two large mirros as shown .Which of the following observes will see only one image of S?
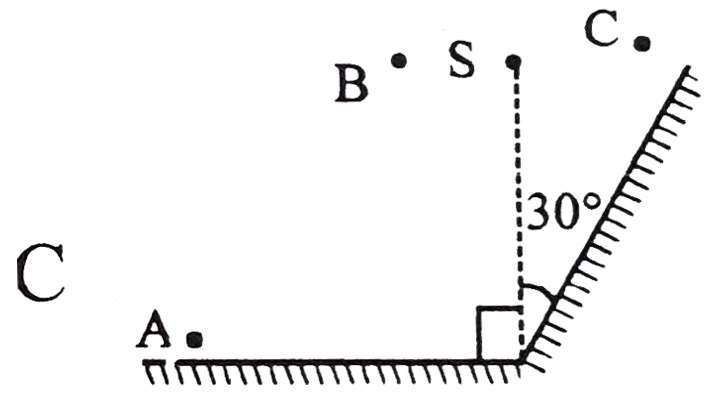 View Solution
View SolutionS1andS2 are two coherent sources. The intensity of both sources are same. If the intensity at the point of maxima is 4Wm−2, the intensity of each source is
View Solution

