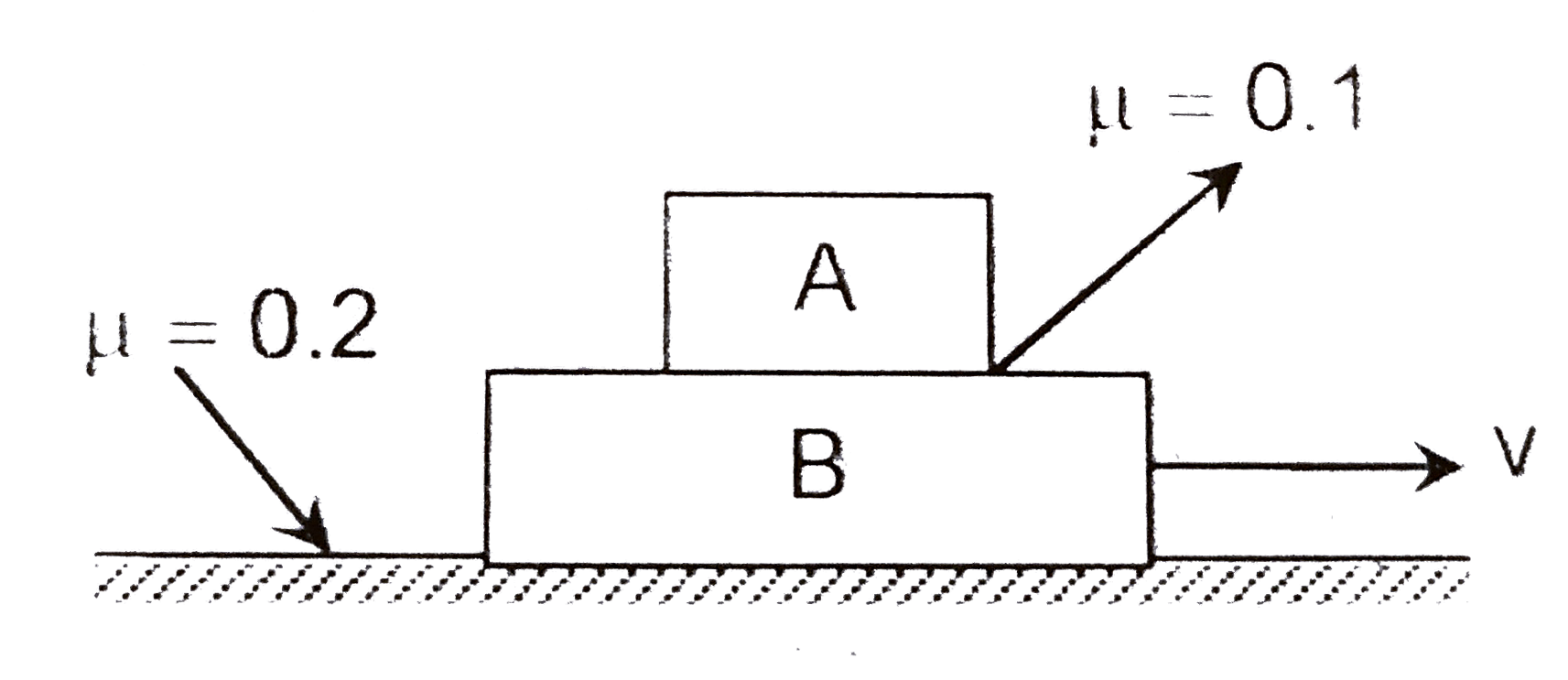
Both the blocks are given same velocity towards right. In how much time , the smaller block will separate from the large block.
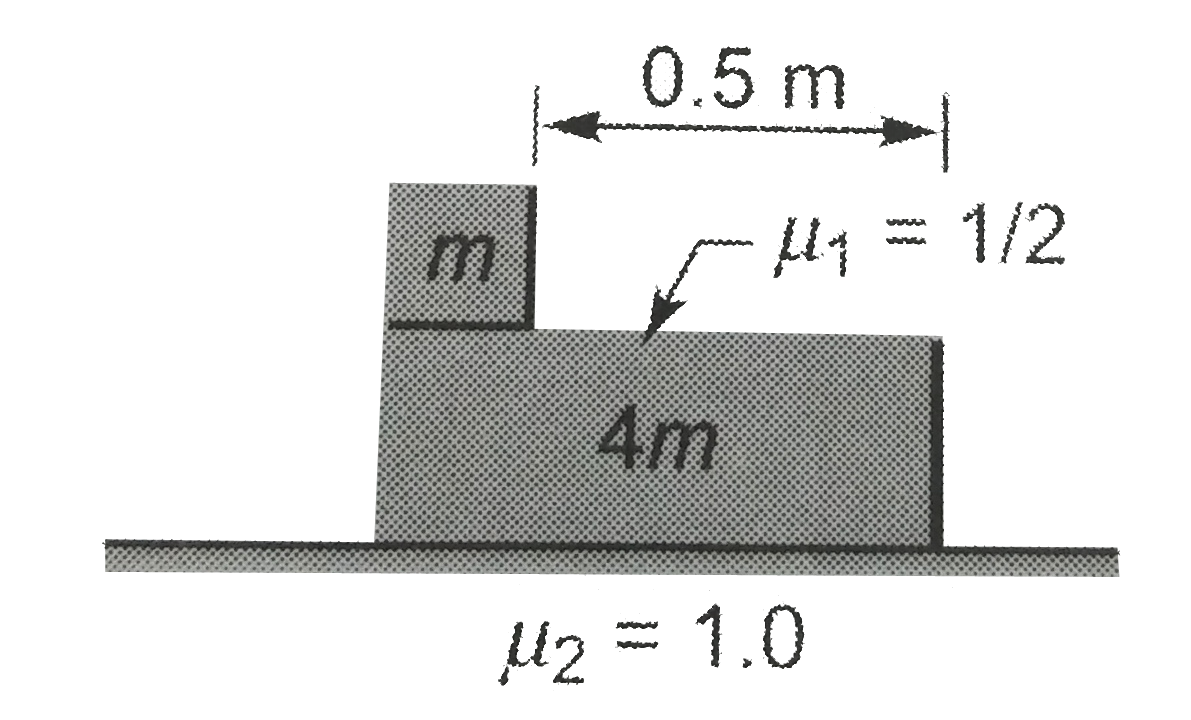
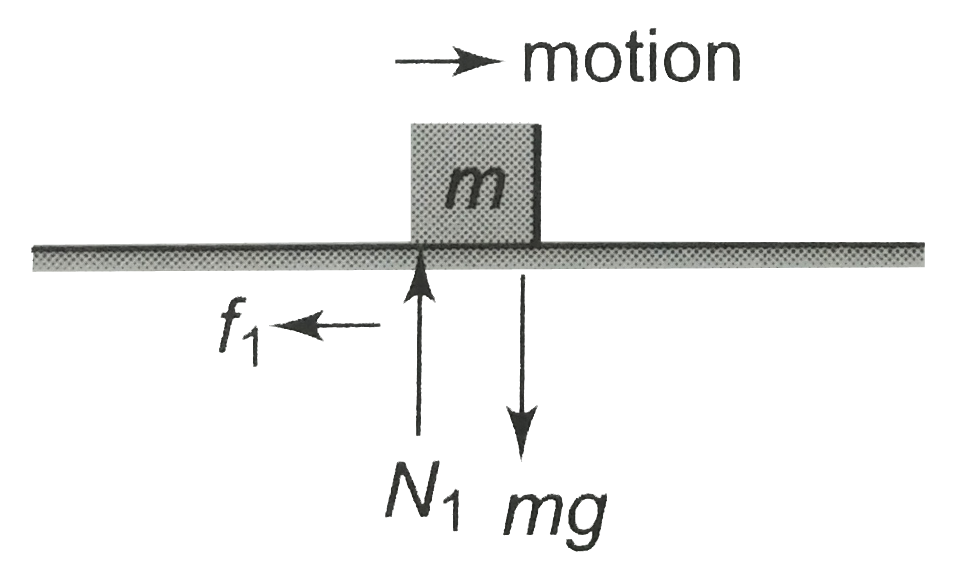
Upper block :
Retardation
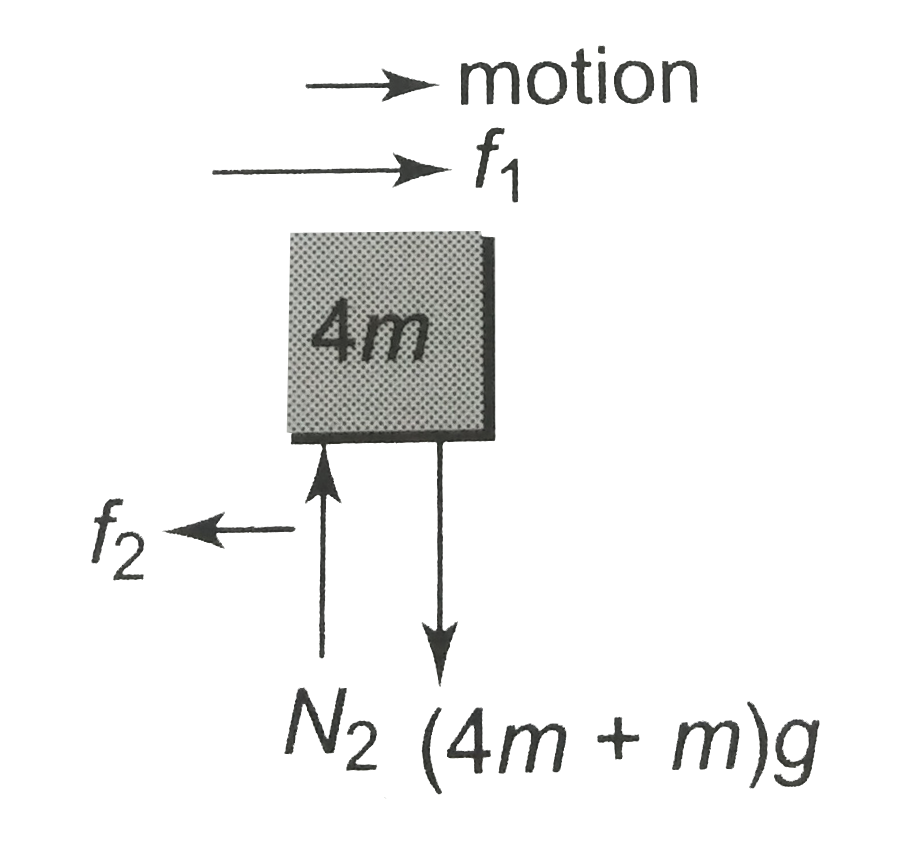
Lower block :
Retardation
, i.e. the upper block is accelerating w.r.t. the lower block.
Acceleration of the upper block with respect to the lower block
Relative velocity
Topper's Solved these Questions
Similar Questions
If the friction coefficient between the blocks are μs=0.52 and μk=0.50 , in how much time , the upper block will lose contact with the lower block.

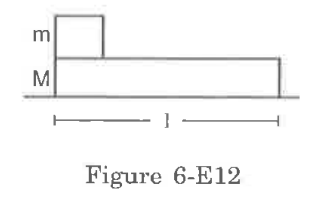
View SolutionFigure shows a small block of mass m kept at the left end of a larger block of mass M and length l. The system canslide on a horizontal road. The system is started towards right with an initia velocity v. The friction coefficient between the road and the bigger block is μ and that between the block is μ2. Find the time elapsed before the smaller blocks separates from the bigger block.
View Solution
Knowledge Check
Both the blocks as shown in figure are given together a horizontal velocity towards right. The acceleration of the centre of mass of the system of block is (mA=2mB=2kg).
 AzeroB53m/s2C73m/s2D2m/s2
AzeroB53m/s2C73m/s2D2m/s2Determine the time in which the smaller block reaches other end of bigger block in figure
 A4sB8sC2.19sD2.13s
A4sB8sC2.19sD2.13sThree blocks of masses m , m and M are kept on a frictionless floor as shown in the figure .The leftmost block is given velocity v towards the right . All the collision between the blocks are perfectly inelastic . The loss in kinetic energy after all the collision is 5/6th of initial kinetic energy. The ratio of M/m will be
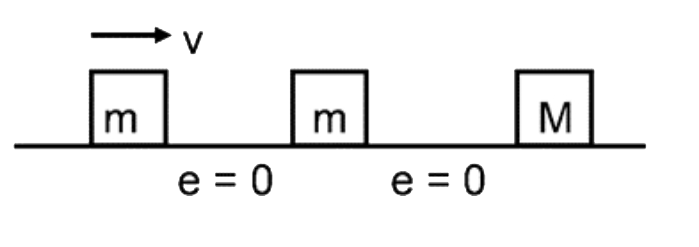 A14B18C2D4
A14B18C2D4
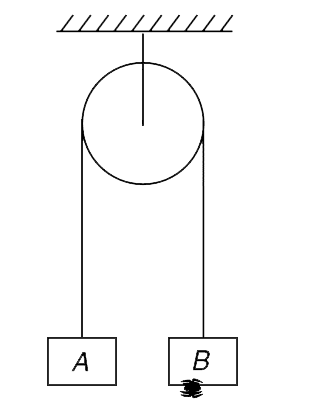
In the arrangement shown in the figure the system is in equilibrium. Mass of the block A is M and that of the insect clinging to block B is m. Pulley and string are light. The insect loses contact with the block B and begins to fall. After how much time the insect and the block B will have a separation L between them.
View SolutionInfinite blocks each of mass M are placed along a straight line with a distance d between each of them. At t = 0 the leftmost block is given a velocity V towards right. The coefficient of frication between any block and the surface is μ and all collisions are elastic. Let the total number of collisions be N then N is

View SolutionTwo blocks of masses m and M are held against a compressed spring on a frictionless horizontal floor with the help of a light thread. When the thread is cut, the smaller block leaves the spring with a velocity u relative to the larger block. Find the recoil velocity of the larger block.

View SolutionIn the figure two blocks are given velocities as shown. The lower block is very long. Find velocities of both the blocks when relative motion between them is ceased
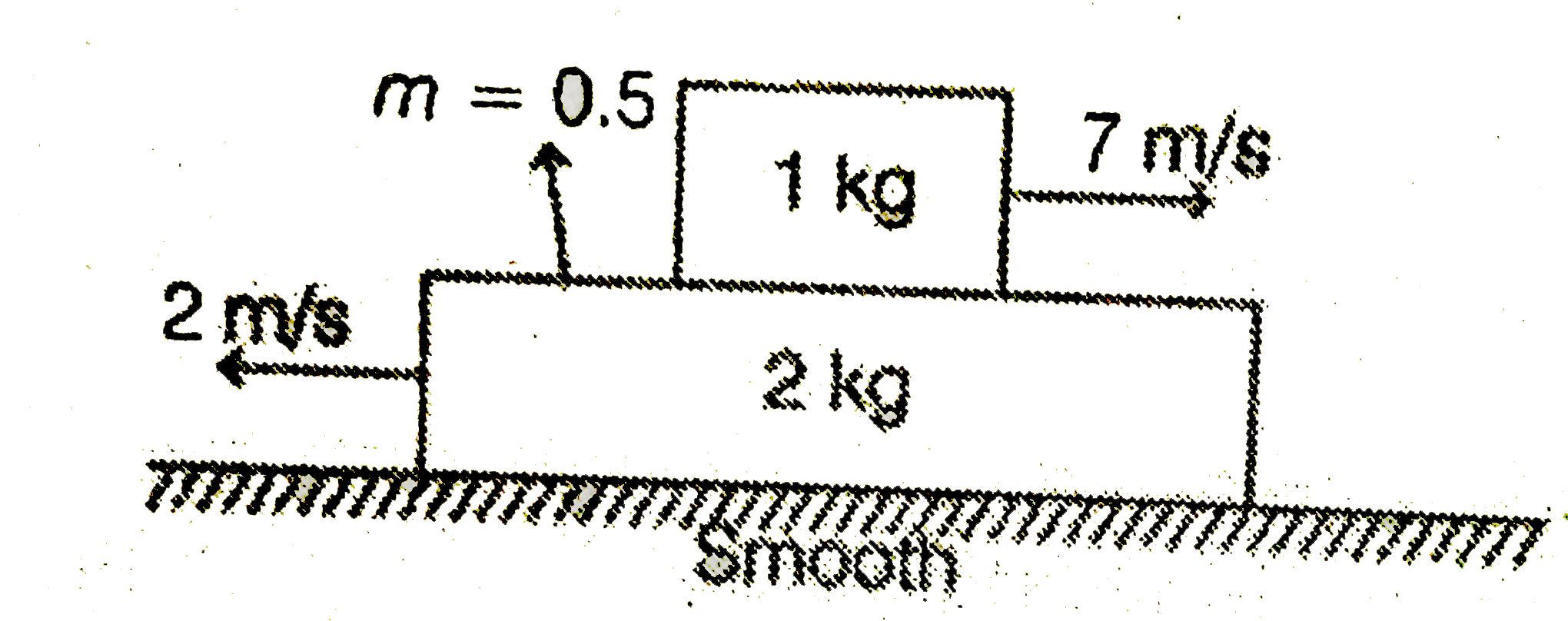
View SolutionIf block B moves towards right with acceleration b, find the net acceleration of block A.

View Solution
