If the potential energy is minimum for two atoms at r0=0.75Å. This is the equilibrium distance. Then,
the force is attractive at = r = 0.5 Å
the force is attractive at = r = 0.75 Å
the force is repulsive at r = 0.5 Å
the force is repulsive at r = 0.75 Å
The correct Answer is:C
The P.E. is minimum when the interatomic distance is r0=0.75Å
When r<r0(0.5Å<0.75Å), the force is repulsive.
When r>r0, the force is attractive.
Topper's Solved these Questions
- ELASTICITYBOOK - MARVEL PUBLICATIONCHAPTER - ELASTICITYEXERCISE - Test Your Grasp - 5
15 Videos
- CURRENT ELECTRICITYBOOK - MARVEL PUBLICATIONCHAPTER - CURRENT ELECTRICITYEXERCISE - MCQ
151 Videos
- ELECTROMAGNETIC INDUCTION AND ALTERNATING CURRENTS BOOK - MARVEL PUBLICATIONCHAPTER - ELECTROMAGNETIC INDUCTION AND ALTERNATING CURRENTS EXERCISE - TEST YOUR GRASP - 16
30 Videos
Similar Questions
What should be the orientation of a magnetic dipole in a uniform magnetic field so that its potential energy is minimum (or the dipole is in stable equilibrium) ?
View SolutionIf the potential energy between two molecules is given by U=−Ar6+Br12 , then at equilibrium , separation between molecules , and the potential energy are :
View Solution
Knowledge Check
Statement I : For the stable equilibrium force has to be zero and potential energy should be minimum.
Statement II : For the equilibrium it is not necessary that the force is not zero.AIf both Statement- I and Statement- II are true, and Statement - II is the correct explanation of Statement– I.BIf both Statement - I and Statement - II are true but Statement - II is not the correct explanation of Statement – ICIf Statement - I is true but Statement - II is false.DIf Statement - I is false but Statement - II is trueR is the interatomic distance, a and b are positive constants, u denotes potential energy which is a function dependent on R as follows v=ar10−br5.The equilibrium distance between two atoms is
A(b2a)15B(2ab)15C(b2a)110D(2ab)110The potential energy W of a system of two atoms A and B varies as a function of their distance of separation r as folllows
W=−Arn+Brm
where A' and B' are characteristie constant independent of r.
The bond distance between A and B that is dA−B is given by :AdA−B=(mB'nA')1/m−nBdA−B=(nA'mB')1/m−nCdA−B=mB'nA'DdA−B=(mB'nA')m/n
What will be the energy corresponding to the first excited state of a hydrogen atom if the potential energy of the atom is taken to be 10eV when the electron is widely separated from the proton ? Can be still write E0−E1/n2,or,rn=a0n2?
View SolutionConsidering θ=π/2 as the zero-energy position of a current loop of magnetic moment m placed in a magnetic field of strength B,find the minimum potential energy. In what position is the potential energy minimum? Is this an equilibrium position ? What will be the work done in turning it through α from this position ? Examine whether you get the dame position for equilibrium and same value for work if θ=0 is taken as the zero-energy position.
View SolutionThe potential energy W of a system of two atoms A and B varies as a function of their distance of separation r as folllows
W=−Arn+Brm
where A' and B' are characteristie constant independent of r.
The bond dissociation energy of A−B bond, DA−B is given by
View SolutionIn the potential energy curve for the formation of H2 molecule as a function of internuclear distance of H atoms, what happens at the minimum in the curve?
View SolutionThe given plot shows the variation of U, the potential energy of interaction between two particles with the distance separating them r,
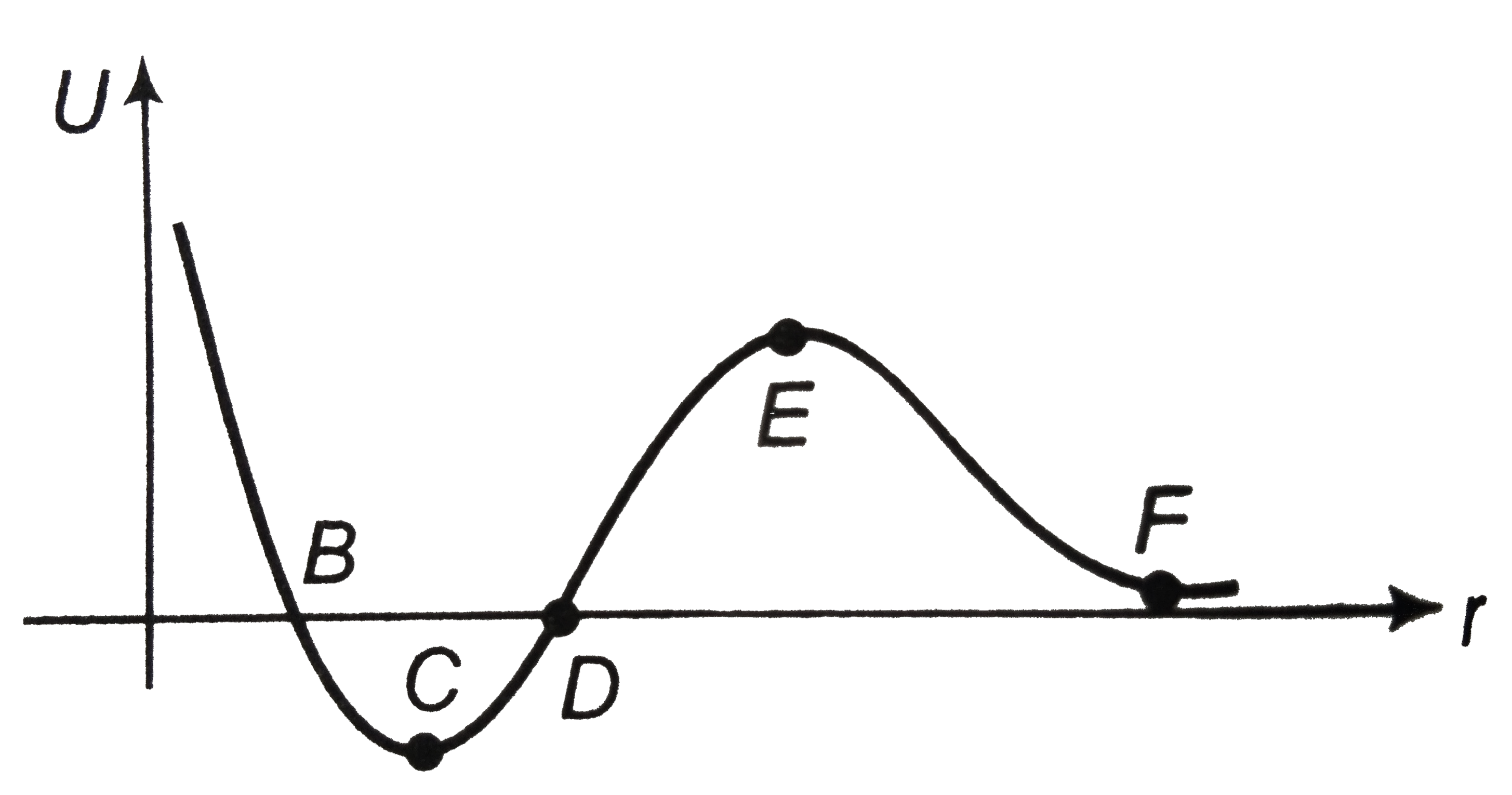
View Solution
