When a mass is suspended from the end of wire the top end of which is attached to the roof of the lift, the extension is 'e' when the lift is stationary . If the lift moves up with constant acceleartion g/2 the extension of the wire would be
2e3
3e2
2e
3e
The correct Answer is:B
e2=e1[1+ag]
Topper's Solved these Questions
- MECHANICAL PROPERTIES OF SOLIDSBOOK - NARAYNACHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - NCERT BASED QUESTIONS
21 Videos
- MECHANICAL PROPERTIES OF SOLIDSBOOK - NARAYNACHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - SINGLE ANSWER TYPE
15 Videos
- MECHANICAL PROPERTIES OF SOLIDSBOOK - NARAYNACHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - LEVEL-II (C.W)
32 Videos
- MECHANICAL PROPERTIES OF FLUIDSBOOK - NARAYNACHAPTER - MECHANICAL PROPERTIES OF FLUIDSEXERCISE - EXERCISE - III
30 Videos
- MOTION IN A PLANEBOOK - NARAYNACHAPTER - MOTION IN A PLANEEXERCISE - Level-II(H.W)
31 Videos
Similar Questions
Time period of a simple pendulum is T inside a lift when the lift is stationary. If the lift moves upwards with an acceleration g/2, the time period of pendulum will be:
View SolutionTime period of a spring pendulum when lift moves downward with constant velocity v is T second. When the lift moves upward with constant acceleration=g3 , the time period will be
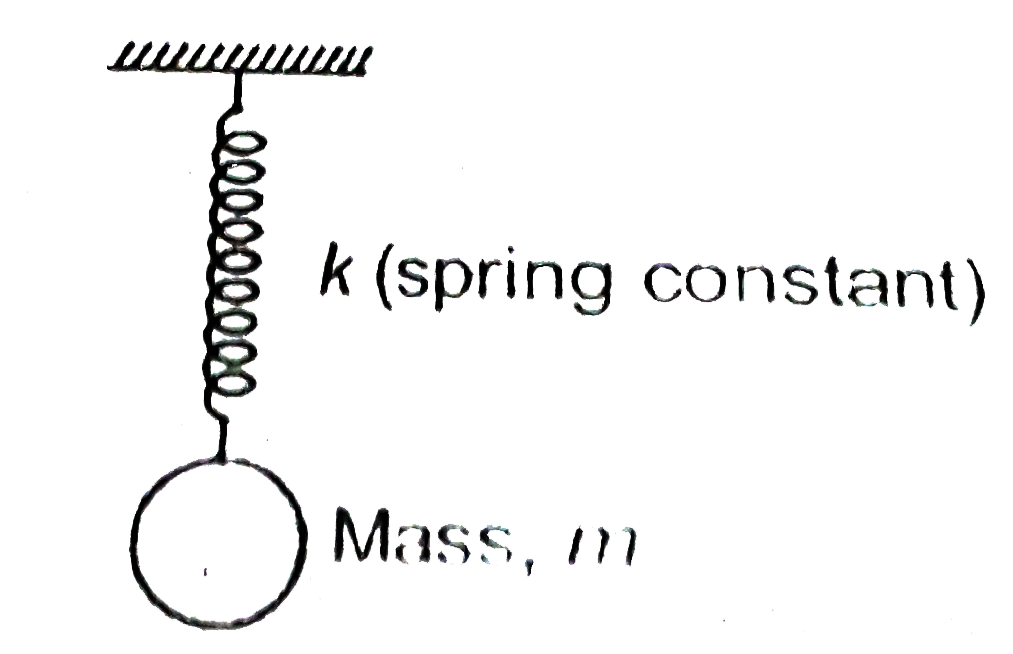
View Solution
Knowledge Check
A spring balance is attached to the ceiling of a lift. A man hangs his bag on the spring and the spring reads 49N, when the lift is stationary. If the lift moves downward with an acceleration of 5m/22, the reading of the spring balance will be
A(a) 24NB(b) 74NC(c) 15ND(d) 49NA coin is dropped in a lift. It takes time t1 to reach the floor when lift is stationary. It takes time t2 when lift is moving up with constant acceleration. Then
At1>t2Bt2>t1Ct1=t2Dt1≥t2A coin is dropped in a lift. It takes time t1 to reach the floor when lift is stationary. It takes time t2 when lift is moving up with costant acceleration. Then
At1>t2Bt2>t1Ct1=t2Dt1≥t2
A uniform rope of mass m and length L is attached to the ceiling of a lift. The other end of the rope is attached to a block of mass M. The lift is moving up with a constant acceleration a0. Find the tension in the rope at a distance x from the ceiling as shown in the diagram.
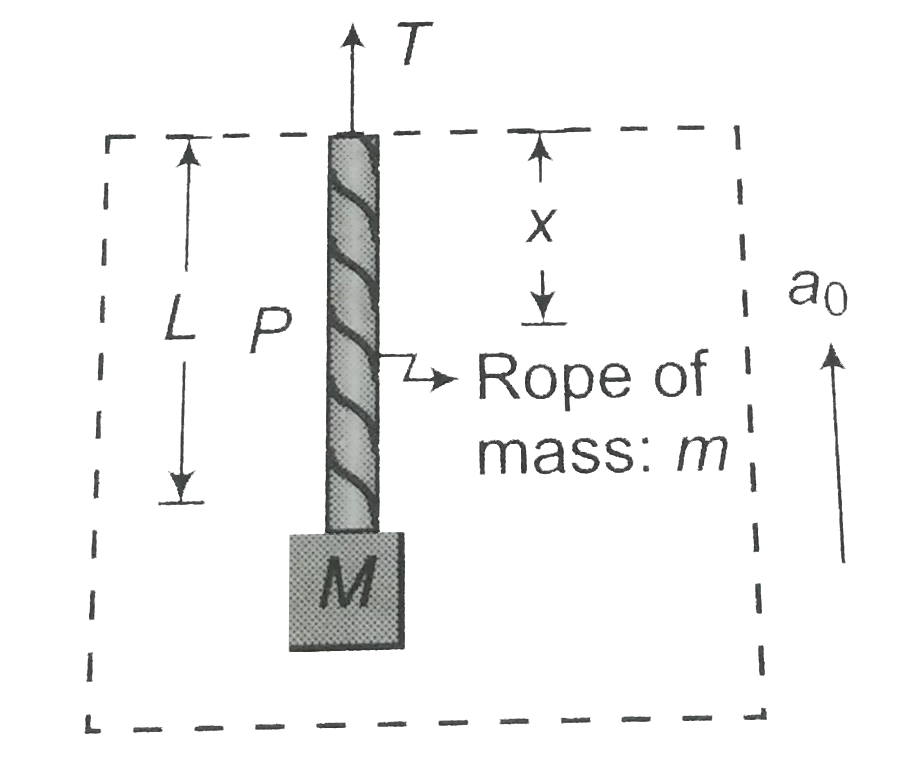
View SolutionA simple pendulum, suspended from the coiling of a lift, has a period of oscillation T, when the lift is at rest. If the lift starts moving upwards with an acceleration a =3g, then the new period will be
View SolutionA simple pendulum is attached to the roof of a lift. If time period of oscillation, when the lift is stationary is T . Then frequency of oscillation, when the lift falls freely, will be
View SolutionA spring balance is attached to the ceiling of a lift. A man hangs his bag on the spring and the spring reads 49 N, when the lift is stationary. If the moves downwards with an acceleration of 5 ms−2, then reading of the spring balance will be
View SolutionA seconds pendulum is suspended form the roof of a lift. If the lift is moving up with an acceleration 9.8m/s2, its time period is
View Solution
