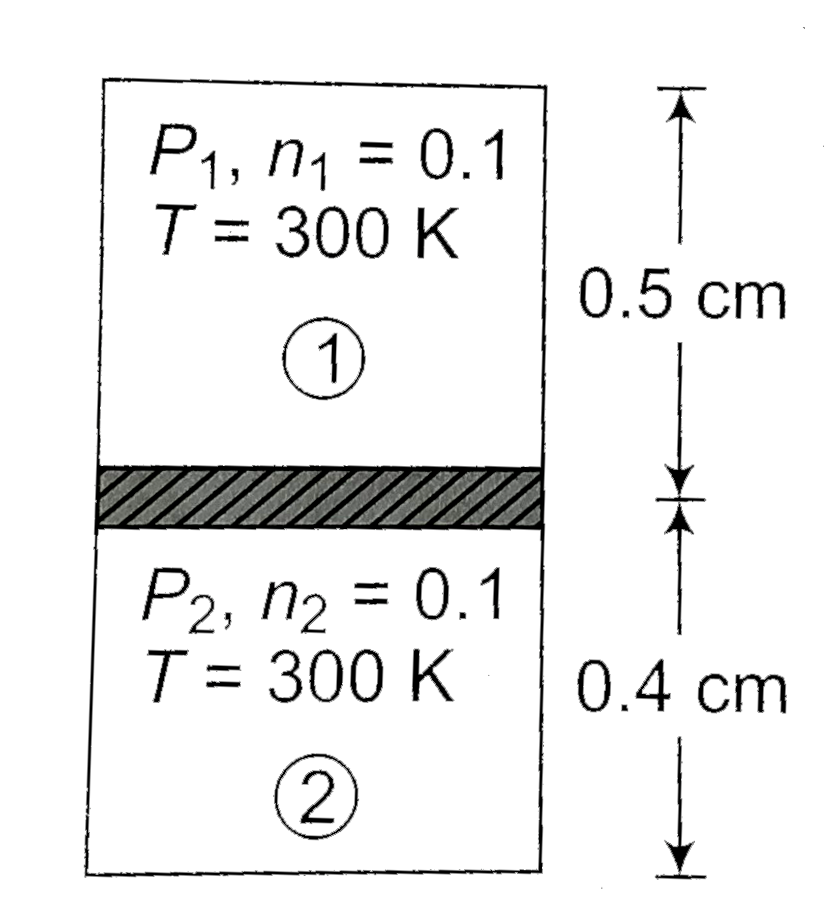
Show a vertical cylindrical vesse seperated in two parts by a frictionless piston free to move along the length of vessel. The length of the cylilender is 90 cm and the piston divides the cylinder in the ratio of 5:4. Each of the two parts of the vessel contains 0.1 mole of an ideal gas. The temoerature of the gas is 300K in each part. Calculate the mass of the piston.(figure)
m: mass of piston
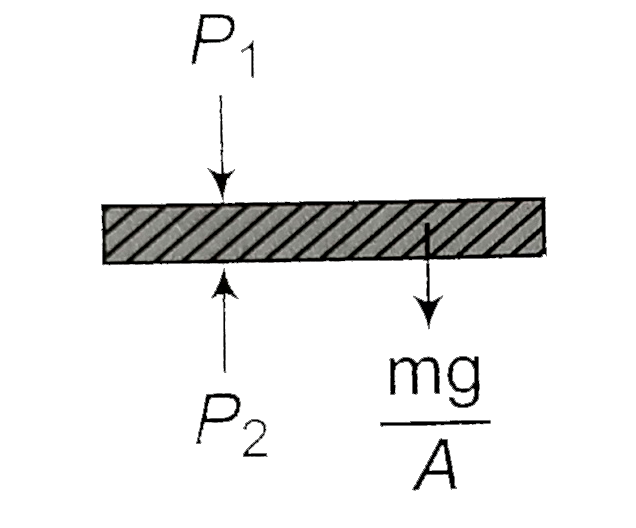
For equilibrium of piston
P1+mgA=P2
mg=(P2−P1)A (i)
Chamber ①, P1V=n1RT⇒P1=n1RTV=n1RTA×0.5
Chamber ②, P2V=n2RT⇒P2=n2RTV=n2RTA×0.4
(P2−P1)A=(n2RT0.4A−n1RT0.5A)A=0.1RT(10.4−10.5)
=0.1RT(2.5−2)=0.05RT
mg=(P2−P1)A=0.05RT
m=0.05RTg=0.05×8.3×30010
=12.45kg
Topper's Solved these Questions
Similar Questions
Draw a line segment of length 5.6 cm and divide it in the ratio 4:3. Measure the two parts.
View SolutionA thermally isolated cylindrical closed vessel of height 8 m is kept vertically . It is divided into two equal parts by a diathermic (perfect conductor) frictionless partition of mass 8.3 kg . Thus the partition is held initially at a distance of 4 m from the top , as shown in the schematic figure below . Each o the two parts of the vessel contians 0.1 mole of an ideal gas at temperature 300 K. The partition is now released and moves without any gas leaking from one part of the vessel to the other . When equilibrium is reached, the distance of the parition form one part of the vessel to the otehr . When equilibrium is reached the distacne of the partition from the top (in w) will be _______(take the accelearation due to gravity = 10 ms−2 and the universal gas constant =8.3J mol^(-1) K^(-1)).
View Solution
Knowledge Check
A vertical closed cylinder is separated into two parts by a frictionless piston of mass m and of negligible thickness. The piston is free to move along the length of the cylinder. The length of the cylinder above the piston is l1, and that below the piston is l2, such that l1>l2. Each part of the cylinder contains n moles of an ideal gas at equal temeprature T. If the pistion is stationary, its mass, m, will be given by : (R is universal gas constant and g is the acceleration due to gravitey)
ARTng[l1−3l2l1l2]BRTg[2l1+l2l1l2]CnRTg[1l2+1l1]DnRTg[l1−l2l1l2]A vertical closed cylinder is separated into two parts by a frictionless piston of mass m and of negligible thickness. The piston is free to move along the length of the cylinder. The length of the cylinder above the piston is l1, and that below the piston is l2, such that l1>l2. Each part of the cylinder contains n moles of an ideal gas at equal temeprature T. If the pistion is stationary, its mass, m, will be given by : (R is universal gas constant and g is the acceleration due to gravitey)
ARTng[l1−3l2l1l2]BRTg[2l1+l2l1l2]CnRTg[1l2+1l1]DnRTg[l1−l2l1l2]
A vertical cylinder closed at both ends is divided into two parts by a frictionless piston, each part containing one mole of air. At 300K the volume of the upper part is 4 times of the lower part. At what temperature will the volume of the upper part be three times that of the lower part?
View SolutionA line segment 56 cm long is to be divided into two parts in the ratio of 2:5. Find the length of each part.
View SolutionA vertical cylinder closed at both ends is divided into two parts by a frictionless piston, each part containing one mole of air. At 300K the volume of the upper part is 4 times of the lower part. At what temperature will the volume of the upper part be three times that of the lower part?
View SolutionA line segment 56cm long is to be divided into two parts in the ratio of 2 : 5. Find the length of each part.
View SolutionA 35-cm line segment is divided into two parts in the ratio 4:3. Find the length of each part.
View SolutionThe pressure of a gas contained in a cylinder with a movable piston is 300 Pa. The area of the piston is 0.5m2. Calculate the force that is exerted on the piston.
View Solution
