Question

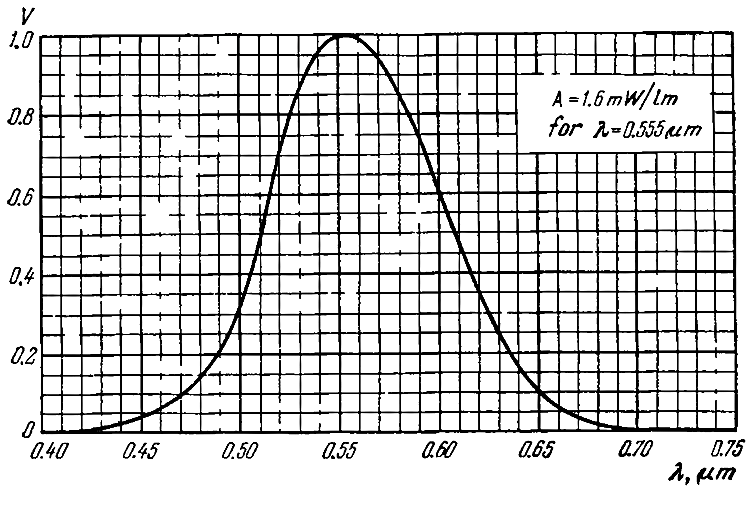
Making use of the spectral response curve for an eye (see Fig.), find :
(a) the energy flux corresponding to the luminous flux of $1.01m$ at the wavelength $0.51$ and $0.64\mu m$,
(b) the luminous flux corresponding to the wavelength interval from $0.58$ to $0.63\mu m$ if the respective energy flux, equal to $\Phi =4.5mW$, is unifromly distributed over all wavelengths of the interval. The function $V\left(\lambda \right)$ is assumed to be linear in the given spectral interval.

Explanation

The relative spectral response `V(lambda)` shown in Fig. o fthe book is so defined that `A//V (lambda)` is the enegry flux of light of wave length `lambda` needed to produce a unit luminous flux at that wavelength. (`A` is the converison factor defined in the book)
At `lambda = 0.51 mu m`, we read from the figure
`V (lambda) = 0.50` so
energy flux corresponding to a luminous flux of `1` lumen `= (1.6)/(0.50) = 3.2 mW`
At `lambda = 0.64 mu m`, we read
`V (lambda) = 0.17`
and energy flux corresponding to a luminous flux of `1` lumen `= (1.6)/(.17) = 9.4 mW`
(b) Here `d Phi_(e) (lambda) = (Phi_(e))/(lambda_(2) - lambda_(1)) d lambda, lambda_(1) le lambda le lambda_(2)`
since energy is distributied unifromly. then
`Phi = int_(lambda_(1))^(lambda_(2)) V(lambda) d Phi_(2) (lambda)//A = (Phi_(e))/(A (lambda_(2) - lambda_(1)))int_(lambda_(1))^(lambda_(2)) V(lambda) d lambda`
since `V(lambda)` is assumed to very linearly in the interval `lambda_(1) le lambda le lambda_(2)`, we have
`(1)/(lambda_(1) - lambda_(2))int_(lambda_(1))^(lambda_(2)) V (lambda)d lambda = (1)/(2) (V (lambda_(1)) + V(lambda_(2)))`
Thus, `Phi = (Phi_(e))/(2A) (V (lambda_(1)) + V (lambda_(2)))`
Using `V (0.58 mu m) = 0.85`
`V (0.63 mu m) = 0.25`
Thus `Phi = (Phi_(e))/(2xx1.6) xx 1.1 = 1.55` lumen.