Find the period of small torsional oscillational of a system consisting of two discs slipped oon a thin rod with rod with torsional coefficient . The moments of inertia of discs relative to the rod's axis are equal to and .
Suppose the disc 1 rotates by angle and the disc 2 by angle in the opposite sense. Then total torsion of the rod
and torsional
The K.E. of the system neglecting the moment of inertia of the rod is
So total energy of the rod
We can put the total angular momentum of the rod equal to zero since the frequency associated with the rigid rotation of the whole system must be zero and is known .
Thus or
So and
and
The angular oscillation, frequency corresponding to this is
and , where

Topper's Solved these Questions
- OSCILLATIONS AND WAVESBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OSCILLATIONS AND WAVESEXERCISE - Electric Oscillations
56 Videos
- OSCILLATIONS AND WAVESBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OSCILLATIONS AND WAVESEXERCISE - Elastic Waves
39 Videos
- OPTICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OPTICSEXERCISE - Exercise
2 Videos
- PHYSICAL FUNDAMENTALS OF MECHANICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - PHYSICAL FUNDAMENTALS OF MECHANICSEXERCISE - Relativistic Mechanics
49 Videos
Similar Questions
A uniform horizontal disc fixed at its centre to an elastic vertical rod performs forced torsional oscillations dur to the moment of forces Nz=Nmcosωt. The oscialltions obey the law φ=φmcos(ωt−α).
(a) the work performed by friction forces acting on the disc during one oscillation period ,
(b) the quality factor of the given oscillator if th emoment of inertia of the disc relative to the axis is equal to I.
View SolutionTwo physical pendulums perform small oscillations about the same horizontal axis with frequencies ω1 and ω2 . Their moments of inertia relative to the given axis are equal to I1 and I2 respectively. In a state of stable equilibium the pendulums were fastened rigifly together. What will be the frequency of small oscillations of the compound pendulum ?
View Solution
Knowledge Check
The moment of inertia of a thin uniform rod of mass M and length l about an axis perpendicular to the rod through its centre is I. The moment of inertia of the rod through its end point is
AI4BI2C2ID4IThree thin rods, each of length 2 m and mass 3 kg are placed along x, y and z axes, such that one end of each rod is at the origin. The moment of inertia of this system about the x axis is
A2kgm2B4kgm2C6kgm2D8kgm2Three thin rods each of length Land mass M are placed along x, y and z-axes such that one of each rod is at origin. The moment of inertia of this system about z-axis is
A2.3ML2B(4ML^(2))/3C(5ML^(2))/3D(ML^(2))/3
Moment of Inertia of system of particles : rod, ring, disc, sheet, sphere etc
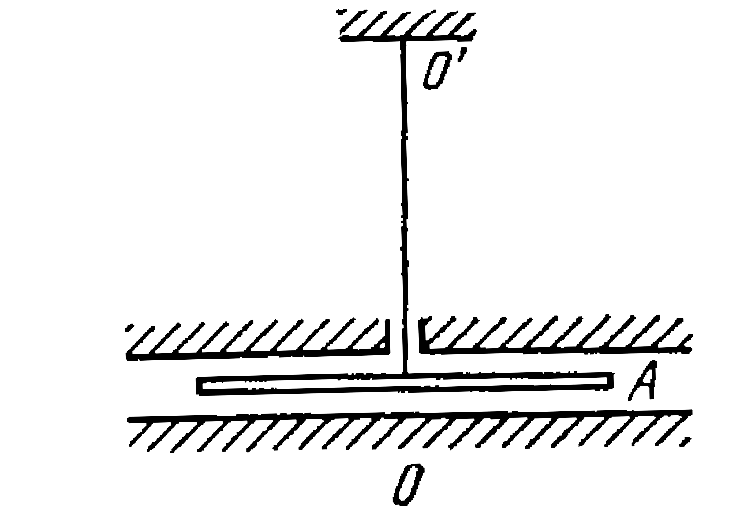
View SolutionA disc A of radius R suspended by an elastic threat between two stationary plane ( figure ) performs torsional oscillations about its axis OO′ . The moment of inertia of the disc relative to that axis is equal to I, the clearance between the disc and each of the planes is equal to h, with h<<R. Find the viscosity of the gas surrounding the disc A if the oscilaltion period of the disc equals T and the logarithmic damping decrement, λ.
View SolutionTwo horizontal discs rotate freely about a vertical axis passing through their centres. The moments of inertia of the discs relative to this axis are equal to I1 and I2, and the angular velocities to ω1 and ω2. When the upper disc fell on the lower one, both discs began rotating, after some time, as a single whole (due to friction). Find:
(a) teh steady-state angular rotation velocity of the discs,
(b) the work performed by the friction forces in this process.
View SolutionThe moment of inertia of a thin uniform rod of mass M and length L about an axis perpendicular to the rod, through its centre is I. The moment of inertia of the rod about an axis perpendicular to rod through its end point is
View SolutionMoment of inertia of a thin uniform rod about an axis passing through one end perpendicular to its length is I . Then moment of inertia the same rod about the central axis perpendicular to its plane is
View Solution
