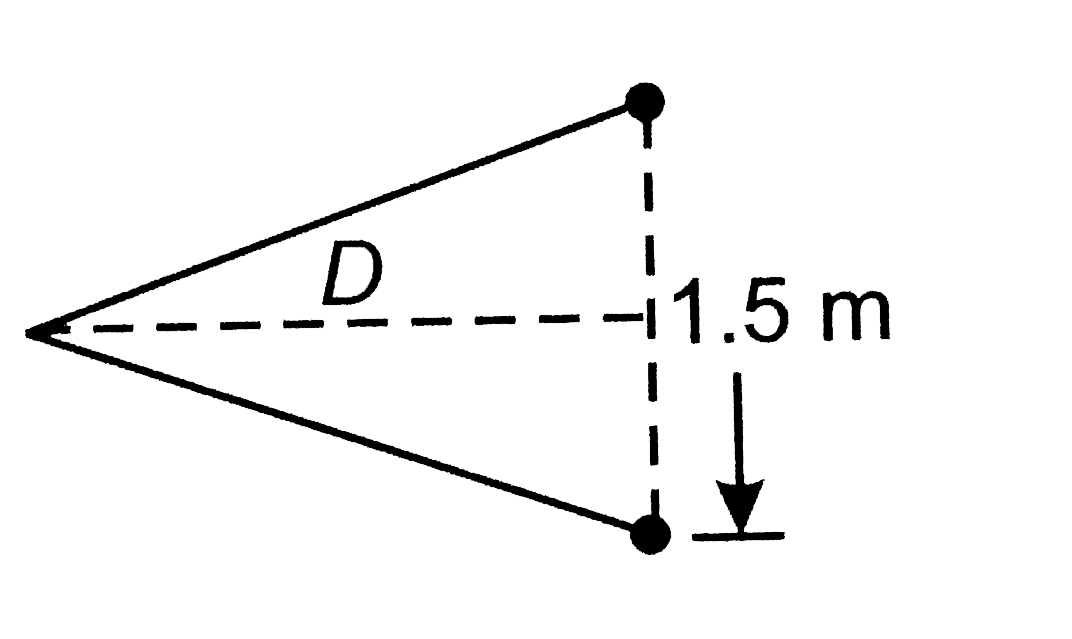
For the situation of the previous question, find the maximum distance at which the eye would be able to resolve the two headlights of a car which are at a distance of $1.5 m$ .

(a) $4000 m$

(b) $1200 n m$

(d) $25 m$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:B

Updated on:21/07/2023

A person's salary has increased from rs. 14400 to rs. 16200. What is the percentage increase in his salary?
Aa. 25%
Bb. 12.5%
Cc. 20%
Dd. 35%
In the previous question ( number 19). Find the probability that none of the two will be able to solve the problem.
A $\frac{13}{15}$
B $\frac{4}{15}$
C $\frac{2}{15}$
D $\frac{23}{30}$
In previous question, if the total distance covered by the car is s, the maximum speed attained by it will be :
A ${(2 s . \frac{a_{1} a_{2}}{a_{1} + a_{2}})}^{\frac{1}{2}}$
B ${(2 s . \frac{a_{1} a_{2}}{a_{1} - a_{2}})}^{\frac{1}{2}}$
C ${(\frac{s}{2} . \frac{a_{1} a_{2}}{a_{1} + a_{2}})}^{\frac{1}{2}}$
D ${(\frac{s}{2} . \frac{a_{1} a_{2}}{a_{1} - a_{2}})}^{\frac{1}{2}}$