A river $500 m$ wide flows at a rate at a rate of $4 k m h^{- 1}$ . A swimmer who can swim at $8 k m h^{- 1}$ . In still water, wishes to cross the river straight. (i) Along what direction must he strike? (ii) What should be his resultant velocity. (iii) What is the time of crossing the river?

Video Solution

Text Solution

Verified by Experts

(i) Refer to fig. 2(c ) 22,
velocity of river $= 4 k m h^{- 1} = (O A)$
Velcity of swinmmer in still water
$v_{s 0 = 8 k m h^{- 10 = (O B)}}$
The swimmer will cross the river straitght if the resultant velocity $\vec{v} o f {\vec{v}}_{r} and {\vec{v}}_{s}$ is perpendiculat to the bank fof the river, i.e., along $(\vec{O} C)$ . This will be possible if the swimmer groes upatream of the river along $O B$ , making an angle $θ$ with $O C$ . In right $Δ O C B$ , sin $θ \frac{N C}{O B} = \frac{v_{r}}{v_{s 0 = \frac{4}{8} = \frac{1}{2} = {\sin 30}^{00}}}$
:. $θ = 30^{00}$ (ii) Resultant velocity of the swimmer
$v = \sqrt{v_{s}^{2} - v_{r}^{2}} = \sqrt{8^{20 - 4^{2}} = \sqrt{48} = 4 \sqrt{3} k m h^{- 1} = 4 \sqrt{3} \times 5 / 18 = 1.92 m / s < b r > (i i i) T i m e t a k e n \to c r o s s t h e r i v e r,}$ t=(width of river)/v =(500 m)/(1.92 ms^(-1)) =260.4 s.
.

Updated on:21/07/2023

|

Class 11 PHYSICS RECTILINEAR MOTION

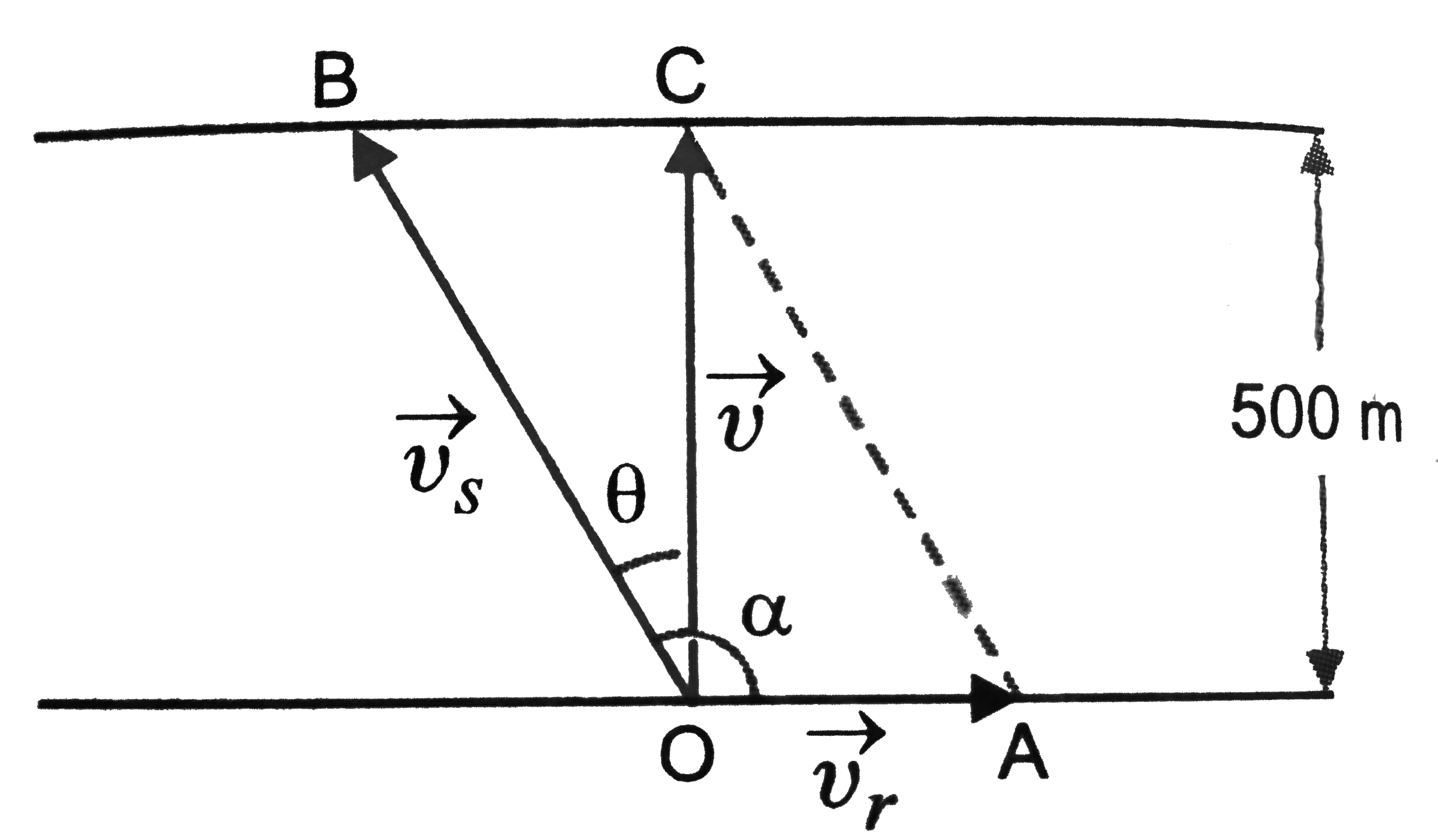Topper's Solved these Questions

Similar Questions

PRADEEP-KINEMATICS-1 NCERT Comprehension

Home
Ask Doubt
Profile