a long coaxial cable consists of two thin-walled conducting cylinder carries a steady current 0.1A, and the outer cylinder provides the return path for that current. The current produced a magnetic field between the two cylinders. Find the energy stored in the magnetic field for length 5m of the cable. Express answer in Nj (use 1n2=0.7).
The correct Answer is:7
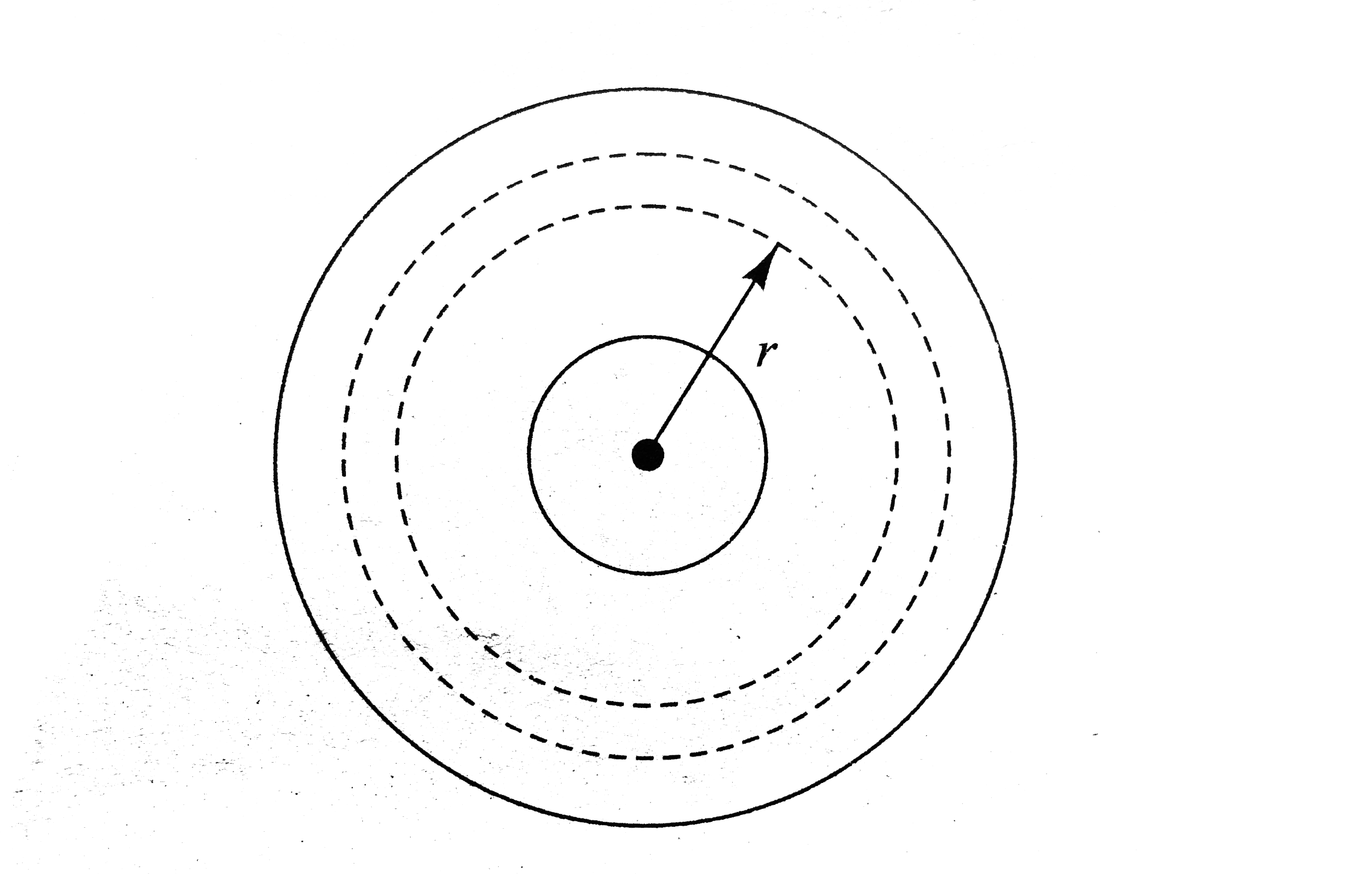
The magnetic field inside is only due to the current of the inner cylinder.
B=μ0i2πr
Magnetic field energy density is not uniform in the space between the cylinders. At a distance r from the centre,
uB=B22μ0=μ0i28π2r2
Energy in volume of element (length l)
dUB=uBdV=μ0i28π2r2(2πrl)dr=μ0i2l4πdrr
UB=μ0i2l4π∫badrr=μ0i2l4πln(b)a
Using values, we get U=7nJ
Topper's Solved these Questions
- ELECTROMAGNETIC INDUCTIONBOOK - CENGAGE PHYSICSCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Archives Fill In The Blanks
3 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - CENGAGE PHYSICSCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Archives Single Correct
11 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - CENGAGE PHYSICSCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Exercises Linked Comprehension
36 Videos
- ELECTROMAGENTIC INDUCTIONBOOK - CENGAGE PHYSICSCHAPTER - ELECTROMAGENTIC INDUCTIONEXERCISE - QUESTION BANK
40 Videos
- ELECTRON,PHONTS,PHOTOELECTRIC EFFECT & X-RAYSBOOK - CENGAGE PHYSICSCHAPTER - ELECTRON,PHONTS,PHOTOELECTRIC EFFECT & X-RAYSEXERCISE - dpp 3.3
15 Videos
Similar Questions
a long coaxial cable consists of two thin-walled conducting cylinder carries a steady current 0.1A, and the outer cylinder provides the return path for that current. The current produced a magnetic field between the two cylinders. Find the energy stored in the magnetic field for length 5m of the cable. Express answer in Nj (use 1n2=0.7).
View SolutionA long coaxial cable consists of two concentric cylinders of radii a and b. The central conductor of the cable carries a steady current I and the outer conductor provides the return path of the current. Calculate the energy stored in the magnetic field of length l of such a cable
View Solution
Knowledge Check
Find the energy stored in the magnetic field if current of 5A produces a magnetic flux of 2×10−3 Wb through a coil of 500 turns.
A2.5JB0.25JC250JD1.5JA coaxial cable consists of a thin inner current carrying conductor fixed along the axis of a hollow current conductor, Let B1 and B2 be the magnetic fields in the region between the conductors, and outside the conductor respectively.
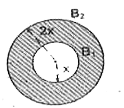 AB1≠0,B2≠0 for conductors carrying equal currents in opposite directionsBB1≠),B2=0, for conductors carrying equal currents in opposite directionsCB1≠0,B2≠0, for conductors carrying equal currents in same directionsDB1=B2 for conductors carrying equal currents in same directions at distance x and 2x from the axis respectively. 2x gt the radius of the outer cable and x lies between the cables.
AB1≠0,B2≠0 for conductors carrying equal currents in opposite directionsBB1≠),B2=0, for conductors carrying equal currents in opposite directionsCB1≠0,B2≠0, for conductors carrying equal currents in same directionsDB1=B2 for conductors carrying equal currents in same directions at distance x and 2x from the axis respectively. 2x gt the radius of the outer cable and x lies between the cables.The figure shows the cross-section of a long conducting cylinder of inner radius a and outer radius b. The cylinder carries a current whose current density J=Cr2 where C is a constant. What is the magnitude of the magnetic field B at a point r, where a<r<b?
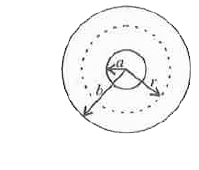 AB=μ0πC4πr2(r2−a2)BB=μ0πC4πr2(r4−a4)CB=μ0πC4π(r2−a2)DB=μ0πC4π(r4−a4)
AB=μ0πC4πr2(r2−a2)BB=μ0πC4πr2(r4−a4)CB=μ0πC4π(r2−a2)DB=μ0πC4π(r4−a4)
There is one long coaxial cable which consists of two concentric cylinders of radii a and b. Central conductor carries steady current I and outer conductor acts as return path for the current. (i) Calculate energy stored in the magnetic field of length l of such a cable. (ii) Calculate self-inductance for l length of this cable.
View SolutionFind the inductance of a unit length of a cable consisting of two thin-walled coaxial metallic cylinders if the radius of the outside cylinder is η=3.6 times that of the inside one. The permeabitity of a medium between the cylinders is assumed to be equal to unity.
View SolutionFind the self inductance of a unit length of a cable consisting of two thin walled coaxial metallic cylinders if the radius of the outside cylinder is η(η>1) times that of the inside one. The permeability of the medium between the cylinders is assumed to be equal to unity.
View SolutionA long wire carries a current of 4.00 A. Find the energy stored in the magnetic field inside a volume of 1.mm3 at a distance of 10.0 cm from the wire.
View SolutionTwo concentric coils carry the same current in opposite directions. The diameter of the inner coil is equal currents. The outer coil. If the magnetic field produced by the outer coil at the common centre are 1T, the net field at the centre is
View Solution
