In a modified YDSE, the region between the screen and slits is immersed in a liquid whose refractive index varies with time as μ1=(5/2)−(T/4) until it reaches s steady state value of 5/4. A glass plate of thickness 36 μm and refractive index 3/2 is introduced in front of one of the slits.

What is the speed of the central maxima when it is at
3×10−3ms−1
4×10−3ms−1
6×10−3ms−1
1×10−3ms−1
The correct Answer is:a
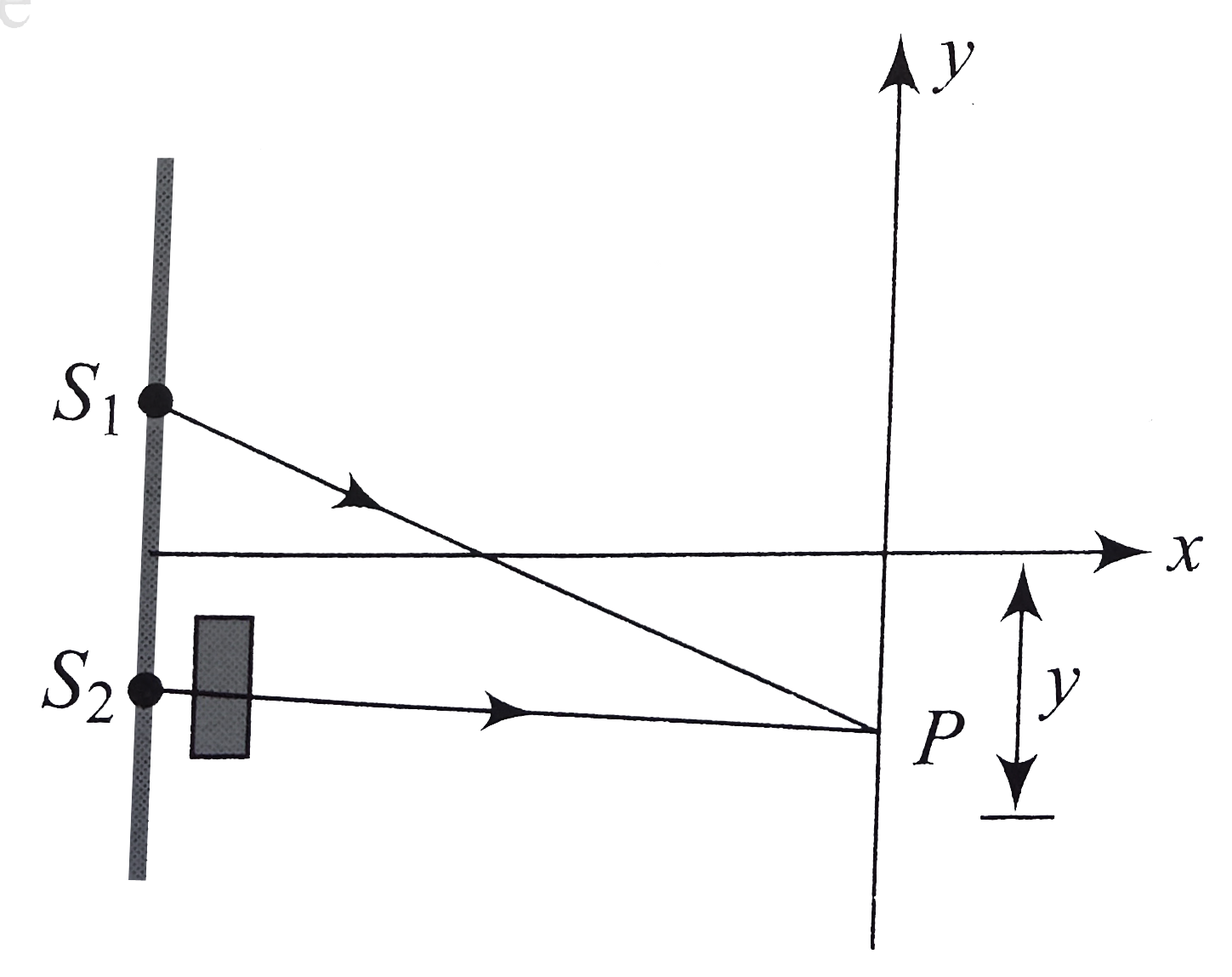
We consider a point P on the screen.
Optical path length,
[S1P]liquid=[μ1S1P]air
[S1P−t]liquid+tglass=μ[S1P−t]air+[μgt]air
=[μ1S2P+(μg−μ1)t]air
Hence, optical path difference at P,
Δx=[μ1S2P+(μg−μ1)t]−μ1S1P
=μ1(S1P−S1P)+(μg+μ1)t
For a point P at the screen in the absence of liquid,
S2P=S1P=ydD
If a liquid is filled,
[S2P−S1P]liquid=μ1ydD
Thus,
Δx=μ1ydD+[μg−μt]t
a. For central maxima,
Δx=0
y=[μg−μ1μ1]tDd=(4−T)tD(10−T)d
At Point O, y=0
Thus, T=4
b. Speed of central maxima,
v=∣∣∣dydt∣∣∣=6Dt(10−T)2d
Central maxima is at O at time t=4 s.
∴v=6Dt36D=1×36×10−66×2×10−3=3×10−3ms−1
Topper's Solved these Questions
- WAVE OPTICSBOOK - CENGAGE PHYSICSCHAPTER - WAVE OPTICSEXERCISE - Integer
3 Videos
- WAVE OPTICSBOOK - CENGAGE PHYSICSCHAPTER - WAVE OPTICSEXERCISE - Archives
4 Videos
- WAVE OPTICSBOOK - CENGAGE PHYSICSCHAPTER - WAVE OPTICSEXERCISE - Assertion- Reasoning
13 Videos
- THERMODYNAMICSBOOK - CENGAGE PHYSICSCHAPTER - THERMODYNAMICSEXERCISE - QUESTION BANK
34 Videos
- WAVESBOOK - CENGAGE PHYSICSCHAPTER - WAVESEXERCISE - QUESTION BANK
38 Videos
Similar Questions
Knowledge Check
In a modified YDSE, the region between the screen and slits is immersed in a liquid whose refractive index varies with time as μ1=(5/2)−(T/4) until it reaches s steady state value of 5/4. A glass plate of thickness 36 μm and refractive index 3/2 is introduced in front of one of the slits.
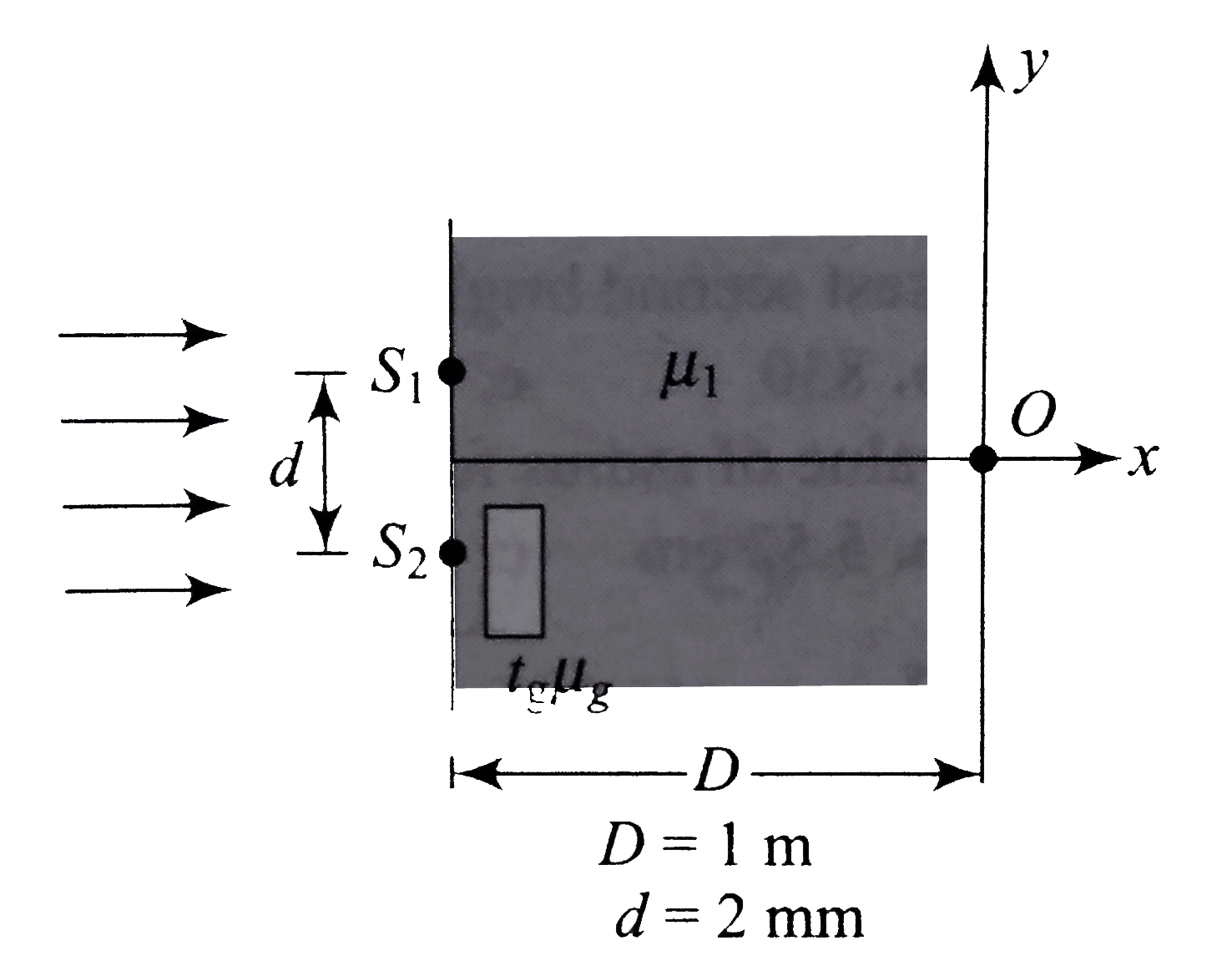
Find the time when central maxima is at point O. located symmetrically on the x-asix.A2 sB4 sC6 sD8 sA double slit experiment is performed with light of wavelength 500 nm A thin film of thickness 2 μm and refractive index 1.5 is introduced in the path of the upper beam the location of the central maximum will
Aremain unshiftedBshift downward by nearly two fringesCshift upward by nearly two fringesDshift downward by its fringesA double slit experiment is performed with light of wavelength 500 nm A thin film of thickness 2 μm and refractive index 1.5 is introduced in the path of the upper beam the location of the central maximum will
Aremain unshiftedBshift downward by nearly two fringesCshift upward by nearly two fringesDshift downward by its fringes
In the Young's double slit experiment , a mica slip of thickness t and refractive index μ is introduced in the ray from first source S1 . By how much distance fringes pattern will be displaced ? (d = distance between the slits and D is the distance between slits and screen)
View SolutionIn YDSE when slab of thickness t and refractive index μ is placed in front of one slit then central maxima shifts by one fringe width. Find out t in terms of λ and μ.
View SolutionIn Young's double-slit experiment, let A and B be the two slit. A thin film of thickness t and refractive index μ is placed in front of A. Let β= fringe width. Then the central maxima will shift
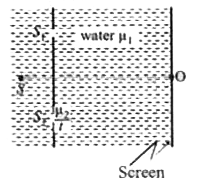
View SolutionA YDSE is conducted in water (μ1) as shown in figure. A glass plate of thickness t and refractive index μ2 is placed in the path of S2. The optical path difference at O is
View SolutionLight travels through a glass plate of thickness t and refractive index μ. If c is the speed of light in vacuum, the time taken by light to travel this thickness of glass is
View Solution
