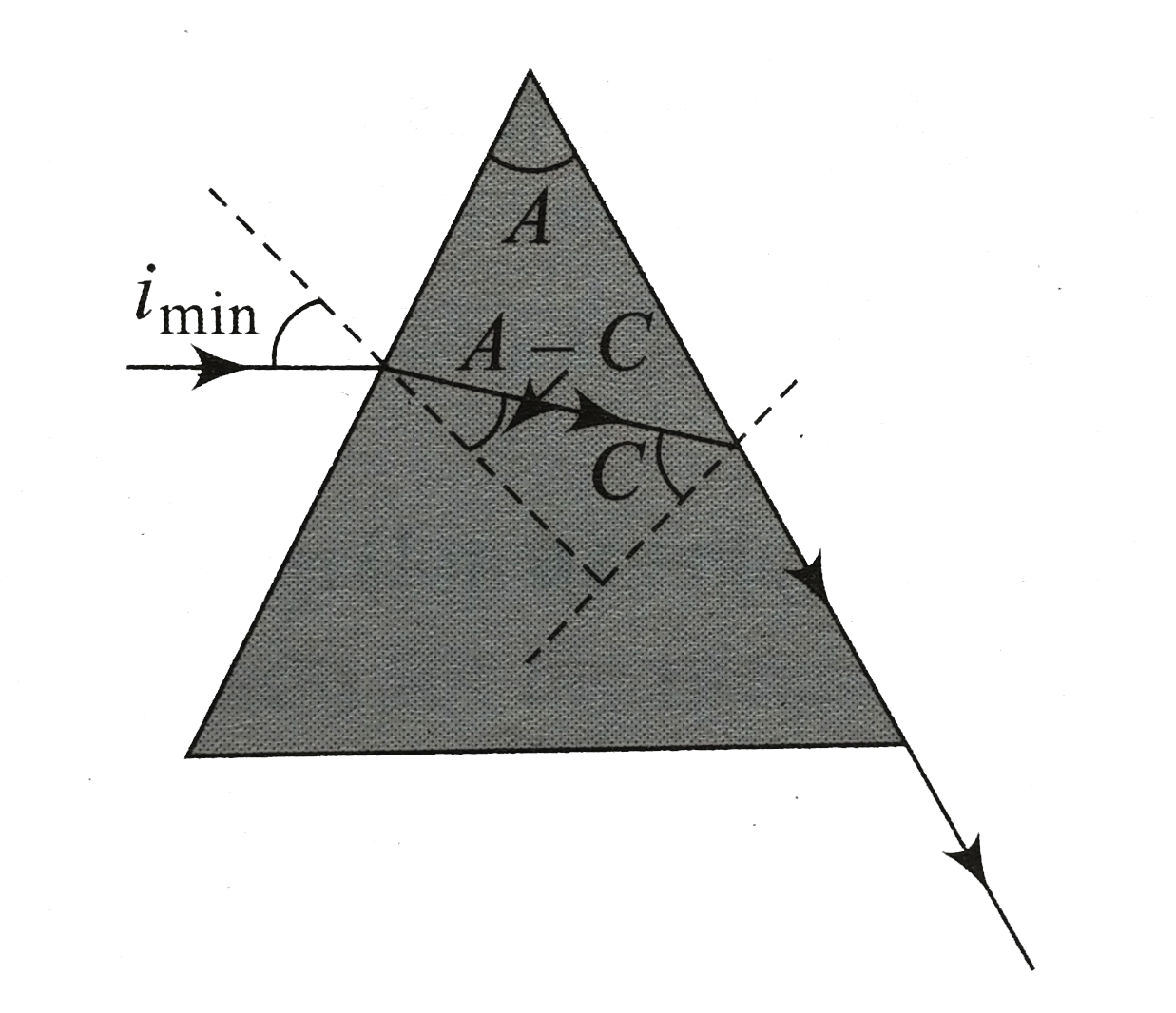
For a prism, A=60^(@), n=sqrt(7//3) . Find the minimum possible angle

Light ray is incident on a prism of angle $A = 60^{\circ}$ are refractive index $μ = \sqrt{2}$ . The angle of incidence which the emergent rays grazes the surface is given
A ${sin}^{- 1} (\frac{\sqrt{3} - 1}{2})$
B ${sin}^{- 1} (\frac{\sqrt{2} - 1}{2})$
C ${sin}^{- 1} (\frac{\sqrt{3}}{2})$
D ${sin}^{- 1} (\frac{2}{3 \sqrt{3}})$
Light ray is incident on a prism of angle $A = 60^{\circ}$ and refractive index $μ = \sqrt{2}$ .the angle of incidence at which the emergent ray grazes the surface is given by
A ${sin}^{- 1} (\frac{\sqrt{3} - 1}{2})$
B ${sin}^{- 1} (\frac{1 - \sqrt{3}}{2})$
C ${sin}^{- 1} (\frac{\sqrt{3}}{2})$
D ${sin}^{- 1} (\frac{2}{\sqrt{3}})$
Refractive index of a prism is $\sqrt{7 / 3}$ and the angle of prism is $60^{\circ}$ . The minimum angle of incidence of a ray that will be transmitted through the prism is
A $30^{\circ}$
B $45^{\circ}$
C $15^{\circ}$
D $50^{\circ}$

For a prism, $A = 60^{\circ}, n = \sqrt{7 / 3}$ . Find the minimum possible angle of incidence, so that the light ray is refracted from the second surface. Also, find $δ_{max}$ .