For a cicuit shown in fing 5.235 swhitch S1isclosedatt=0, then at t=(2R2+R1)C,S1 is opened and S2 is closed.

a. Find the charge on capactior at t=(2R2+2R1)C.
b. Find current through R2 (adjacent to the battery) at t=(3R1+2R2)C.
a. For t =0 to t=(2R2+R1)C, capacitor gets charged from all
the three resistors. So
q=CE[1−et(2R2+R1)C].
Putting t=(2R2+R1)C, we get
q1=CE[1−e−1]=CE(e−1)e
Now battery is disconnected and the capacitor gets discharged
through R1 after S1 is opened and S2 is closed. So
∫q2q1dqq−∫(2R2+2R1)C(2R2+R1)CdtR1C or q2=q1e=CE(e−1)e2
b. Since battery is disconnected at this time, there is no current
in R2
Topper's Solved these Questions
- ELECTRIC CURRENT AND CIRCUITBOOK - CENGAGE PHYSICSCHAPTER - ELECTRIC CURRENT AND CIRCUITEXERCISE - Single Correct
72 Videos
- ELECTRIC CURRENT AND CIRCUITBOOK - CENGAGE PHYSICSCHAPTER - ELECTRIC CURRENT AND CIRCUITEXERCISE - Multiple Correct
16 Videos
- ELECTRIC CURRENT AND CIRCUITBOOK - CENGAGE PHYSICSCHAPTER - ELECTRIC CURRENT AND CIRCUITEXERCISE - Exercise 5.2
50 Videos
- ELECTRIC CURRENT & CIRCUITSBOOK - CENGAGE PHYSICSCHAPTER - ELECTRIC CURRENT & CIRCUITSEXERCISE - Kirchhoff s law and simple circuits
15 Videos
- ELECTRIC FLUX AND GAUSS LAWBOOK - CENGAGE PHYSICSCHAPTER - ELECTRIC FLUX AND GAUSS LAWEXERCISE - MCQ s
38 Videos
Similar Questions
Knowledge Check
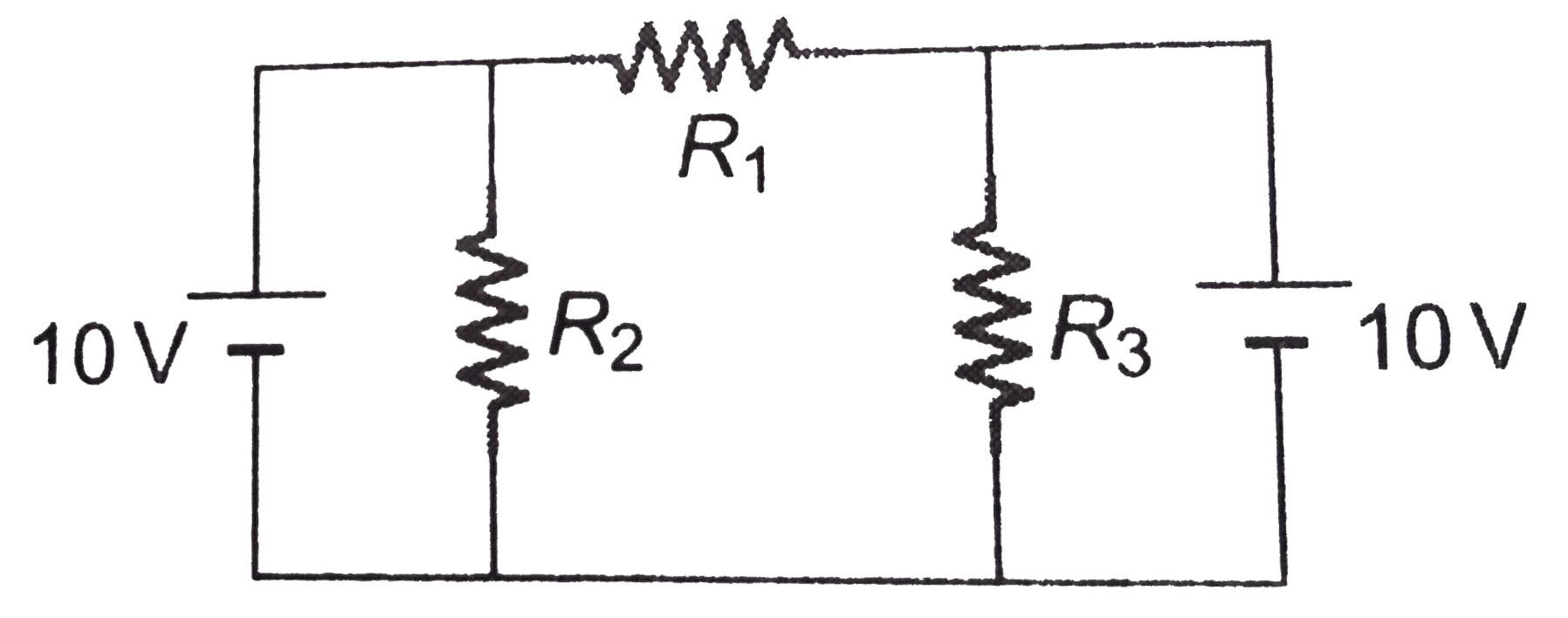
In the circuit shown below the switch is closed at t=0. For 0<t<R(C1+C2), the current I1 in the capacitor C1 in terms of total current I is
 A(C1C2)IB(C2C1)IC(C1C1+C2)ID(C1(C1+C2))I
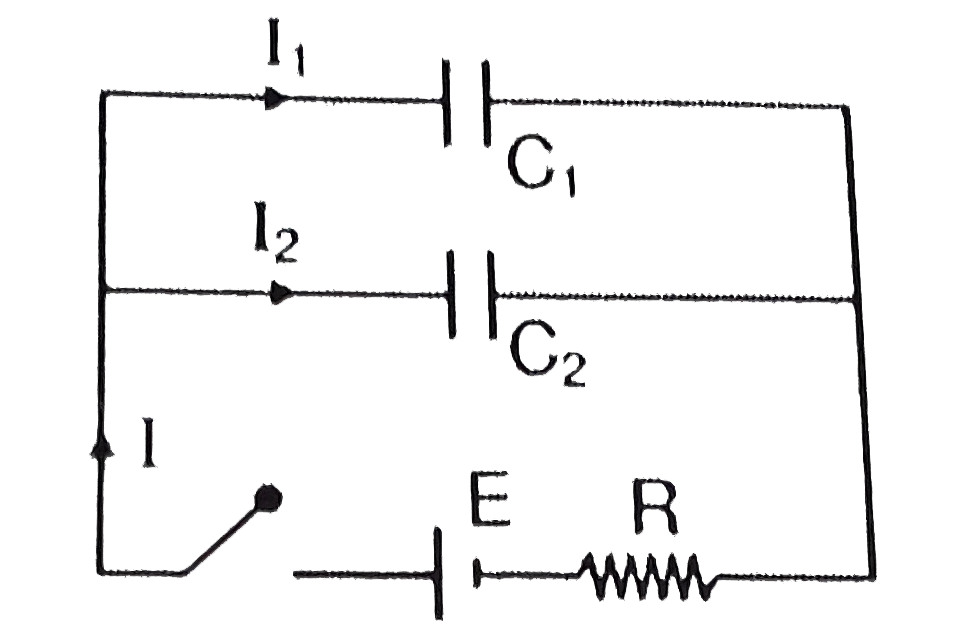
A(C1C2)IB(C2C1)IC(C1C1+C2)ID(C1(C1+C2))IInitially the switch 'S' is open for a ling time. Now the switch 'S' is closed at t=0(R1=2Ω,R2=2Ω,C1=1F and C2=1F) Then
 AThe current through the wire AB at t=2 sec is 1/2e ampereBThe current through the wire at t=2 sec is 1/e ampere.CThe charge on the capacitor C2, which has initially unchargd at t=2 sec is (1−12e) coulimbsDThe charge on the capacitor C1 at t=2 sec is (1−12e) coulombs
AThe current through the wire AB at t=2 sec is 1/2e ampereBThe current through the wire at t=2 sec is 1/e ampere.CThe charge on the capacitor C2, which has initially unchargd at t=2 sec is (1−12e) coulimbsDThe charge on the capacitor C1 at t=2 sec is (1−12e) coulombs
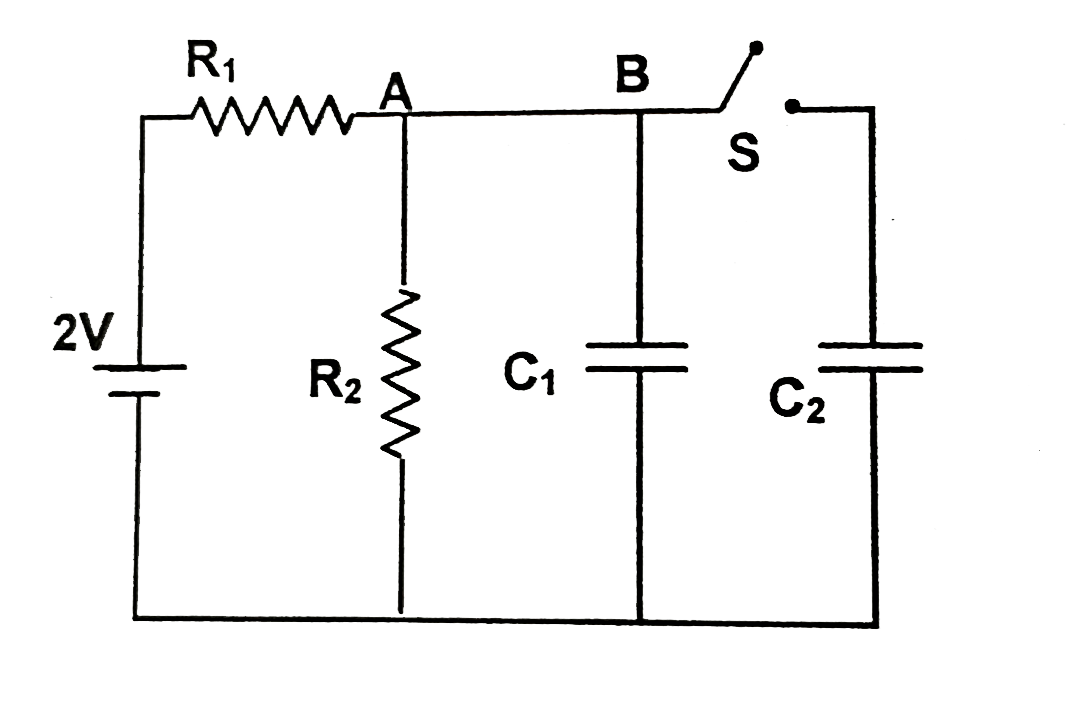
At t =0 switch S1 is closed and remains closed for a long time and S2 remains open. Now S1 is opened and S2 is closed. Find out
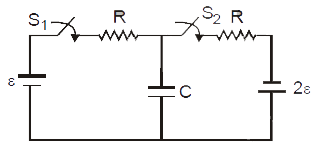
Charge on the capacitor long after that moment.
View SolutionThe capacitor shown in figure has been charged to a potential difference of V volt, so that it carries a charge CV with both the switches S1andS2 remaining open. Switch S1 is closed at t=0. At t=R1C switch S1 is opened and S2 is closed. Find the charge on the capacitor at t=2R1C+R2C.

View SolutionThe given R-C circuit has two swithes S1andS2 Swithc S2 is clsoed and S1 is open till the capacitor is fully charged to q0 the S2 is opened and S1 is closed simultaneously till the charge on capacitor remains q0/2 for which it takes time t1. Now S1 is again opened, and S2 is closed till chrage on capacitor becomes 3q04. it takes time t2 (see fig for referecne). Find the raito t1/t2
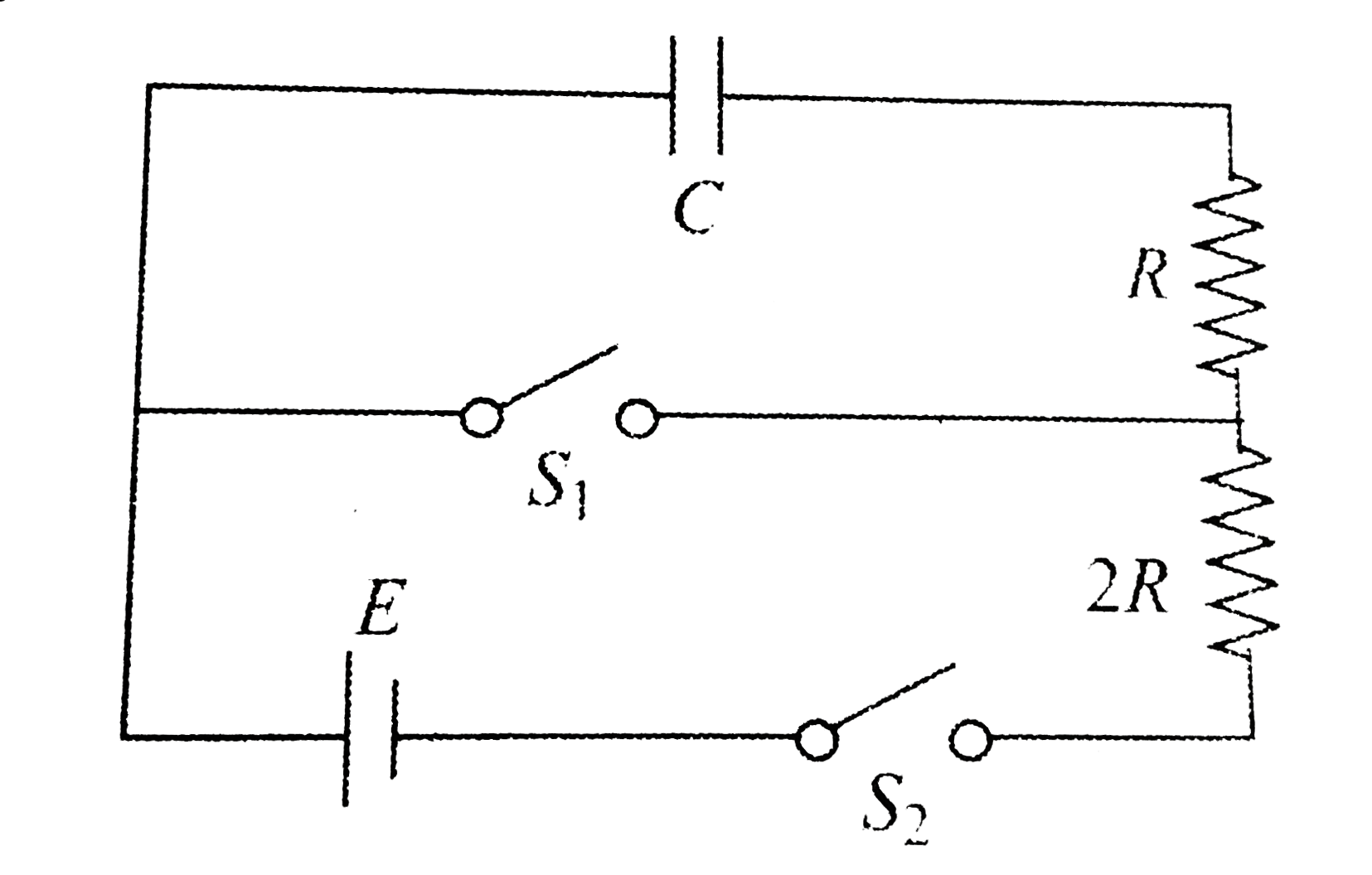
View SolutionIn the circuit shown in figure R1=R1=6R3=300MΩ,C=0.01μF and E−10V. The switch is closed at t=0, find
(a) Charge on capacitor as a function of time
(b) enegry of the capacitor at t=20s
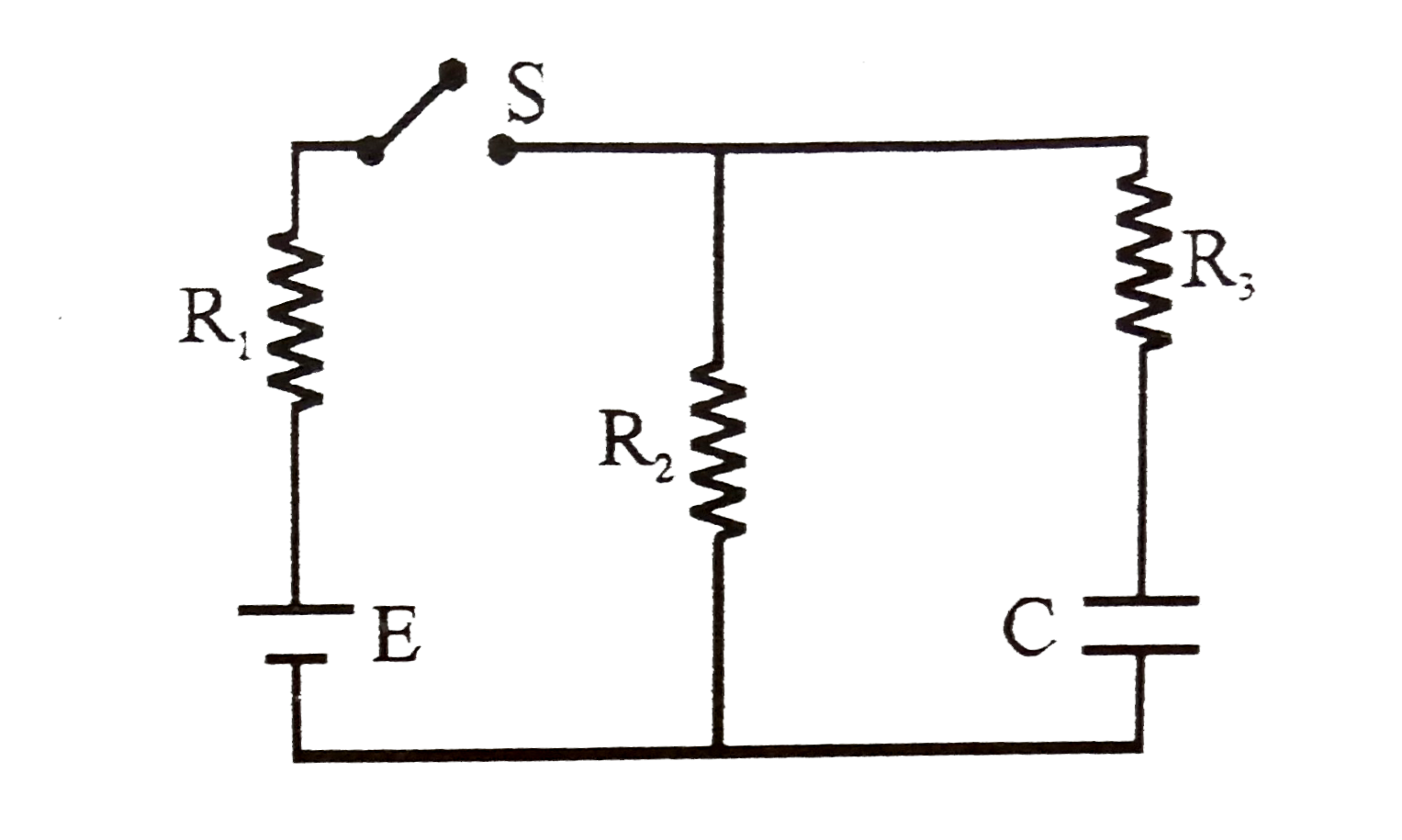
View SolutionIn the circuit shown in Figure, switch S1 is kept closed and S2 open for a long time. Resistance R1=1000R and R2=10−3R.
(a) Switch S1 is opened at time t = 0. Write the current and potential drop across R1 immediately after S1 is opened. What will be value of current after a long time?
(b) Switch S2 is closed (S1 is already closed since long) at time t = 0. Write current and potential drop across R2 immediately after this operation. Write current through the inductor as a function of time.
View SolutionFind the locus of the point (t2−t+1,t2+t+1),t∈R.
View Solution

_PHY_E06_157_Q01.png)