A cube has sides of length L=0.300m. It is placed with one corner at the origin ( refer to Fig.2.119).The electric field is not uniform but is given by →E=(−5.00NC−1)xˆi+(3.00NC−1)zˆk.
The surface that have zero flux are
S2,S4,andS5
S1,S3,S4,andS6
S1,S2,andS3
S2,S3,andS4
The correct Answer is:B
For S1,→A=L2(−ˆj),→E=−5xˆi+3zˆk.
Here →E⊥→A , so ϕs1=0.
For S2,→A=L2ˆk,→E=−5xˆi+3Lˆk.
Here −5xˆi is perpendicular to →A , so no flux due to this component.
ϕs2=(3Lˆk).L2ˆk=3L3=81×10−3Nm2C−1
For S3,→A=L2ˆj,→E=−5xˆi+3zˆk.
Here →E⊥→A , so ϕs3=0.
For S4,→A=−L2ˆk,→E=−5xˆi+0ˆk ("since" z = 0).<br>Herevec _|_ vec(A),sophi_(S_(4)) = 0.<br>ForS_(5) , vec(A) = L^(2) hat (i) , vec (E ) = - 5 L hat(i) + 3z hat(k).<br>Nofluxdue→3 z hat (k) compo≠nt.<br>phi_(S_(5)) = ( - 5 L hat (i)) . L^(2) hat(i) = - 5 L^(3) = - 135 xx 10^(-3) Nm^(2) C^(-1)<br>For S_(6), vec(A) = - L^(2) hat(i) , vec( E) = 0 hat (i) + 3 z hat(k)(sincex = 0).<br>Herevec(E ) _|_ vec(A),sophi_(s_(6)) = 0.<br>Totalfluxthroughthecubeisphi = phi (S_(1) + S_(2) + S_(3) + S_(5) + S_(6))<br>= 81 xx 10^(-3) - 135 xx 10^(-3) = - 54 xx 10^(-3) Nm^(2) C^(-1)<br>Now,phi = ( q_(in))/( epsilon_(0)) or q_(in) = phi epsilon_(0) = - 0.054 epsilon_(0) C
Topper's Solved these Questions
- ELECTRIC FLUX AND GAUSS LAWBOOK - CENGAGE PHYSICSCHAPTER - ELECTRIC FLUX AND GAUSS LAWEXERCISE - Subjective type
7 Videos
- ELECTRIC FLUX AND GAUSS LAWBOOK - CENGAGE PHYSICSCHAPTER - ELECTRIC FLUX AND GAUSS LAWEXERCISE - MCQ s
38 Videos
- ELECTRIC FLUX AND GAUSS LAWBOOK - CENGAGE PHYSICSCHAPTER - ELECTRIC FLUX AND GAUSS LAWEXERCISE - Multiple Correct
8 Videos
- ELECTRIC CURRENT AND CIRCUITBOOK - CENGAGE PHYSICSCHAPTER - ELECTRIC CURRENT AND CIRCUITEXERCISE - Interger
8 Videos
- ELECTRIC POTENTIALBOOK - CENGAGE PHYSICSCHAPTER - ELECTRIC POTENTIALEXERCISE - DPP 3.5
14 Videos
Similar Questions
A cube has sides of length L=0.2m. It is placed with one corner at the origin as shown in figure. The electric field is uniform and given by E=(2.5N/C)ˆi−(4.2N/C)ˆj. Find the electric flux through the entire cube.
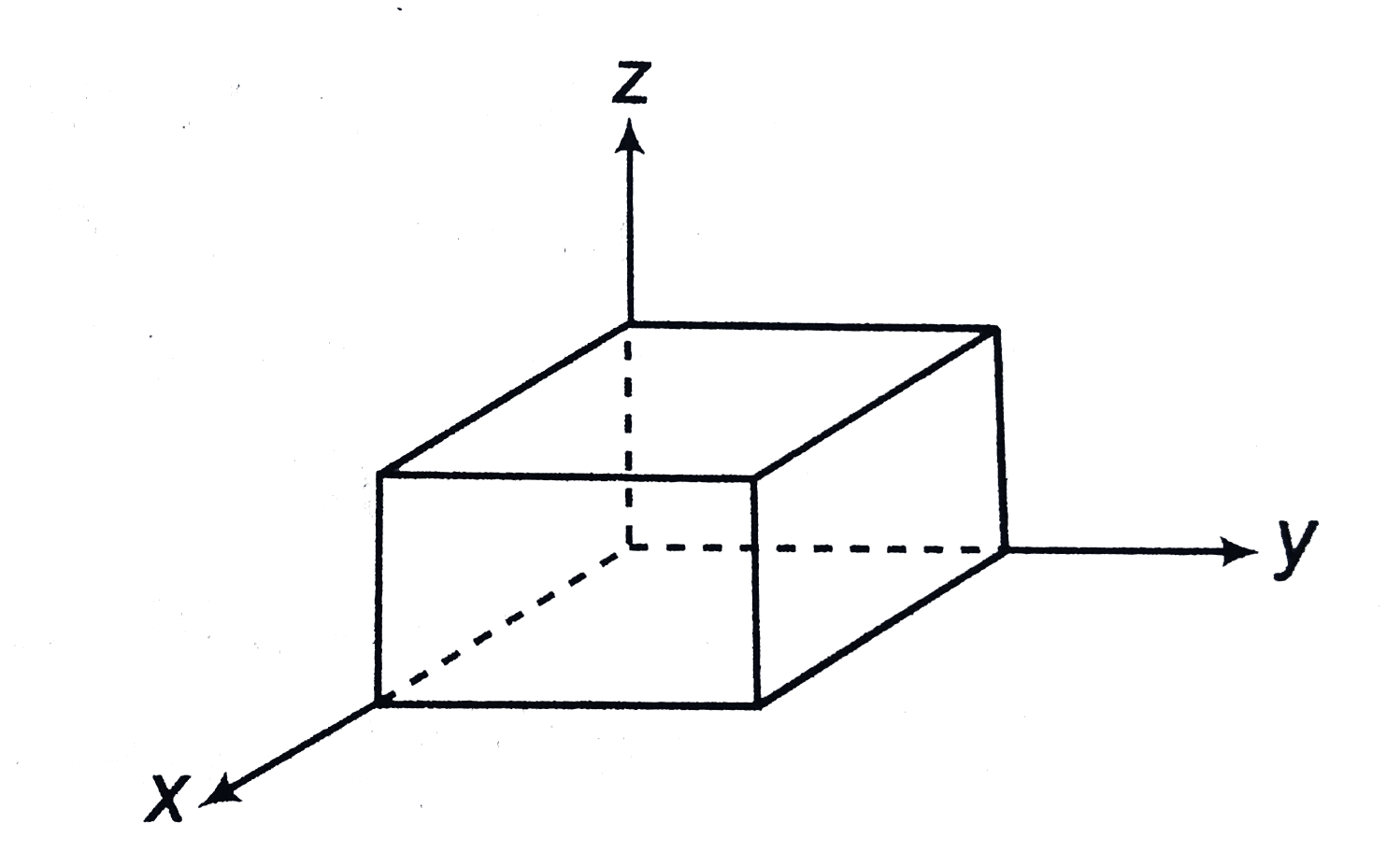
View SolutionA cube has sides of length L=0.2 m It is placed with one corner at the origin as shown in figure The electric field in uniform and given by →E=(2.5ˆi−4.2ˆj)N/C .Find the electric flux through the entire cube.

View Solution
Knowledge Check
A cube has sides of length L=0.300m. It is placed with one corner at the origin ( refer to Fig.2.119).The electric field is not uniform but is given by →E=(−5.00NC−1)xˆi+(3.00NC−1)zˆk.
The flux passing through the surface S5 will beA−0.135Nm2C−1B−0.054Nm2C−1C0.081Nm2C−1D0.054Nm2C−1A cube has sides of length L=0.300m. It is placed with one corner at the origin ( refer to Fig.2.119).The electric field is not uniform but is given by →E=(−5.00NC−1)xˆi+(3.00NC−1)zˆk.
The total flux passing through the cube isA−0.135Nm2C−1B−0.054Nm2C−1C0.081Nm2C−1DzeroA cube has sides of length L=0.300m. It is placed with one corner at the origin ( refer to Fig.2.119).The electric field is not uniform but is given by →E=(−5.00NC−1)xˆi+(3.00NC−1)zˆk.
The total electric charge inside the cube isA−0.054ε0CB0.081ε0CC0.135ε0CD0.054ε0C
If the electric field is given by →E=8ˆi+4ˆj+3ˆkNC−1, calculate the electric flux through a surface of area 100m2 lying in X-Y plane.
View SolutionA cube has sides of length L. It is placed with one corner at the origin ( refer to Fig.2.119). The electric field is uniform and given by →€=−Bˆi+Cˆj−Dˆk , where B,C,andD are positive constants.
The total flux passing through the cube is
View SolutionA cube has sides of length L. It is placed with one corner atAthe origin as shown in figure. The electric field is uniform and given by E=−Bˆi+Cˆj−Dˆk, where B, C and D are positive constants.
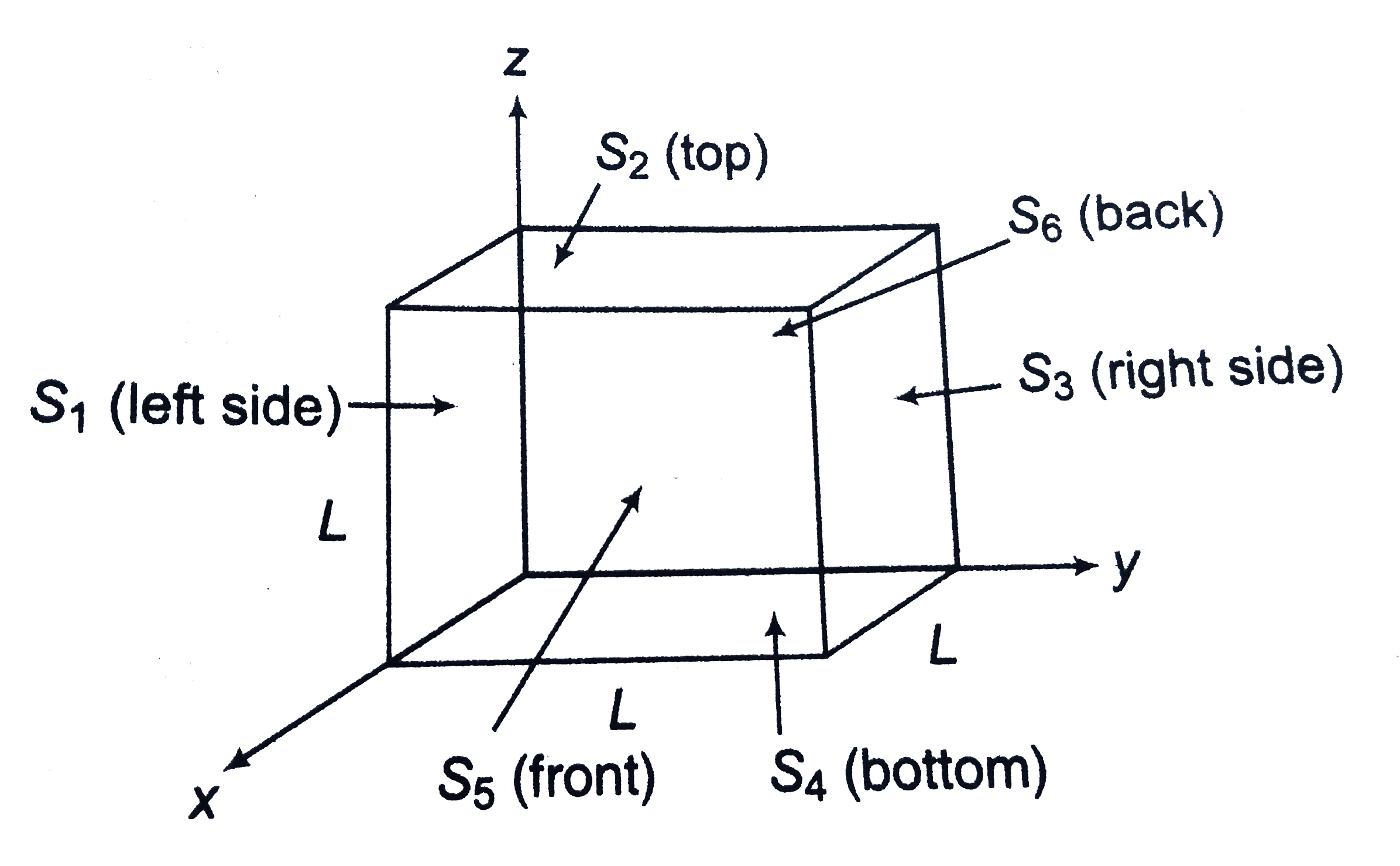
(a) Find the electric flux through each of the six cube faces S1,S2,S3,S4andS5andS6.
(b) Find the electric flux through the entire cube.
View SolutionThe cube shown in Fig. 2.119 has sides of length L=10.0cm . The electric field is uniform , has a magnitude E=4.00×103NC−1 and is parallel to the xy plane at an angle of 37∘ measured from the +x−aξs toward the +y−aξs .
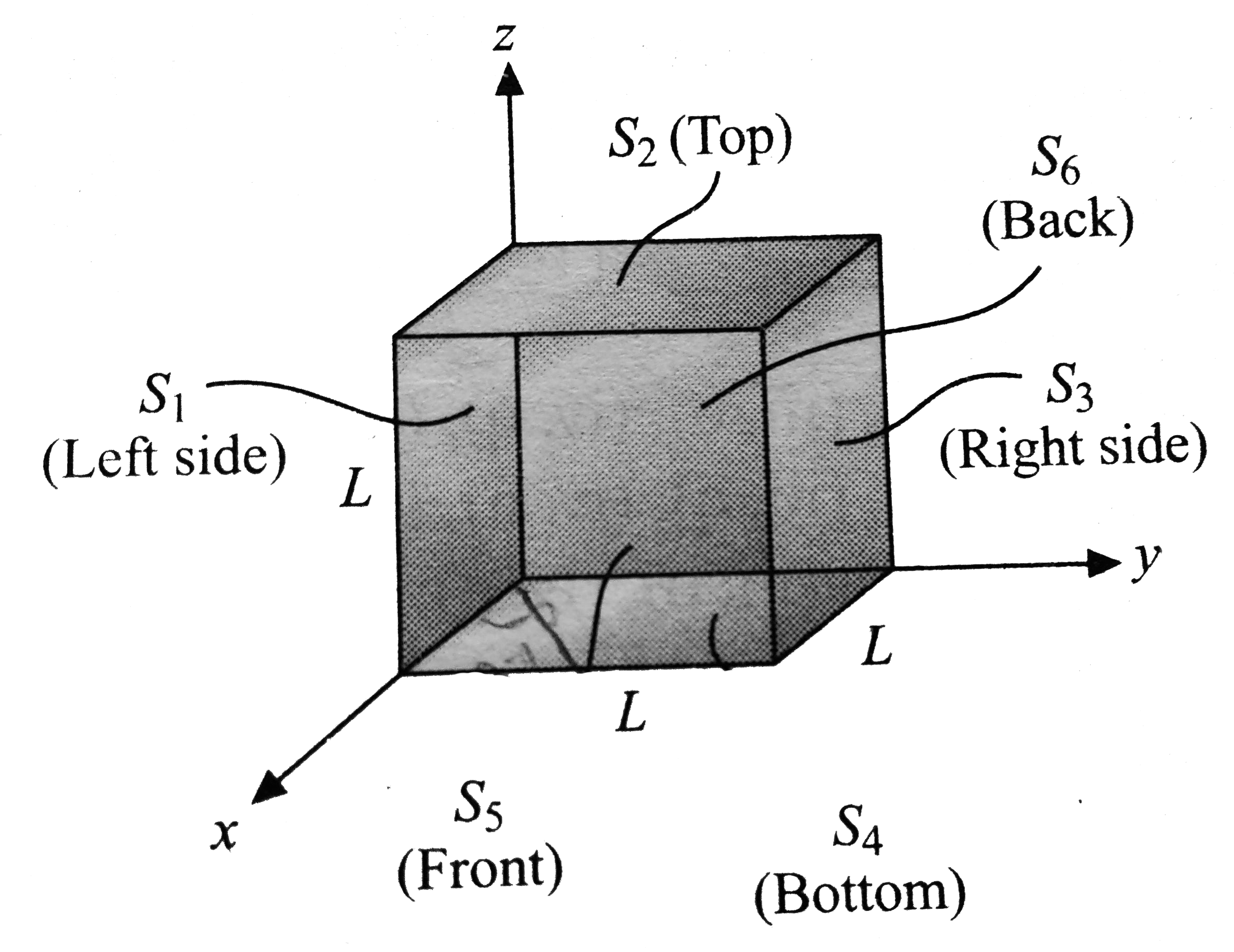
The surface that have zero flux are
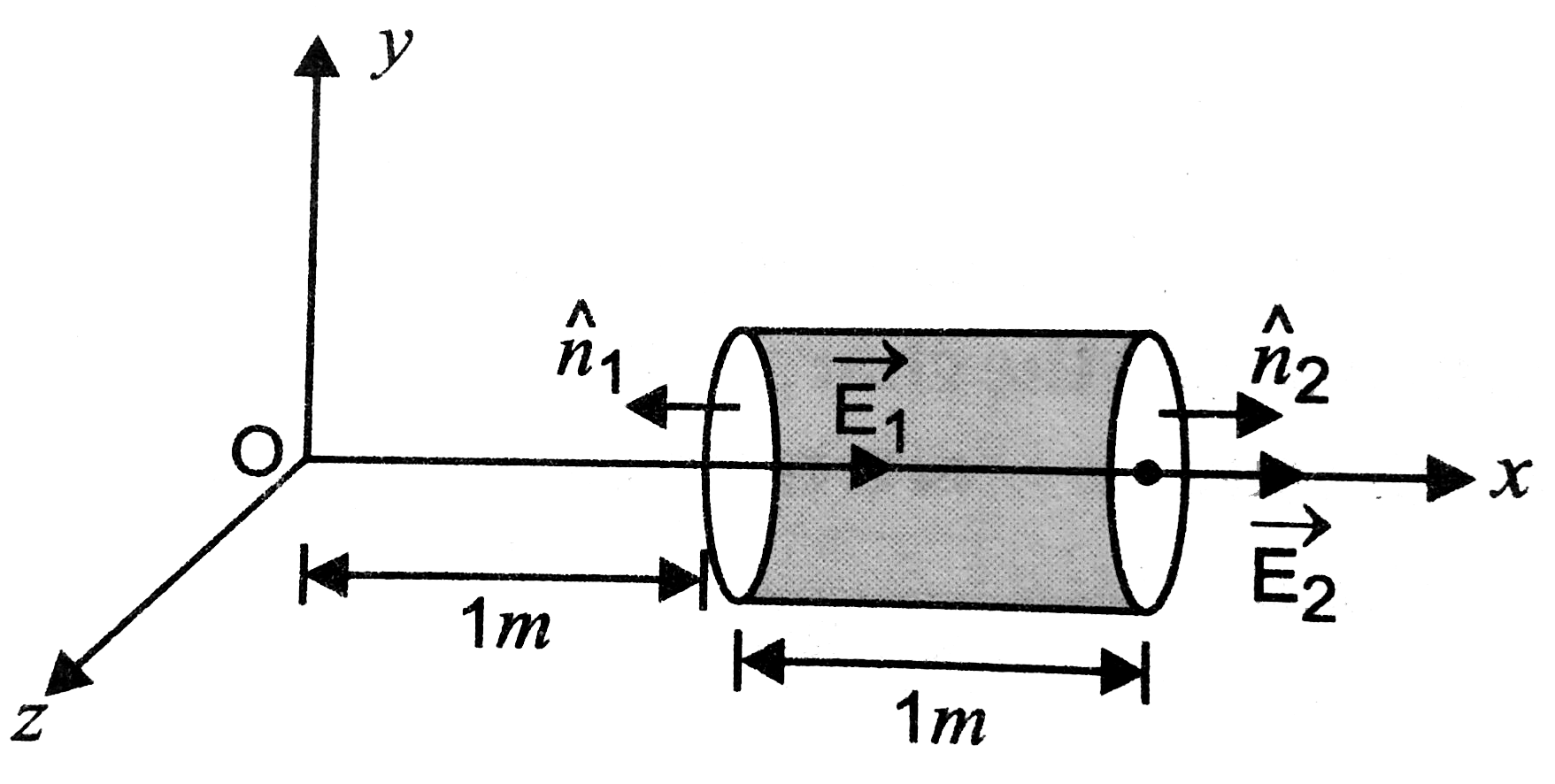
View SolutionA hollow cylindrical box of length 1m and area of cross section 25cm2 is placed in a three dimensional co-ordinate system as shown in Fig, The electric field in the
region is given by →E=50xˆi , where E is in NC−1 and x is in meter. Find
(i) Net flux through the cylinder
(ii) Charge enclosed by the cylinder.
View Solution
