Three elastic wires PQ,PR and PS support a body P of mass M, as shown in figure. The wires are of the some material and cross sectional area, the middle one being vertical. Find the loads by each wire.

Figure shows the situation wn the load (body P) desceneds due to the stretch in the wires. The new positions of wires PQ and PS are shown by the dotted lines. Let T1 and T2,T3 be teloads (tensions) carried by the three wires PQ,PR and PS, respectively, with the wires PQ and PS making angle θ with the vertical.

Considering the horizontal equilibrium of point P
T1sinθ=T3sinθ⇒T1=T3=T (say)
Considering vertical equilibrium of point P
T2+2Tcosθ=Mg..........i
If δ1 and δ2 be the elongations in the wires PR and PQ (or PS), respectively then
δl2=δl1cosθ (from geometry)
If A and Y be the cross sectional area and Young's modulus of each of the wires, then
TAY(Asecθ)=T2AYAcosθ⇒T=T2cos2θ........ii
Solving eqn i and ii T2=Mg1+2cos3θ
and T1=T3=T=Mgcos2θ1+2cos3θ
Topper's Solved these Questions
- PROPERTIES OF SOLIDS AND FLUIDSBOOK - CENGAGE PHYSICSCHAPTER - PROPERTIES OF SOLIDS AND FLUIDSEXERCISE - Solved Examples
11 Videos
- PROPERTIES OF SOLIDS AND FLUIDSBOOK - CENGAGE PHYSICSCHAPTER - PROPERTIES OF SOLIDS AND FLUIDSEXERCISE - Exercise 5.1
12 Videos
- NEWTON'S LAWS OF MOTION 2BOOK - CENGAGE PHYSICSCHAPTER - NEWTON'S LAWS OF MOTION 2EXERCISE - Integer type
1 Videos
- RIGID BODY DYNAMICS 1BOOK - CENGAGE PHYSICSCHAPTER - RIGID BODY DYNAMICS 1EXERCISE - Integer
11 Videos
Similar Questions
Knowledge Check
Three vertical wires, I,II and III are supporting a block of mass M in horizontal positon. The wires are to equal length and cross-sectional area. It is given that Young's modulus of wire II,Y2=Y3 (Yong's modulus of wire III). The wire I and III and attached at extreme ends of the block.
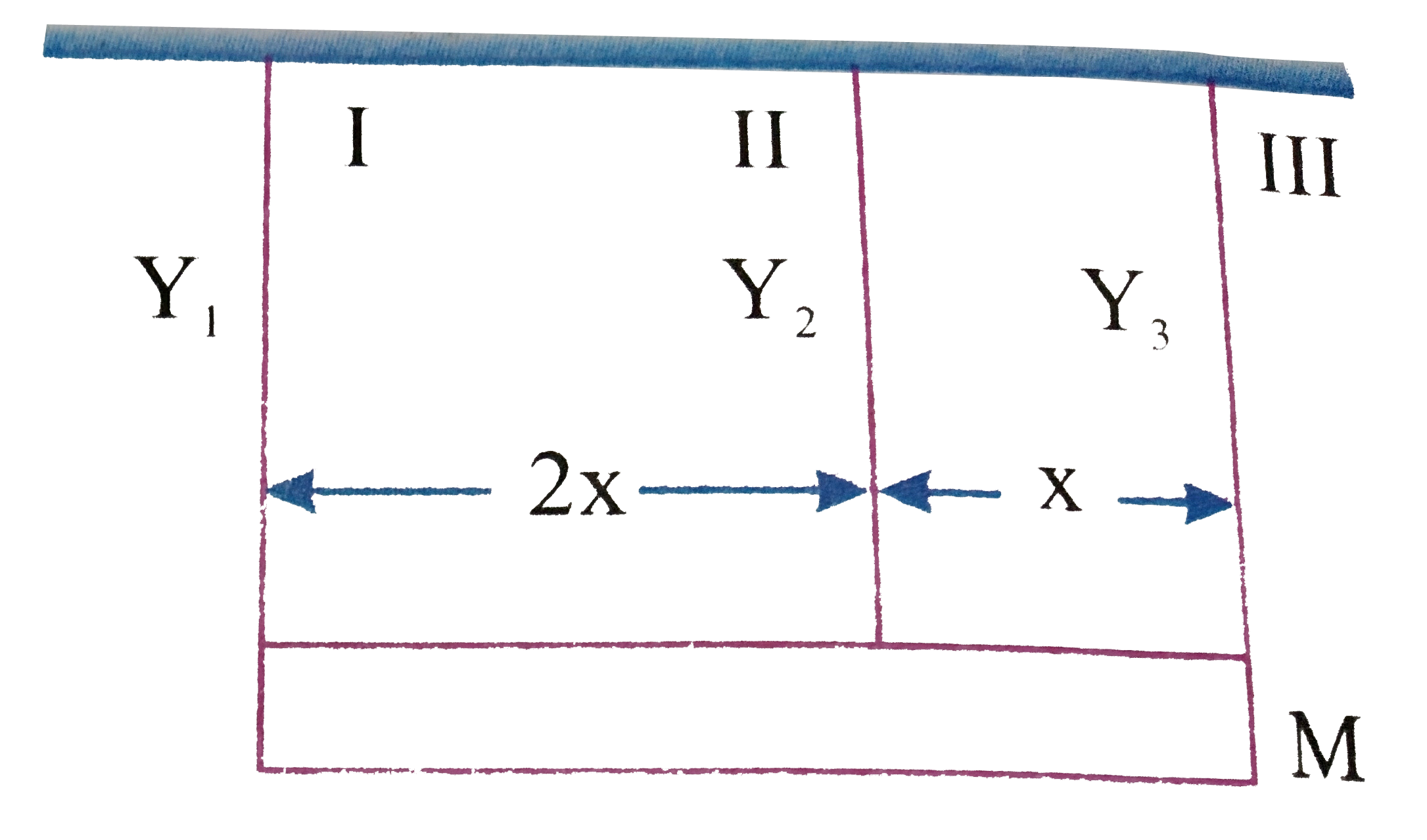 AT1=2T3B2T1=T3CT2=T3DT1=Mg/5
AT1=2T3B2T1=T3CT2=T3DT1=Mg/5A load is supported using three wires of same cross section area as shown. Then fox
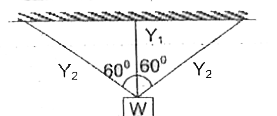
 APQRS2314BPQRS1423CPQRS3241DPQRS2234
APQRS2314BPQRS1423CPQRS3241DPQRS2234A block of mass M is suspended from a wire of length L, area of cross-section A and Young's modulus Y. The elastic potential energy stored in the wire is
A12M2g2LAYB12MgALYC12M2g2AYLD12MgYAL
A wire of length 10 cm and area of cross section 1mm2 has a resistance of 5Ω. Find the resistivity of the material of the wire.
View SolutionA bar of mass m and length l is hanging from point A as shown in the figure . If the Young's modulus of elasticity of the bar is Y and area of cross - section of the wire is A, then the increase in its length due to its own weight will be

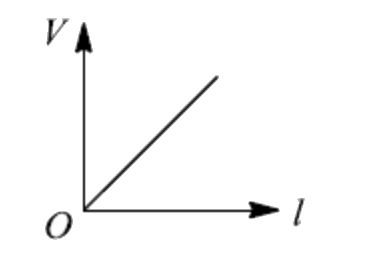
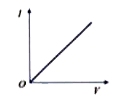
View Solutionl-V characteristic of a copper wire of length Land area of cross-section A is shown in figure. The slope of the curve becomes
View SolutionA metal wire of length L1 and area of cross section A is ttached to a rigid support. Another metal wire of length L2 and of the same cross sectional area is attached to the free end of the first wire. A body of mass M is then suspended from the free end of the second wire, if Y1 and Y2 are the Young's moduli of the wires respectively the effective force constant of the system of two wires is
View SolutionFind the resistance (in mega Ω) of a wire of length 10 m, cross sectional area 1cm2 and made of a material of resistivity 100 Ωm.
View Solution