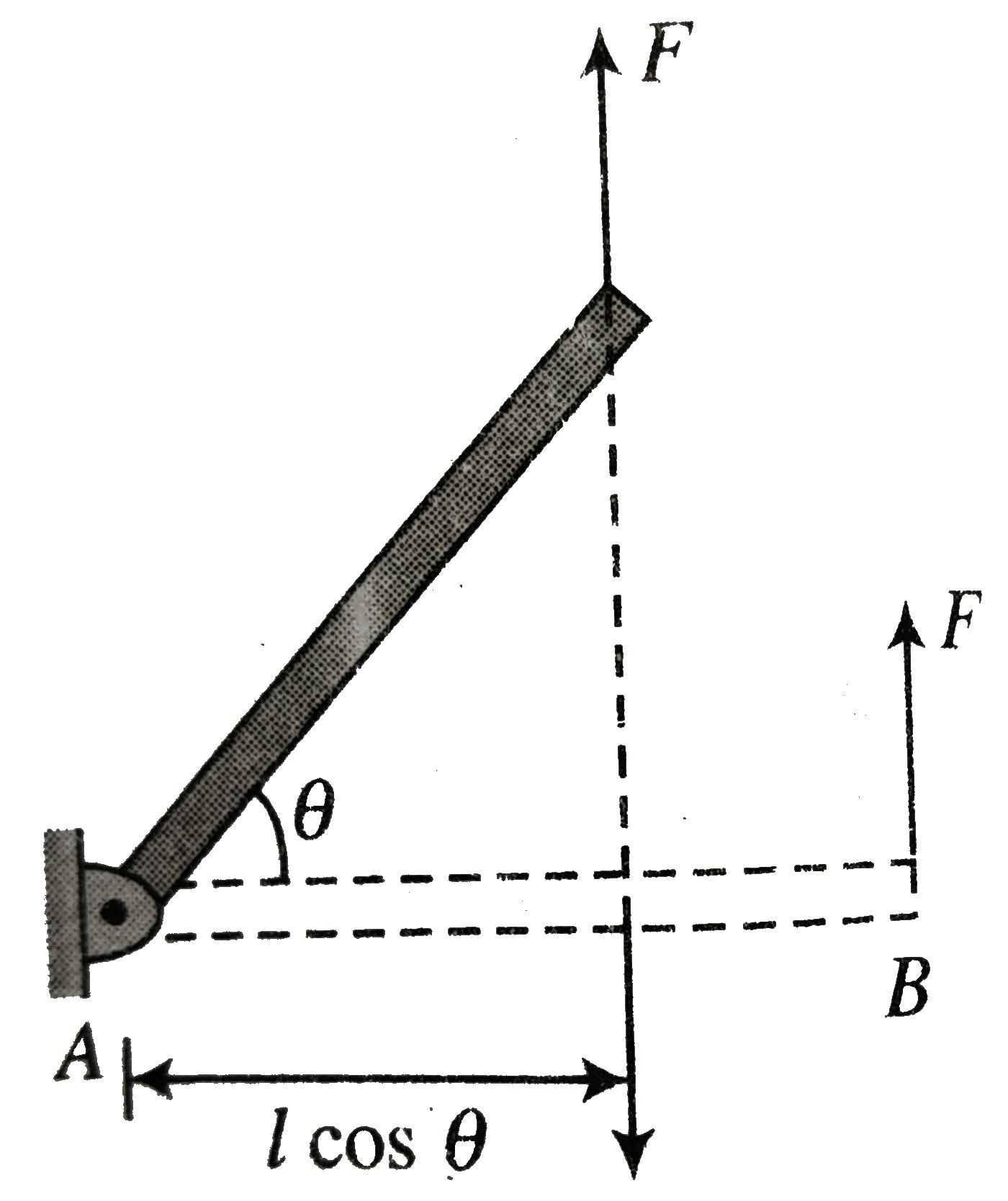
A thin horizontal uniform rod $A B$ of mass $m$ and length $l$ can rotate freely about a vertical axis passing through its end $A$ . At a certain moment, the end $B$ starts experiencing a constant force $F$ which is always perpendicular to the original position of the stationary rod and directed in a horizontal plane. The angular velocity of the rod as a function of its rotation angle $θ$ measured relative to the initial position should be.

Video Solution

Text Solution

Verified by Experts

Work done by the torque
$△ W = \int τ d t h η = \int_{0}^{θ} F l cos θ d θ$
$△ W = F l sin θ$
Now using work energy theorem
$△ W = △ k$
$∴ F l sin θ = [\frac{1}{2} (\frac{m l^{2}}{3}) ω^{2} - 0]$
Which gives $ω = (\frac{\sqrt{6 F sin θ}}{m l})$

Updated on:21/07/2023

A uniform rod of mass $m$ and length $l$ rotates in a horizontal plane with an angular velocity $ω$ about a vertical axis passing through one end. The tension in the rod at a distance x from the axis is
A $\frac{1}{2} m ω^{2} x$
B $\frac{1}{2} m ω^{2} (1 - \frac{x^{2}}{l})$
C $\frac{1}{2} m ω^{2} l (1 - \frac{x^{2}}{l^{2}})$
D $\frac{1}{2} m ω^{2} l [1 - \frac{x}{l}]$
A uniform metal rod is rotated in horizontal plane about a vertical axis passing through its end at uniform rate. The tension in the rod is
Asame at all points
Bdifferent at differen points and maximum at centre of rod
Cdifferent at different points and minimum at axis of rotation.
Ddifferent at different points and maximum at axis of rotation
A thin uniform rod of length $l$ and mass $m$ is swinging freely about a horizontal axis passing through its end. Its maximum angular speed is $ω$ . Its centre of mass rises to a maximum height of -
A $\frac{1}{3} \frac{l^{2} ω^{2}}{g}$
B $\frac{1}{6} \frac{l ω}{g}$
C $\frac{1}{2} \frac{l^{2} ω^{2}}{g}$
D $\frac{1}{6} \frac{l^{2} ω^{2}}{g}$