Two capacitors of 10pF and 20pF are connected to 200 V and 100 V sources, respectively. If they are connected by the wire, then what is the common potential of the capacitors?
133.3 V
150 V
300 V
400 V
The correct Answer is:A
Given, C1=10pF=10×10−12F,
C2=20pF=20×10−12F,
V1=200VandV2=100V
where, C1= Capacitance of Ist capacitor
C2= Capacitance of Iind capacitor
V1= Voltage across Ist capacitor
V2= Voltage across IInd capacitor
We know that, V1=q1C1andV2=q2C2
⇒ q1=V1C1andq2=V2C2
So, common potential of capacitors
V=q1+q2C1+C2=V1C1+V2C2C1+C2
=200×10×10−12+100×20×10−1210×10−12+20×10−12
=200×10+100×2010+20
=2000+200030
=400030=133.3V
Topper's Solved these Questions
- ELECTROSTATICSBOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - ELECTROSTATICSEXERCISE - MHT CET Corner
29 Videos
- ELECTROSTATICSBOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - ELECTROSTATICSEXERCISE - PRACTICE EXERCISE (Exercise 1 (TOPICAL PROBLEMS))
87 Videos
- ELECTRONS AND PROTONSBOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - ELECTRONS AND PROTONSEXERCISE - MHT CET CORNER
19 Videos
- FORCEBOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - FORCEEXERCISE - Exercise 2 (Miscellaneous Problems)
19 Videos
Similar Questions
Two capacitors of capacitance 10 μF and 20 μF are charged to potential 20 V and 10 V respectively. If they are connected to share charges, then loss of energy is
View SolutionTwo parallel plate capacitors of capacitances 1 pF and 2 pF are connected to the batteries of 100 V and 150 V as shown in the figure (r ). Calculate the potential difference across each capacitor.
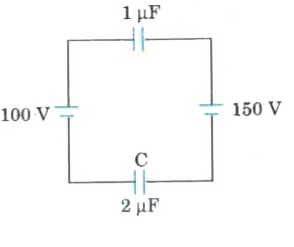
View Solution
Knowledge Check
Two capacitors of 10pF and 20pF are connected to 200 V and 100 V sources, respectively. If they are connected by the wire, then what is the common potential of the capacitors?
A133.3VB150VC300VD400VTwo capacitors of 2μF and 3μF are charged to 150V and 120V, respectively. The plates of capacitor are connected as shown in the figure. An uncharged capacitor of capacity 1.5μF falls to the free end of the wire. Then
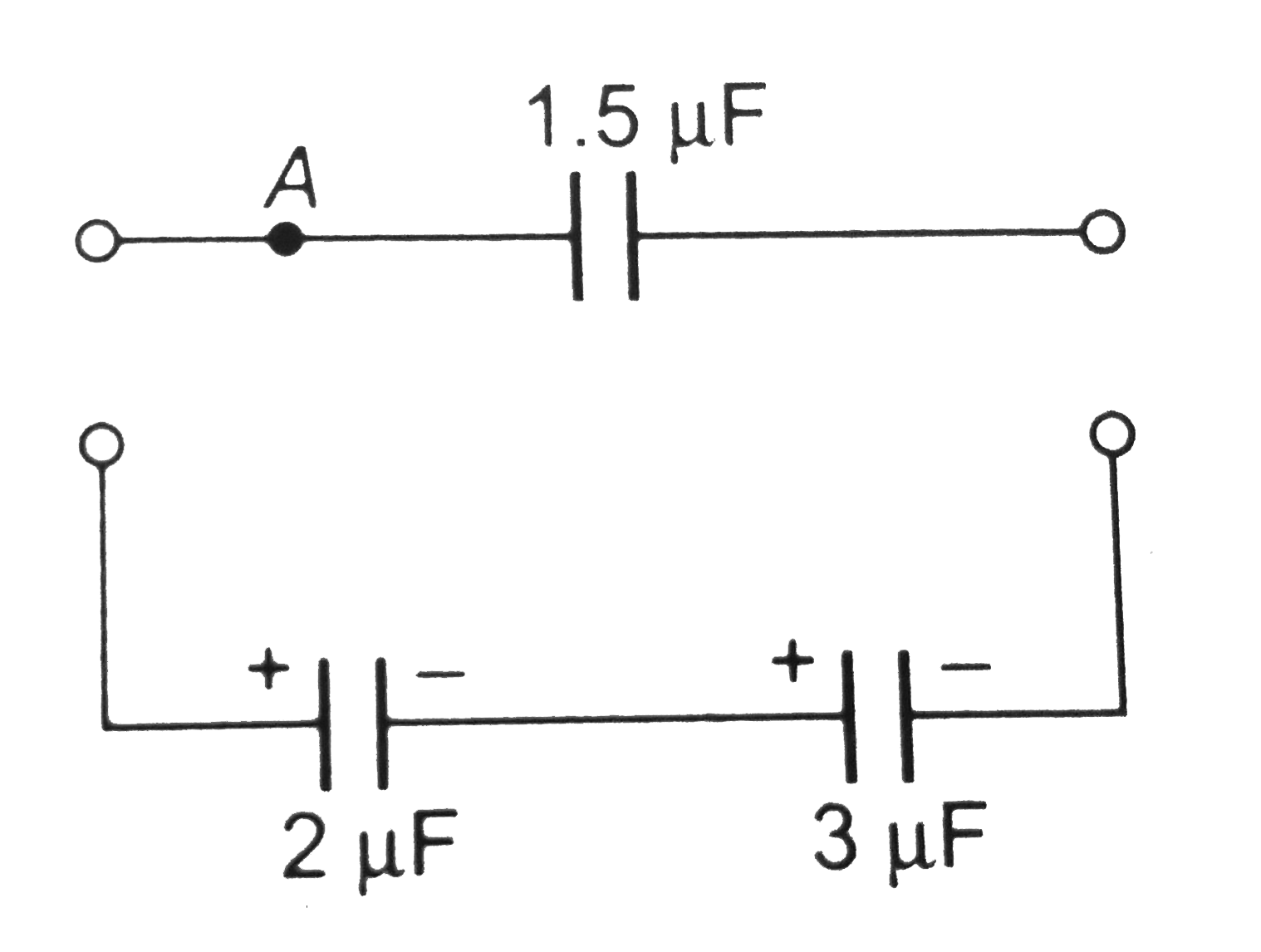 Achargeg on 1.5μF capacitor is 180μFBcharge on 2μFcapacitor is 120μFCpositive chasrge flows through A from right to leftDpositive charge flows through A from left to right
Achargeg on 1.5μF capacitor is 180μFBcharge on 2μFcapacitor is 120μFCpositive chasrge flows through A from right to leftDpositive charge flows through A from left to rightTwo capacitors of 10μF and 20μF are connected in series with a 30V battery. The charge on the capacitors will be, respectively
A100μC,200μCB200μC,100μCC100μC,100μCD200μC,200μC
Two capacitors of capacitances 3μFand6μF, are charged to potentials 2V and 5V respectively. These two charged capacitors are connected in series. Find the potential across each of the two capacitors now.
View SolutionA 5μF capacitor is connected to a 200 V, 100 Hz ac source. The capacitive reactance is
View SolutionThree capacitors of 2.0,3.0 and 6.0μF are connected in series to a 10V source. The charge on the 3.0μF capacitor is
View SolutionTwo capacitor of capacitance 5μF and 10μF are charged to potential 40V and 10V respectively. Now the two capacitors ate connected with plates of same polarities together .
(A) the common potential will be 20V
(B) the final charges of capacitors will be 100μC and 200μC
(c) the ratio of total energy stored in capacitors before and after connection is 3:2
(D) the loss energy of system is 1500μJ
View SolutionTwo capacitors of capacitance 2μFand5μF are charged to a potential difference 100V and 50 V respectively and connected such that the positive plate of one capacitor is connected to the negative plate of the other capacitor after the switch is closed, the initial current in the circuit is 50 mA. the total resistance of the connecting wires is (in Ohm):
View Solution
