Given R1=5.0±0.2Ω,andR2=10.0±0.1Ω. What is the total resistance in parallel with possible % error?
To find the total resistance in parallel for the given resistances R1 and R2 along with the possible percentage error, we can follow these steps:
Step 1: Identify the values of the resistances and their uncertainties
- Given:
- R1=5.0Ω with an uncertainty of ΔR1=0.2Ω
- R2=10.0Ω with an uncertainty of ΔR2=0.1Ω
Step 2: Calculate the total resistance in parallel
The formula for total resistance RP when two resistors are in parallel is given by:
1RP=1R1+1R2
Substituting the values:
1RP=15.0+110.0
Calculating the right-hand side:
1RP=0.2+0.1=0.3
Now, taking the reciprocal to find RP:
RP=10.3≈3.33Ω
Step 3: Calculate the percentage error
To find the percentage error in RP, we can use the formula for propagation of uncertainty in parallel resistances:
ΔRPRP×100=ΔR1R1×100+ΔR2R2×100+ΔR1+ΔR2R1+R2×100
Substituting the values:
1. Calculate each term:
- For R1:
ΔR1R1×100=0.25.0×100=4%
- For R2:
ΔR2R2×100=0.110.0×100=1%
- For the total resistance:
ΔR1+ΔR2R1+R2×100=0.2+0.15.0+10.0×100=0.315.0×100=2%
2. Add these contributions together:
Total Percentage Error=4%+1%+2%=7%
Step 4: State the final result
The total resistance in parallel with the possible percentage error is:
RP=3.33Ω±7%
- Given:
- R1=5.0Ω with an uncertainty of ΔR1=0.2Ω
- R2=10.0Ω with an uncertainty of ΔR2=0.1Ω
The formula for total resistance RP when two resistors are in parallel is given by:
To find the percentage error in RP, we can use the formula for propagation of uncertainty in parallel resistances:
- For R1:
ΔR1R1×100=0.25.0×100=4%
- For R2:
ΔR2R2×100=0.110.0×100=1%
- For the total resistance:
ΔR1+ΔR2R1+R2×100=0.2+0.15.0+10.0×100=0.315.0×100=2%
Total Percentage Error=4%+1%+2%=7%
The total resistance in parallel with the possible percentage error is:
Topper's Solved these Questions
- DIMENSIONS & MEASUREMENTBOOK - CENGAGE PHYSICSCHAPTER - DIMENSIONS & MEASUREMENTEXERCISE - Subjective
31 Videos
- DIMENSIONS & MEASUREMENTBOOK - CENGAGE PHYSICSCHAPTER - DIMENSIONS & MEASUREMENTEXERCISE - Single Correct
93 Videos
- DIMENSIONS & MEASUREMENTBOOK - CENGAGE PHYSICSCHAPTER - DIMENSIONS & MEASUREMENTEXERCISE - Exercise 1.2
6 Videos
- CENTRE OF MASSBOOK - CENGAGE PHYSICSCHAPTER - CENTRE OF MASSEXERCISE - INTEGER_TYPE
1 Videos
- FLUID MECHANICSBOOK - CENGAGE PHYSICSCHAPTER - FLUID MECHANICSEXERCISE - INTEGER_TYPE
1 Videos
Similar Questions
108 . If two resistors of resistances R1=(4±0.5)ΩandR2=(16±0.5)Ω are connected (i) in series and (ii) in parallel, find the equivalent resistance in each case with limits of percentage error.
View Solution1resistances R_(1)=(3.0+-0.1)Omega and R_(2)=(6.0+-0.2)Omega are to be joined together. (A) The maximum resistance obtainable is (9.0+-0.3)Omega (B) The maximum resistance obtainable is (9.0+-0.2)Omega (C) The minimum resistance obtainable is (2.0+-0.3)Omega (D) The minimum resistance obtainable is (2.0+-0.2)Omega
View Solution
Knowledge Check
Given Resistance R1=(8±0.4)Ωand Resistence, R2=(8±0.6)Ω What is the net resistence when R1 and R2 are connected in series?
A(16±0.4Ω)B(3.45±0.3)ΩC(3.45±0.4)ΩD(3.45±0.5)ΩTwo resistor R1=(24±0.5)ΩandR2=(8±0.3)Ωare joined in series, The equivalent resistance is
A32±0.33ΩB32±0.8ΩC32±0.2ΩD32±0.5ΩTwo carbon resistances are given by R1=(4±0.4) ohm and R2=(12±0.6)Ω. What is their net resistance with percentage error, if they are connected in series?
A16Ω±5%B16Ω±6.25%C16Ω±8%D16Ω±12%
In the circuit shown in Fig. the emf of the sources is equal to ξ=5.0V and the resistances are equal to R1=4.0Ω and R2=6.0Ω. The internal resistance of the source equals R=1.10Ω. Find the currents flowing through the resistances R1 and R2.
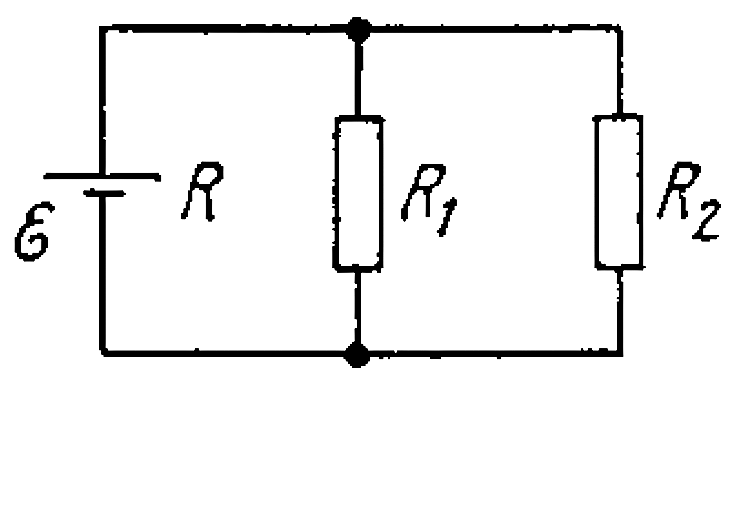
View SolutionThree cells of emf, ε1=1.5V,ε2=2.0Vandε3=3.0V having internal resistance r1=0.30Ω,r2=0.4Ωandr3=0.6Ω respectively are connected in parallel. Find out the equivalent emf and the equivalent resistance of a cell which can replace this combination.
View SolutionTwo resistors R1=(4±0.8)ΩandR2(4±0.4)Ω are connected in parallel. The equivalent resistance of their parallel combination will be :
View SolutionTwo resistor of resistance R1=(6±0.09)Ω and R2=(3±0.09)Ω are connected in parallel the equivalent resistance R with error (in Ω)
View SolutionThe values of two resistors are R1=(6±0.3)kΩ and R2=(10±0.2)kΩ. The percentage error in the equivalent resistance when they are connected in parallel is
View Solution
