In the adjoining figure AD and BE are the perpendiculars on side BC and CA respectively of the ΔABC , Then , A, B, D, E are concyclic. Solution in Bengali
The correct Answer is:1
since the angles ∠ADBand∠AEB on the same side of AB are equal for being right angles each.
Hence the four ponits A,B, D, E are concyclic , by theorem-36

Topper's Solved these Questions
- THEOREMS RELATED TO CYCLIC QUADRILATERAL BOOK - CALCUTTA BOOK HOUSECHAPTER - THEOREMS RELATED TO CYCLIC QUADRILATERAL EXERCISE - Fill in the blanks:
6 Videos
- THEOREMS RELATED TO CYCLIC QUADRILATERAL BOOK - CALCUTTA BOOK HOUSECHAPTER - THEOREMS RELATED TO CYCLIC QUADRILATERAL EXERCISE - EXAMPLE (Short - answer type questions)
5 Videos
- THEOREMS RELATED TO CYCLIC QUADRILATERAL BOOK - CALCUTTA BOOK HOUSECHAPTER - THEOREMS RELATED TO CYCLIC QUADRILATERAL EXERCISE - Long -answer type questions (L.A.)
12 Videos
- THEOREMS RELATED TO CIRCLE BOOK - CALCUTTA BOOK HOUSECHAPTER - THEOREMS RELATED TO CIRCLE EXERCISE - EXERCISE - 1 (Long - answer type question )
9 Videos
- THEOREMS RELATED TO ANGLES IN A CIRCLEBOOK - CALCUTTA BOOK HOUSECHAPTER - THEOREMS RELATED TO ANGLES IN A CIRCLEEXERCISE - EXERCISE 2.3 (Long Answer Type Questions)
14 Videos
Similar Questions
All angles in the same segment are……….
View SolutionIn the adjoining figure , if ∠BAD=60∘.∠ABC=80∘ , then find the values of ∠DPCand∠BQC .
View SolutionIn a cyclic quadrilateral, the ratio of three consecutive angle is 1 : 2 : 3 Find the value of first and third angles.
View SolutionProve that diameter is the greatest chord of a circle.
View SolutionProve that angle in the segment of a circle which is greater than a semi-circle is an acute angle.
View SolutionIn the adjoining figure D, E and F are the mid-points of the sides BC, CA and AB of the equilateral ΔABC. Prove that ΔDEF is also an equilateral triangle.

View SolutionIn the adjoining figure, ΔPQR is formed by the sides PQ, QR and RP which are drawn parallel to sides AB,BC and CA respectively of ΔABC. Prove that
PQ+QR+RP+2(AB+BC+CA).

View SolutionIn the adjoining figure AD and BE are perpendicular ot BC and AC respectively of the ΔABC. The four points A,B,D,E are concylic.
View SolutionIn the adjoining figure, in ΔABC, point D is on side BC such that, ∠BAC=∠ADC. Prove that, CA2=CB×CD.
View Solutionयदि ΔABCकी भुजाओं BC,CA और AB के मध्यबिंदु क्रमशः D,E और F हों तो सिद्ध कीजिए कि AD रेखाखंड EF को समद्विभाजित करती है।
View SolutionIn ΔABC the midpoints are D,E and F of the sides AB,BC and CA, then ΔDEF:ΔABC is
View SolutionD(2,1,0),E(2,0,0),F(0,1,0) are midpoints of the sides BC,CA,AB of ΔABC respectively . The , the centroid of ΔABc is
View SolutionIn ΔABC if D and E are the mid-points of the sides BC and CA respectively then 2(AD+EB)=
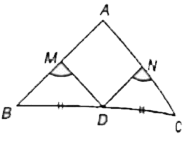
View SolutionIn the adjoining figure D is the midpoint of BC of a ΔABC,DMandDN are the perpendiculars on AB and AC respectively and DM=DN, then the ΔABC is :
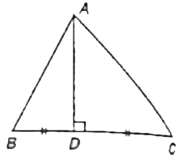
View SolutionIn the adjoning figure of ΔABC,AD is the perpendicular bisector of side BC. The triangle ABC is :
View Solution
