A locomotive engine without wagons can go 24 miles an hour and its speed is diminished as the square root of the number of wagons attached with 4 wagons its speed is 20 miles an hour. Find the maximum number of wagons with which the engine can move. Solution in Bengali
Let the speed diminished by m mile / hour and the number of wagons =n .
As per question , m ∝√nor,m=k√n………(1) (when k ≠ 0= variation constant)
Now , if 4 wagons be attached with the engine, its speed diminishes by
(24-20) mile/hour =4 mile /hour.
∴ from (1) we get , 4=k.√4or,2k=4or,k=2
∴ (1) cecomes , m 2√n.....(2)
Putting m =24 in (2) we get , 24 =2√n
⇒√n=12⇒n=(12)2=144.
therefore the engine will be speedless if 144 wagons are attached with it.
Hence the required maximum number of wagons =144-p1=143 .
Topper's Solved these Questions
Similar Questions
If a ∝1b, then
View SolutionIf A, B, C are the angles of a triangle then tan(B+C2)=
View SolutionA loaded 20,000kg coal wagon is moving on a level track at 6ms^-1 . Suddenly 5000kg of coal is dropped out of the wagon. The final speed of the wagon is
View SolutionA locomotive (engine) pulls a series of wagons. Which is the correct analysis of the situation?
View SolutionA locomotive engine without wagons can go 35 km per hour and its speed is diaminished by a quantity which varies as the square root of the number of wagons attached , with 9 wagons its speed is 20 km per hour . Find the least number of wagons which the engine can move.
View SolutionA 1000 kg engine pulls a train of 4 wagons each of 2500 kg along a horizontal railway track . If the engine exerts a force of 50000 N on wagons and track offers force of friction 10000 N, then calculate
(i) The net accelerating force
(ii) The acceleration of the train
(iii) The force of wagon -1 on wagon - 2
View SolutionThree wagons, each of mass m are being pulled by an engine exerting a froce F on the lead wagon. What is the fore exerted on the last wagon by the middle wagon?
View SolutionFour identical railway wagons each of m, are coupled together and rest on a smooth horizontal track. A fifth wagon of mass 2m and movingk at 5m/s collides and couple with the statioinary wagons. What is the speed of the wagons after the impact?
View SolutionThe reduction is speed, r in miles per hour of an enginge having n bogies attached to it is given as : r=4√n. The final speed, s, in miles per hour , at which the engine can pull the n bogies attached is give as : s=40−r . What is the maximum number of bogies that the engine call pull ?
View SolutionFind velocity of wagon as function of time when it is being filled with stationary hopper at the rate μ kg/sec. and constant force F is applied horizontally on the wagon as shown in figure.
M0 is the mass of the wagon and initial speed of the wagon u = 0 at time t = 0.
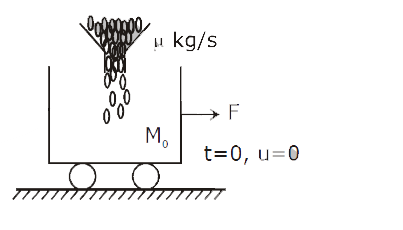
View SolutionA 1000 kg engine pulls a train of 4 wagons each of 2500 kg along a horizontal railway track . If the engine exerts a force of 50000 N on wagons and track offers force of friction 10000 N, then calculate
(i) The net accelerating force
(ii) The acceleration of the train
(iii) The force of wagon -1 on wagon - 2
View SolutionAn engine can move at the speed of 20/3 m/s without any wagon attached. Reduction in the speed of the train is directly proportional to the square root of the no. of wagons attached . When there are only 4 engines attached its speed is (50/9) m/s. The greatest no. of wagons with which the engine can move is :
View Solutionমালবােঝাই করা ভারী মালগাড়ির চালক মালগাড়িকে গতিশীল করার জন্য প্রথমে সামান্য পিছনদিকে চালিয়ে পরে সামনের দিকে চালায় কেন ?
View Solutionएक 2 किग्रा. द्रव्यमान के पिण्ड की चाल 10 सेकण्ड में 4 मी./से. से 10 मी./से. हो जाती है। बल का परिमाण व दिशा ज्ञात कीजिए।
View Solutionएक वाष्पचलित इंजन रेल के डिब्बों के बगैर 24 किमी. / घंटा की चाल से चलता है। इंजन की गति में कमी साथ में लगाए गए डिब्बों की संख्या क्र वर्गमूल के समानुपाती है। 4 डिब्बों के साथ उसकी गति 20 किमी. / घंटा हो जाती है। डिब्बों की अधिकतम संख्या ज्ञात कीजिए जिसे इंजन खींच सकता है ?
View Solution
