Question

Two vectors $\overrightarrow{A}\text{and}\overrightarrow{B}$ inclined at an angle $\theta$ have a resultant $\overrightarrow{R}$ which makes an angle $\alpha$ with $\overrightarrow{A}$ and angle $\beta$ with $\overrightarrow{B}$. Let the magnitudes of the vectors $\overrightarrow{A},\overrightarrow{B}\text{and}\overrightarrow{R}$ be represented by A, B and R respectively. Which of the following relations is not correct ?

Options

A. `(R)/(sin(alpha+beta))=(A)/(sin alpha) = (B)/(sin beta)`
B. `R sin alpha = B sin (alpha + beta)`
C. `A sin alpha = B sin beta`
D. `R sin beta = A sin (alpha + beta)`

Answer

A

Explanation

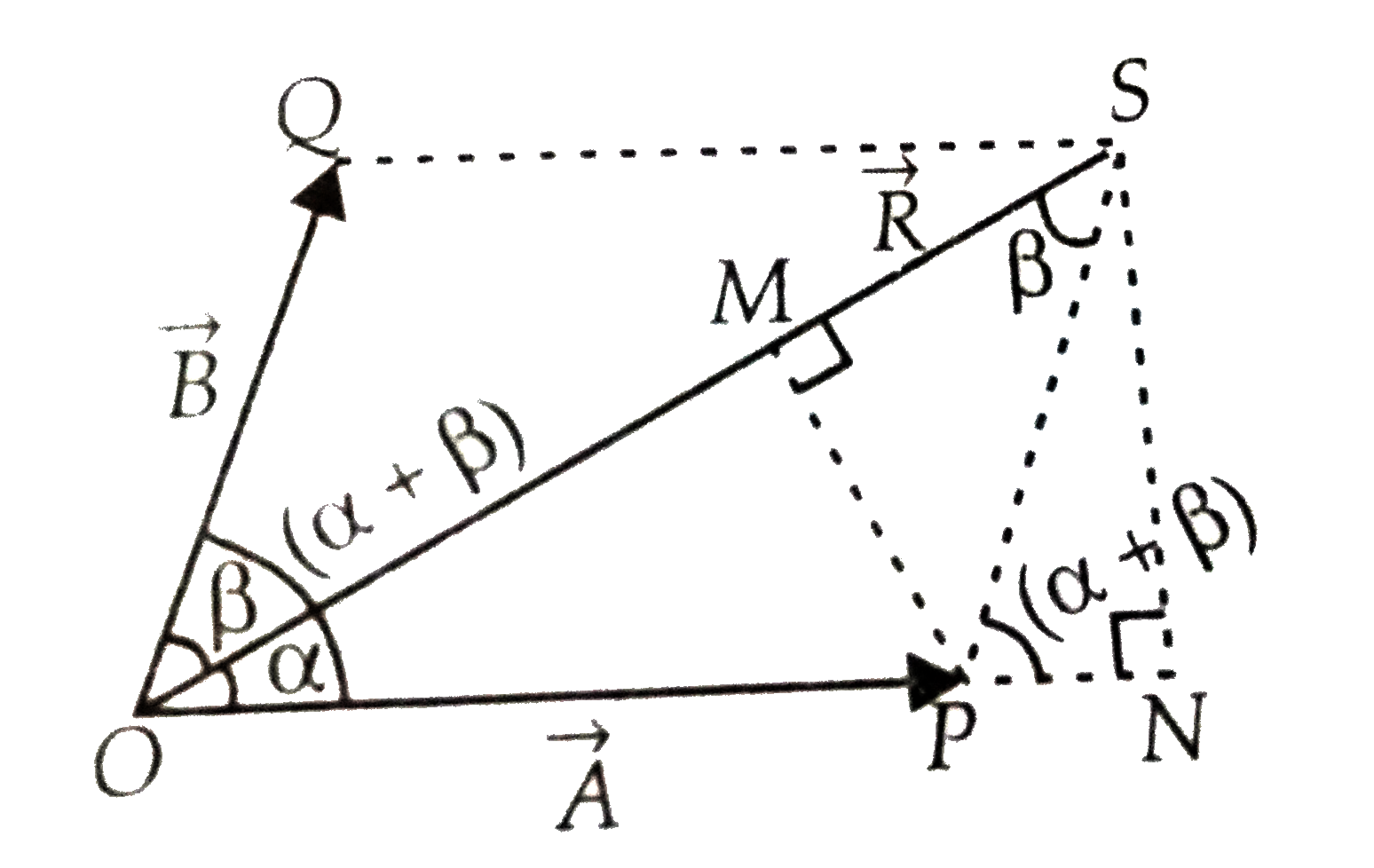
Let `vec(OP)` and `vec(OQ)` represent two vectors `vecA and vecB` making an angle `(alpha + beta)`. Using the parallelogram method of vector addition,
Resultant vector, `vecR = vecA + vecB` SN is normal to OP and PM is normal to OS.
From the geometry of the figure,
`OS^(2) + ON^(2) + SN^(2) = (OP + PN)^(2) + SN^(2)`
` = (A + Bcos(alpha + beta))^(2) + (Bsin(alpha + beta))^(2)`
`R^(2) = A^(2) + B^(2) + 2ABcos (alpha + beta)`
In `triangleOSN, SN = OS sin alpha = R sin alpha `
and in `triangle PSN, SN + pS sin (alpha + beta) = B sin (alpha + beta)`
`therefore R sinalpha = Bsin(alpha + beta) or (R)/(sin(alpha +beta)) = (B)/(sin alpha)" "...(i)`
Similary, PM ` = A sin alpha + B sin beta`
`(A)/(sin beta) = (B)/(sin alpha) " "...(ii)`
Combining (i) and (ii), we get
`(R)/(sin(alpha + beta)) = (A)/(sinbeta) = (B)/(sin alpha) " "...(iii)`
From eqn. (iii), `Rsinbeta = Asin(alpha + beta)`