In the set up shown in figure dltltD and d=4lambda, find.
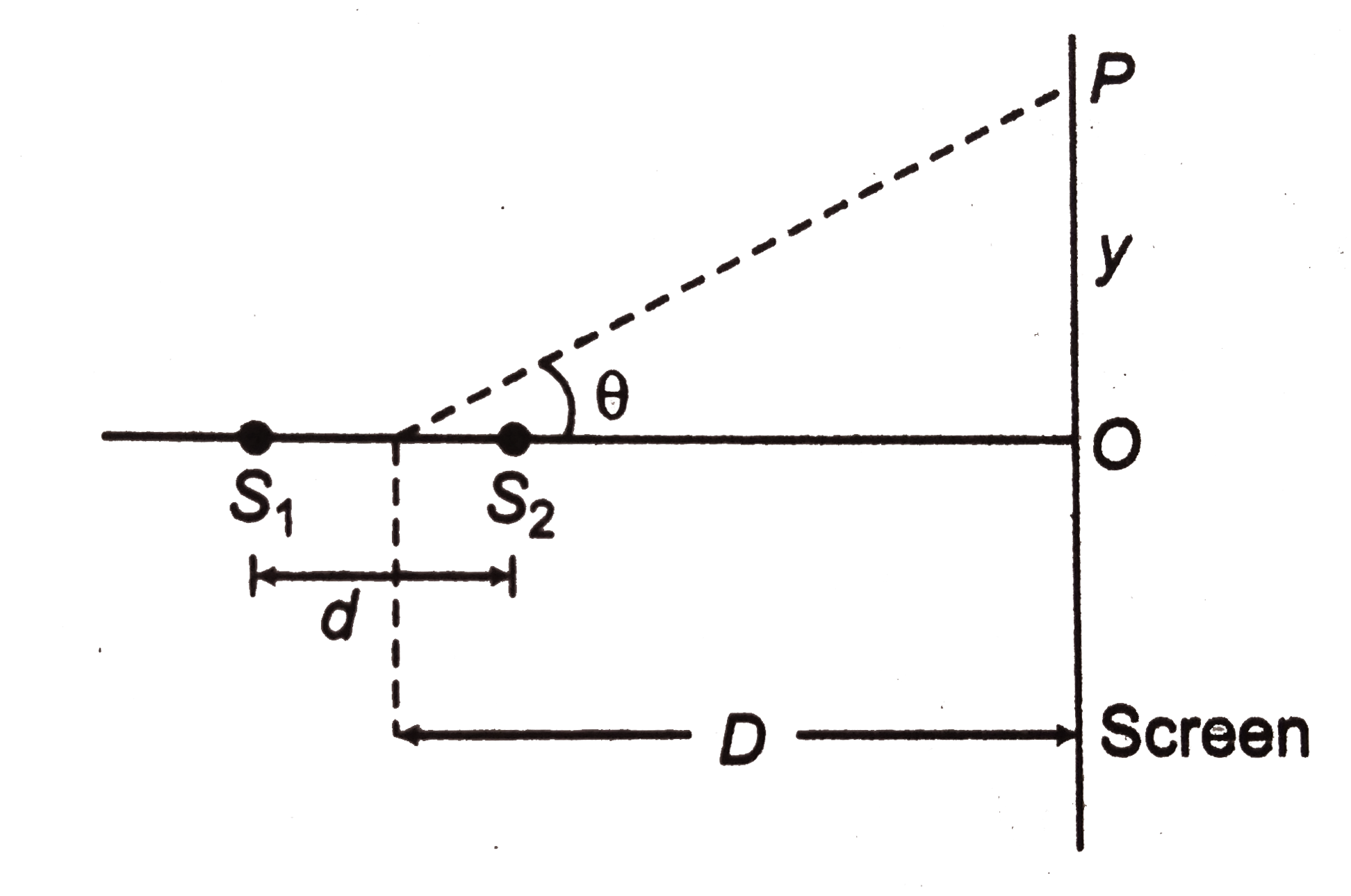
(a) total number of maximas and minimas on the screen
(b) y-coordinates corresponding to minima nearest to O.
(a) In the given set up, dltltD , therefore we can use
Δx=dcosθ
(Δx)min=0at θ=90∘
(Δx)max=d=4λ at θ=0∘
Therefore, total number of maximas are eight corresponding to
Δx=±4λ,±3λ,±2λand±λ
(b) Minima nearest to O are corresponding to
Δx=±3.5λ
∴dcosθ=±3.5λor4λcosθ=±3.5λ
or θ=±cos−1(3.5λ4λ) or θ=±cos−1(78)
Now, yD=tanθ⇒y=Dtanθ
∴y=±Dtan{cos−1(78)} .
Topper's Solved these Questions
- INTERFERENCE AND DIFFRACTION OF LIGHTBOOK - DC PANDEYCHAPTER - INTERFERENCE AND DIFFRACTION OF LIGHTEXERCISE - type-3
1 Videos
- INTERFERENCE AND DIFFRACTION OF LIGHTBOOK - DC PANDEYCHAPTER - INTERFERENCE AND DIFFRACTION OF LIGHTEXERCISE - Example Type 4
1 Videos
- INTERFERENCE AND DIFFRACTION OF LIGHTBOOK - DC PANDEYCHAPTER - INTERFERENCE AND DIFFRACTION OF LIGHTEXERCISE - Example Type 2
2 Videos
- GRAVITATIONBOOK - DC PANDEYCHAPTER - GRAVITATIONEXERCISE - All Questions
120 Videos
- MAGNETIC FIELD AND FORCESBOOK - DC PANDEYCHAPTER - MAGNETIC FIELD AND FORCESEXERCISE - Medical entrance s gallery
59 Videos
Similar Questions
In the YDSE apparatus shown in figure, d<<D and d=6λ. Find
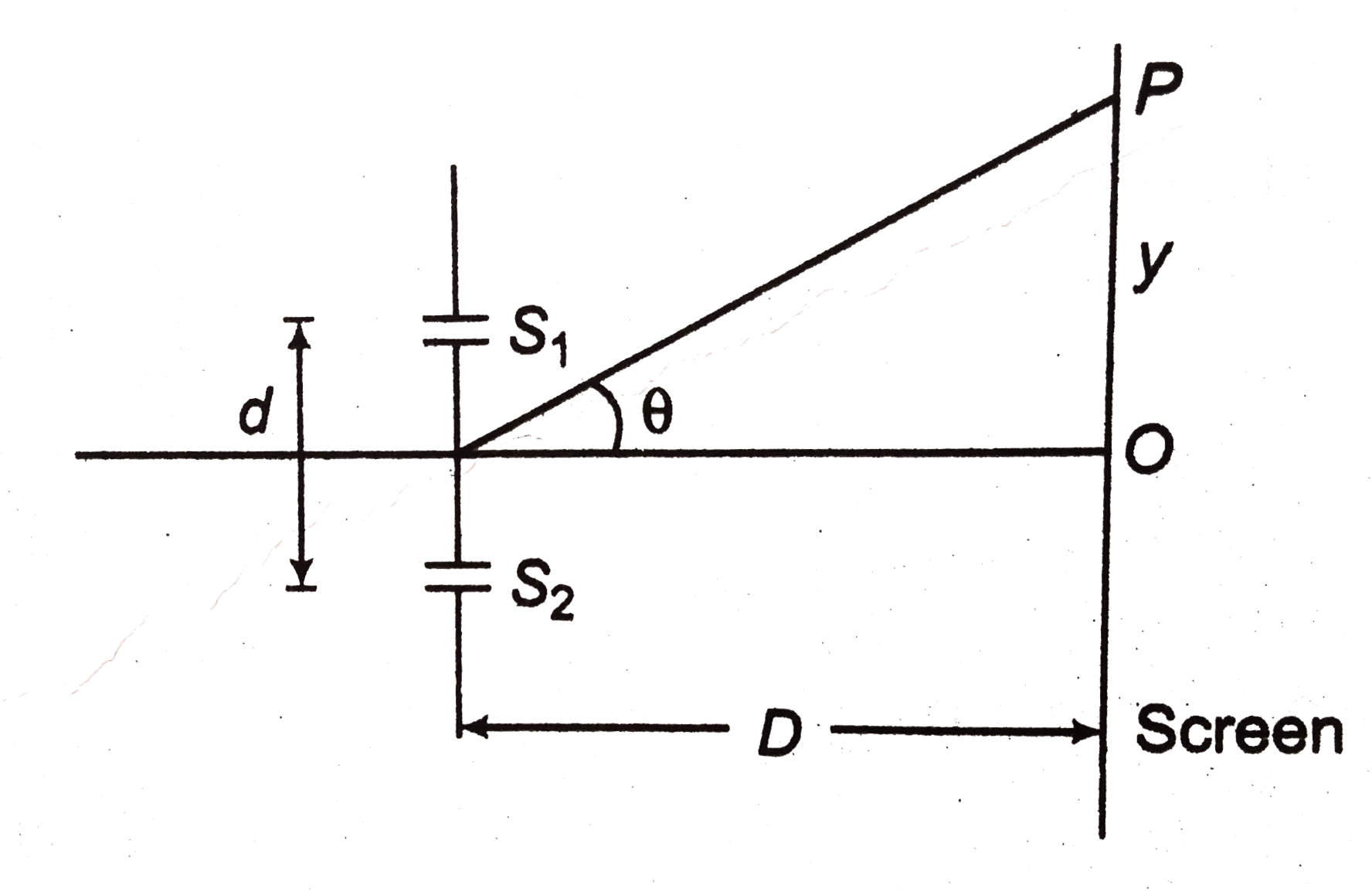
(a) total number of maximas and minimas on the screen .
(b) two y-coordinates corresponding to third order maxima.
View SolutionThere is a large circle (not a screen this time) around two coherent
sources S−1 and S2 kept at a distance d=3.4λ.
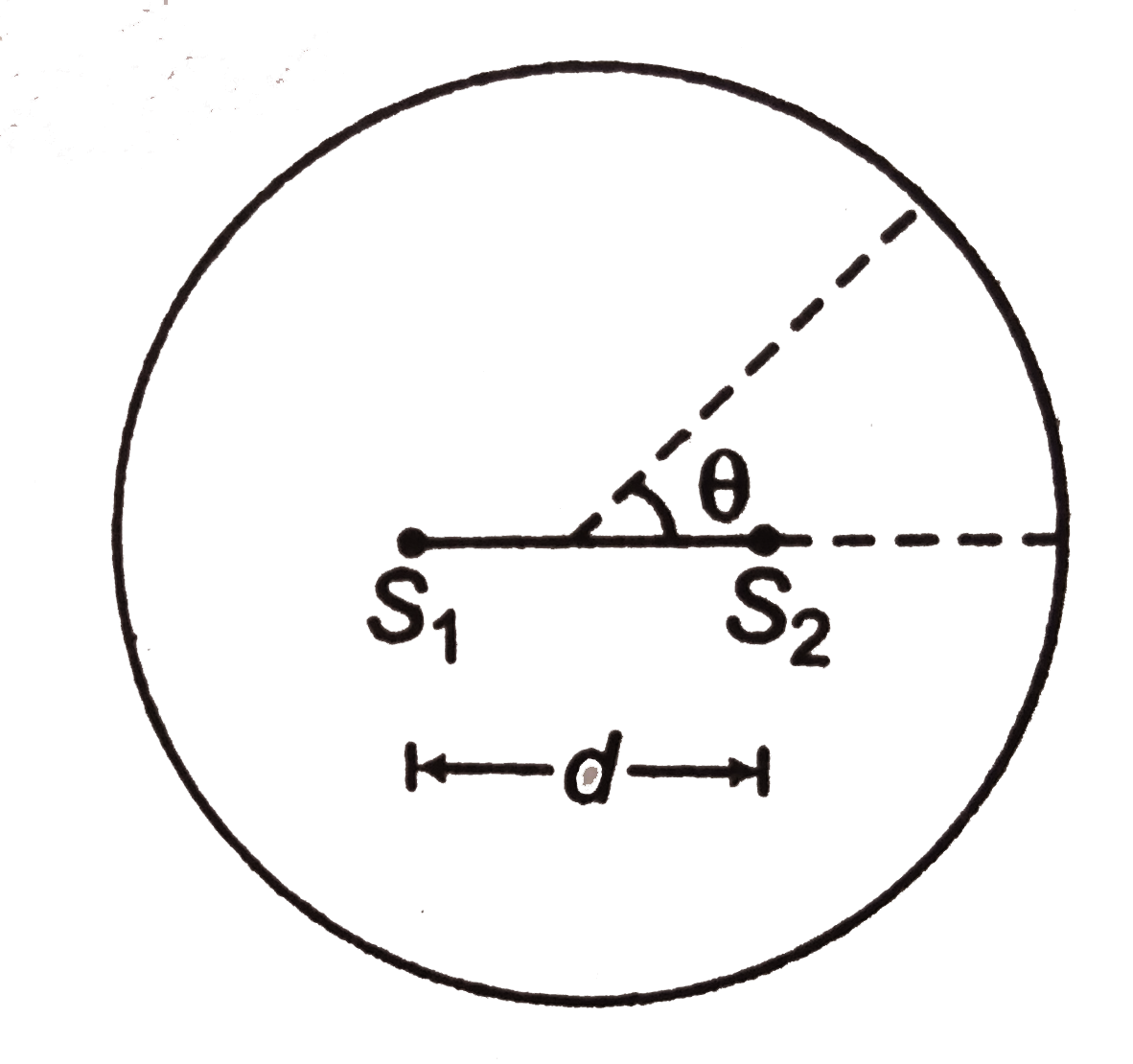
(a) Find total number of maximas on this circle.
(b) Four angular positions corresponding to third order maxima on this circle.
View Solution
Knowledge Check
In the diffraction from a single slit of width 2.5λ, the total number of minimas and secondary maximum (maxima) on either side of the central maximum are
A4 minimas, 2 secondary maximasB2 minimas, 2 secondary maximasC2 minimas, 4 secondary maximasD2 minimas, 3 secondary maximasIn the diffraction from a single slit of width 2.5λ, the total number of minimas and secondary maximum (maxima) on either side of the central maximum are
A4 minimas, 2 secondary maximasB2 minimas, 2 secondary maximasC2 minimas, 4 secondary maximasD2 minimas, 3 secondary maximasStatement I: For the situation shown in figure two identecal coherent light sources produce interference pattern on the screen. The intensity of minima nearest to S1 is not exactly zero.

Statement II: Minimum intensity is zero, when interfering waves have same intensity at the location of superposition.AStatement I is True, statement II is True, Statement II is a correct explanation for Statement I.BStatement I is Ture, Statement II is Ture, Statement II is NOT a correct explanation for Statement I.CStatement I is True, Statement II is False.DStatement I is False, Statement II is True.
In a YDSE, D = 1m, d = 1mm and λ=1/2mm
Find the no of maxima and minima obtained on the screen.
View SolutionA monochromatic light of wavelength λ is incident normally on a narrow slit of width a to produce a diffraction pattern on the screen placed at a distacne D from the slit. With the help of a relevant diagram, deduce the condition for maxima and minima on the screen. use these condition to show that angular width of central maximum is twice the angular width of secondary maximum.
View SolutionIn a YDSE, D=1M,d=1mm,andλ=1/2mm.
(a) Find the distance between the first and central maxima on the screen.
(b) Find the number of maxima and minima obtained on the screen.
View SolutionA coherent parallel beam of microwaves of wavelength A = 0.5 mm fails on a Young's double slit apparatus. The separation between maxima is measured on a screen placed paraiiei to the plane, of the slits at a distance of 1.0 m from it as shown in figtird. The separation between the slits is 2d - 1mm.
(a) If the incident beam fails normally on the double slit apparatus, find the y-coordinates of all the interface minima of the screen.
(b) If the incident beam makes an angle of 30° with the x-axis (as shown in fig. find the y-coordinates of the first minima on either side of the central maximum.
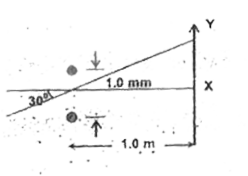
View SolutionAssertion: Distance between two coherent sources S1andS2 is 4λ. A large circle is drawn around these sources with centre of circle lying at centre of S1andS2. There are total 16 maxima on this circle.
Reason: Total number of minimas on this circle are less, compared to total number of maximas.
View Solution
