The region between x=0 and x=L is filled with uniform steady magnetic field B0ˆk. A particle of mass m, positive charge q and velocity v0ˆi travels along x-axis and enters the region of the magnetic field. Neglect the gravity throughout the question.
a. Find the value of l if the particle emerges from the region of magnetic field with its final velocilty at an angel 30∘ to its initial velocity.
b. Find the final velocity of the particle and the time spent by it in the magnetic field, if themagnetic field now extends upto 2.1L.
a. θ=30∘
sinθ=LR
Here,R=mv0B0q

∴Sin30∘=Lmv0B0q
=12=B0qLmv0
∴L=mv02B0q
b. In part a.
sin30∘=LRor12=LR
orL=R2
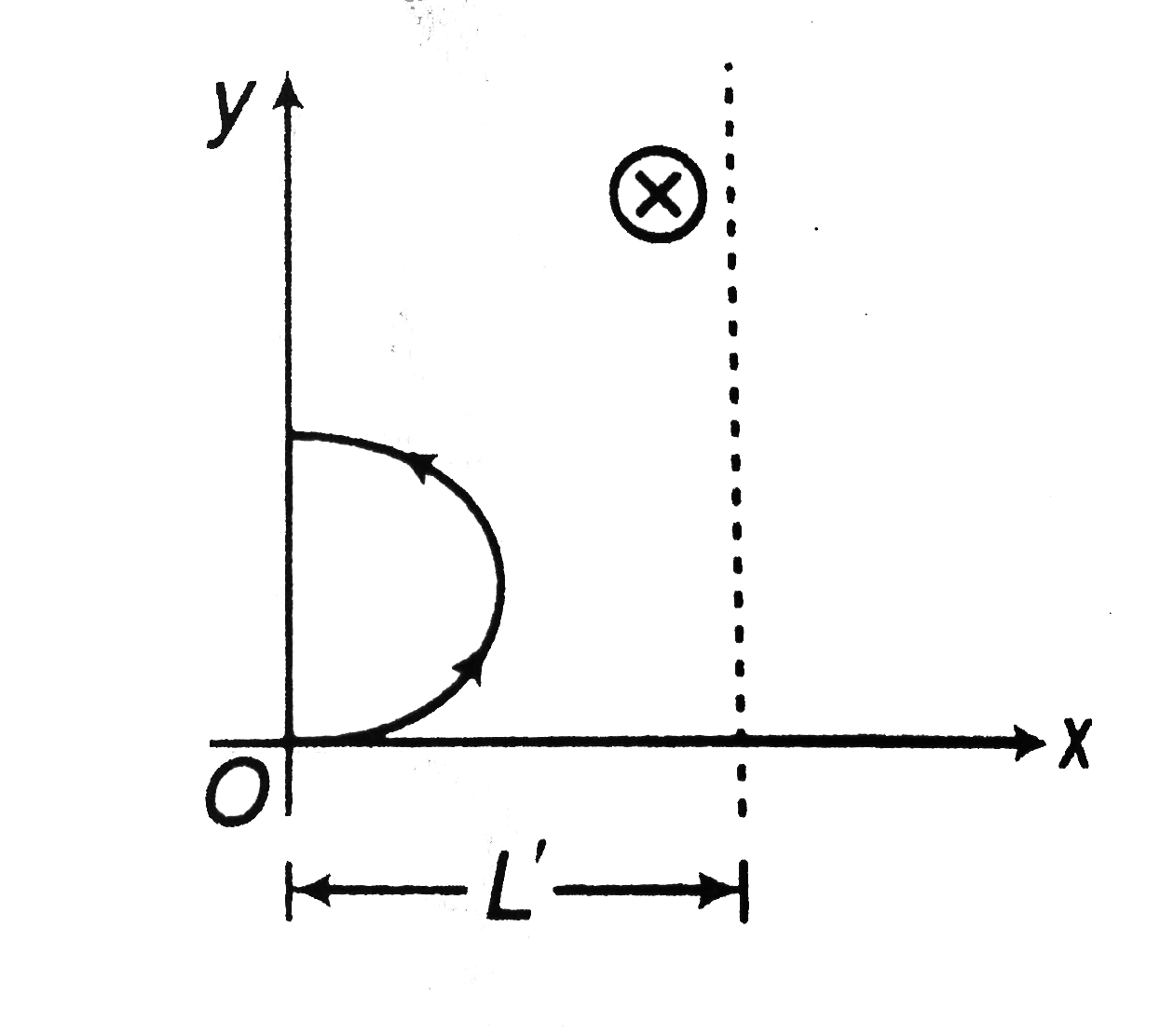
Now when L'=2.1L
or 2.12R⇒L'>R
therefore devistion of the particle is θ=180∘ as shown in figure
∴vf=v0ˆi
and tAB=T2=πmB0q
Topper's Solved these Questions
- MAGNETICSBOOK - DC PANDEYCHAPTER - MAGNETICSEXERCISE - Exercise
160 Videos
- MAGNETICSBOOK - DC PANDEYCHAPTER - MAGNETICSEXERCISE - INTRODUCTORY EXERCISE
1 Videos
- MAGNETIC FIELD AND FORCESBOOK - DC PANDEYCHAPTER - MAGNETIC FIELD AND FORCESEXERCISE - Medical entrance s gallery
59 Videos
- MAGNETISM AND MATTERBOOK - DC PANDEYCHAPTER - MAGNETISM AND MATTEREXERCISE - Medical gallery
1 Videos
Similar Questions
The region between x=oandx=L is filled with uniform, steady magnetic field B0ˆk. A particle of mass m , positive charge q and velocity v0ˆi travels along x−aξs and enters the region of the magnetic field. Neglect gravity throughout the question .
(a) Find the value of L if the particle emerges from the region of magnetic field with its final velocity at angle 30∘ to its initial velocity.
(b) Find the velocity of the particle and the time spent by it in the magnetic field, if the magnetic field now extends upto 2.1L.
View SolutionA particle of mass m and charge q moving with velocity v enters the region of uniform magnetic field at right angle to the direction of its motion. How does its kinetic energy get affected?
View Solution
Knowledge Check
The region between x=0 and x=L is filled with uniform constant magnetic field 25(T)ˆk. A particle of mass m=50g having positive charge q=1C and velocity →V0=50√3ˆi+50ˆjms−1 enters the region of the magnetic field. Neglect gravity throughout the question.
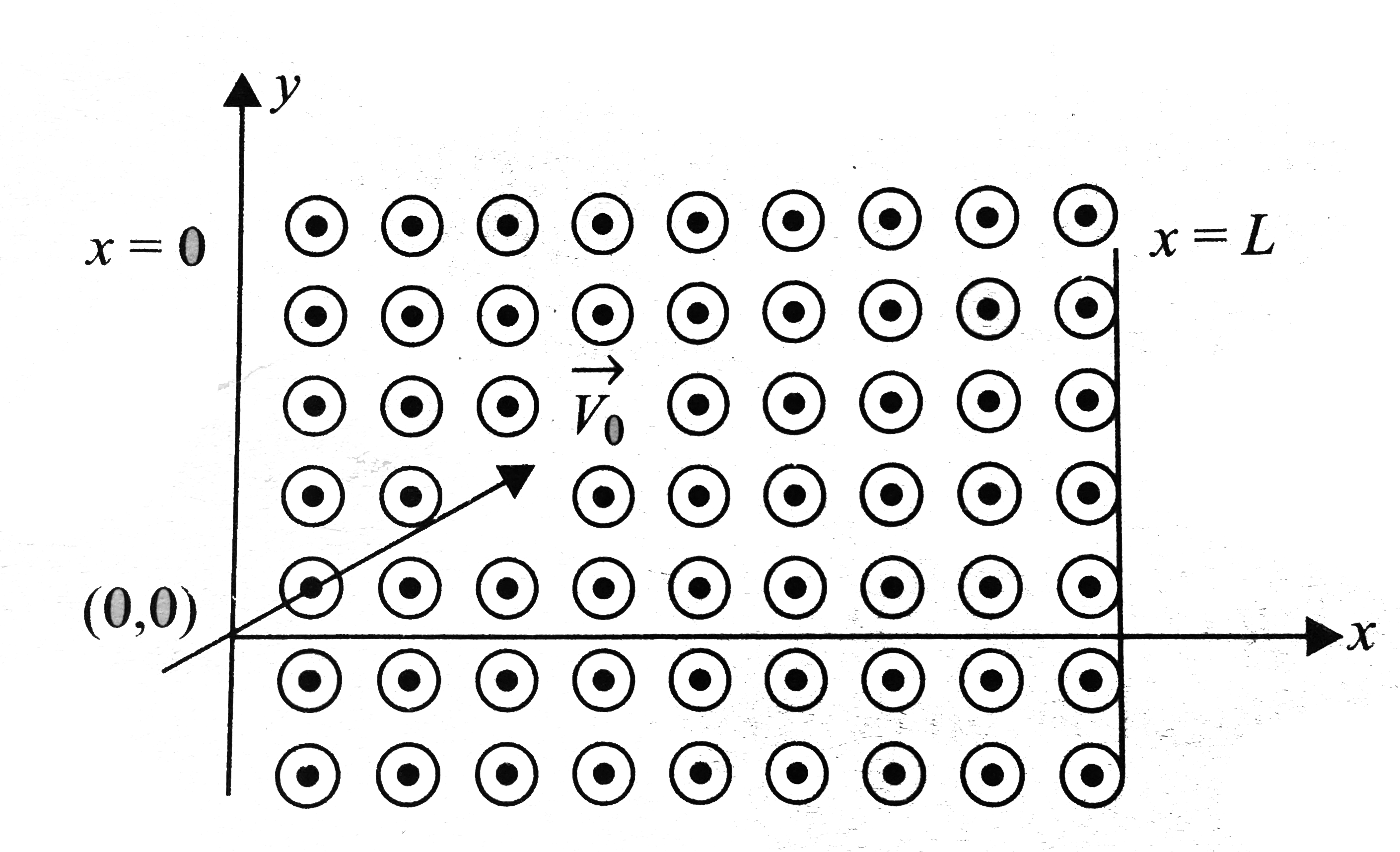
The value of L if the particle emerges from the region of magnetic field with its velocity 100(ms−1)ˆi isA20cmB10cmC30cmDNone of theseThe region between x=0 and x=L is filled with uniform constant magnetic field 25(T)ˆk. A particle of mass m=50g having positive charge q=1C and velocity →V0=50√3ˆi+50ˆjms−1 enters the region of the magnetic field. Neglect gravity throughout the question.
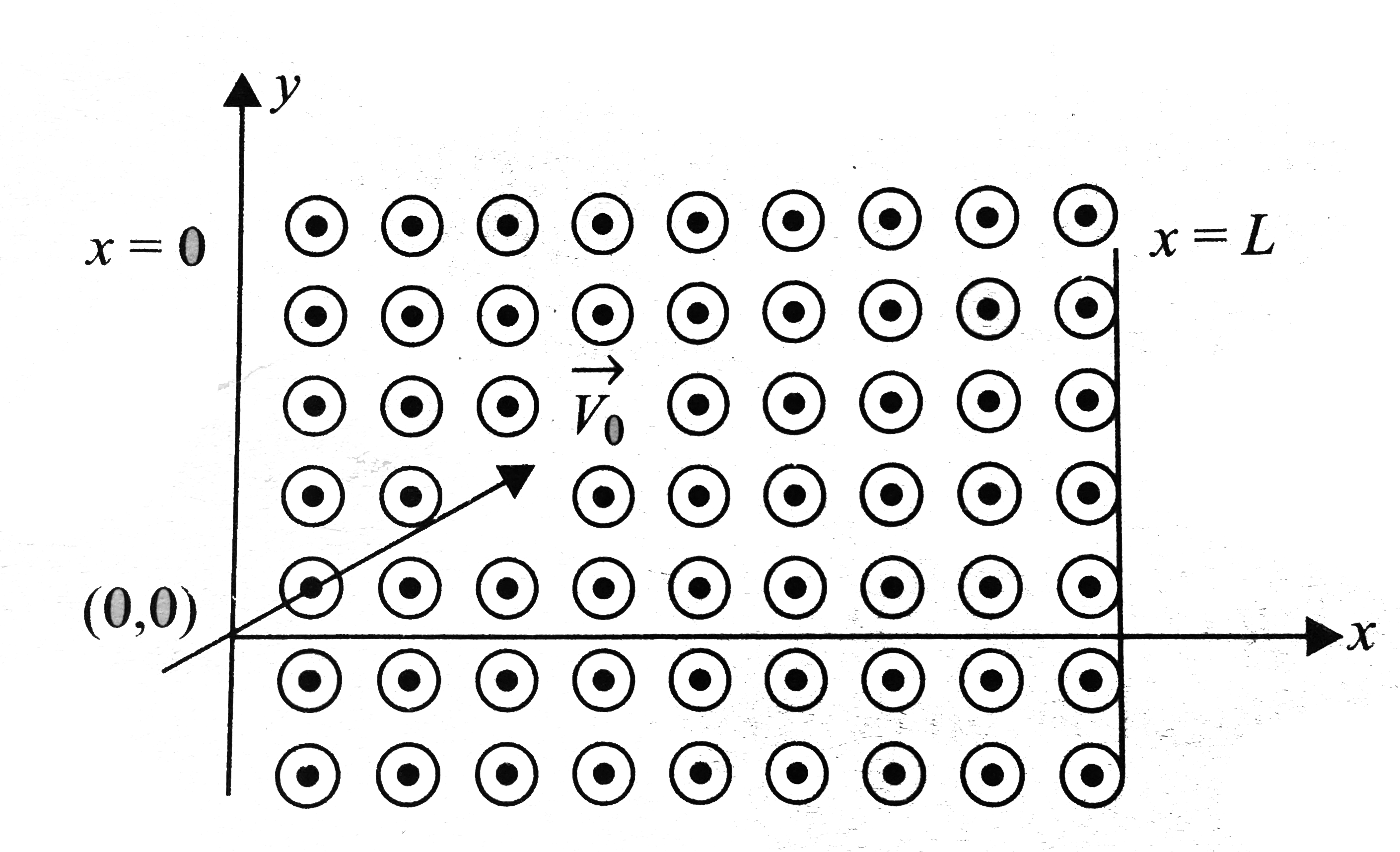
The maximum and minimum values of L such that velocity of emerging particle makes angle π/3 with the y-axis areALmin=10cm,Lmax=∞BLmin=20cm,Lmax=40cmCLmin=10cm,Lmax=20cmDLmin=20cm,Lmax=∞A particle of charge q and mass m moving with a velocity v along the x-axis enters the region x>0 with uniform magnetic field B along the ˆk direction. The particle will penetrate in this region in the x-direction upto a distance d equal to
AZeroBmvqBC2mvqBDinfinity
A positive charge particle of charge q, mass m enters into a uniform magnetic field with velocity v as shown in the figure. There is no magnetic field to the left of PQ. Find
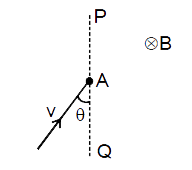
distance travelled in the magnetic field
View SolutionThe figure shows two regions of uniform magnetic field of strengths 2B and 2B. A charged particle of mass m and charge q enters the region of the magnetic field with a velocity υ=qBwm, where w is the width of each region of the magnetic field. The time taken by the particle to come out of the region of the magnetic field is
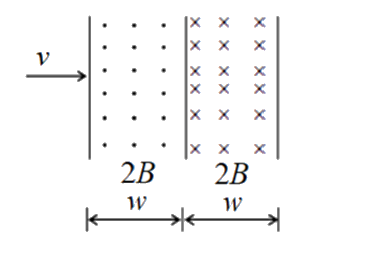
View SolutionA positive charge particle of charge q and mass m enters into a uniform magnetic field with velocity v as shown in Fig. There is no magnetic field to the left of PQ. Find (a) time spent, (b) distance travelled in the magnetic field, (c) impulse of magnetic force.
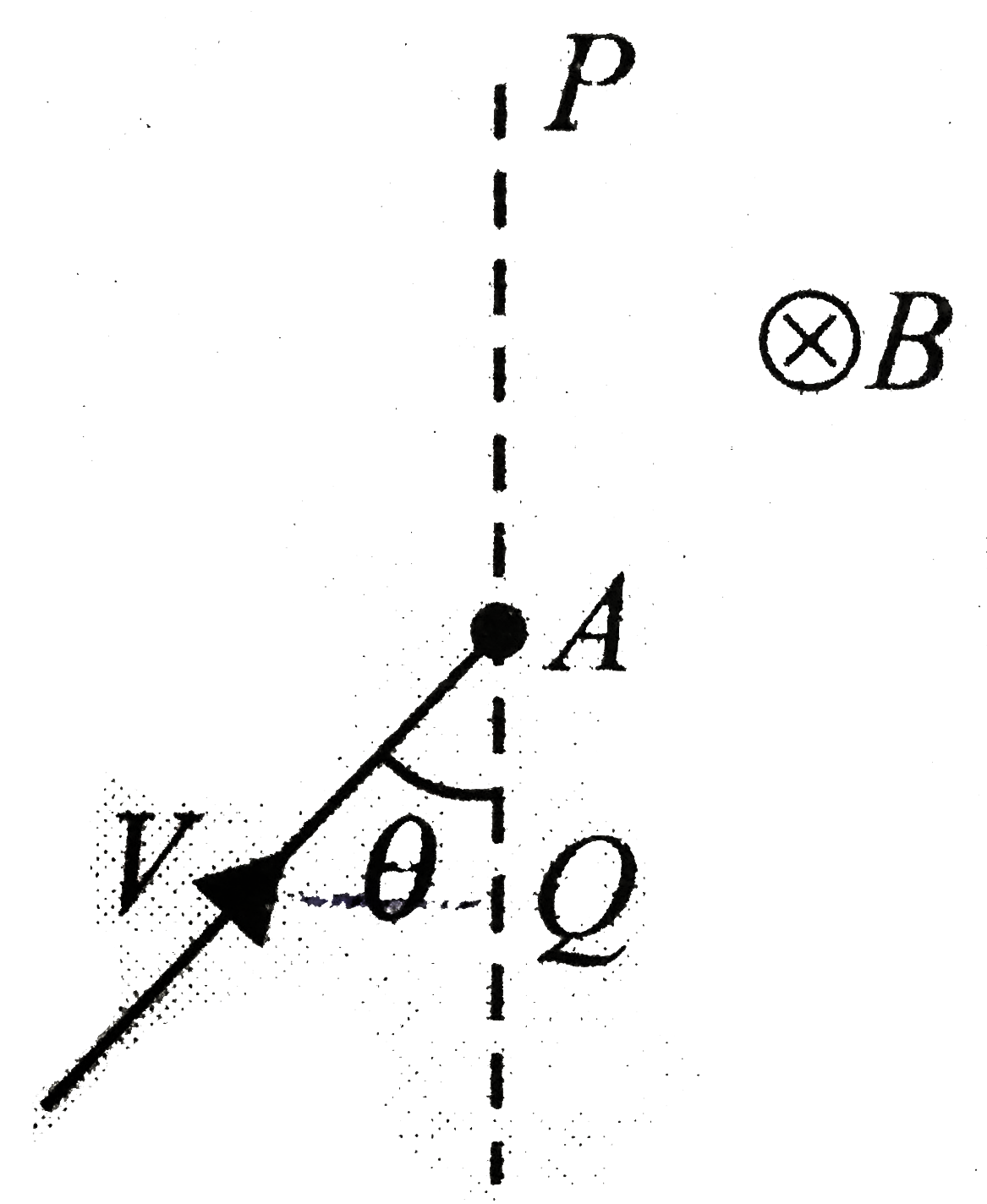
View SolutionA particle of the mass m and cgharge q moves with a constant velocity v along the positive x−direction.Itentersaregionconta∈∈gauniformmag≠ticfieldBdirectedalongtheminiμmvalueofvrequiredsotˆthepartic≤canjustentertheregion xgtb is
View SolutionLet y>0 be the region of space with a uniform and constant magnetic field Bˆk. A particle with charge and mass m travels along the y-axis and enters in magnetic field at origin with speed v0 in region in particle is subjected to an additional friction force →F=−k→v. Assume that particle remains in region y>0.
View Solution
