A charged particle of mass m=1 kg and charge q=2μC is thrown from a horizontal ground at an angle θ=45∘ with the speed 25m/s. In space, a horizontal electric field E=2×107 V/m exists in the direction of motion. Find the range on horizontal ground of the projectile thrown. Take g=10m/s2.
The correct Answer is:A, B, C
T=2usinθg=2×25×sin45∘10=5√2s
R=Sx=uxT+12axT2
=(ucos45∘)(T)+12(qEm)T2
=(25)(1√2)(5√2)+(2×10−6×2×1071)(5√2)2
=62.5+250=312.5m
Topper's Solved these Questions
- ELECTROSTATICSBOOK - DC PANDEYCHAPTER - ELECTROSTATICSEXERCISE - Level 1 Subjective
15 Videos
- ELECTROSTATICSBOOK - DC PANDEYCHAPTER - ELECTROSTATICSEXERCISE - SUBJECTIVE_TYPE
6 Videos
- ELECTROSTATICSBOOK - DC PANDEYCHAPTER - ELECTROSTATICSEXERCISE - Level 1 Assertion And Reason
19 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITORSBOOK - DC PANDEYCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - (C) Chapter exercises
50 Videos
- GRAVITATIONBOOK - DC PANDEYCHAPTER - GRAVITATIONEXERCISE - All Questions
120 Videos
Similar Questions
A charged particle of mass m=1 kg and charge q=2μC is thrown for a horizotal ground at an angle θ=45∘ with speed 20m/s. In space a horizontal electric field E=2×107 V/m exist. Find the range on horizontal ground of the projectile thrown.
View SolutionA particle of mass m and charge q is thrown with initial velocity v0 at an angle with the horizontal. In space there exist an electric field of strength E at angle β with the downward vertical away from the point of projection. Find the time of flight and range of projectile on horizontal ground
View Solution
Knowledge Check
A charged particle of mass m=2kg and charge 1μC is thrown from a horizontal ground at an angle of 45∘ with horizontal with a spedd of 10m/s. In space a horizontal electric field E=2×107N/C exists. Find the range of the projectile
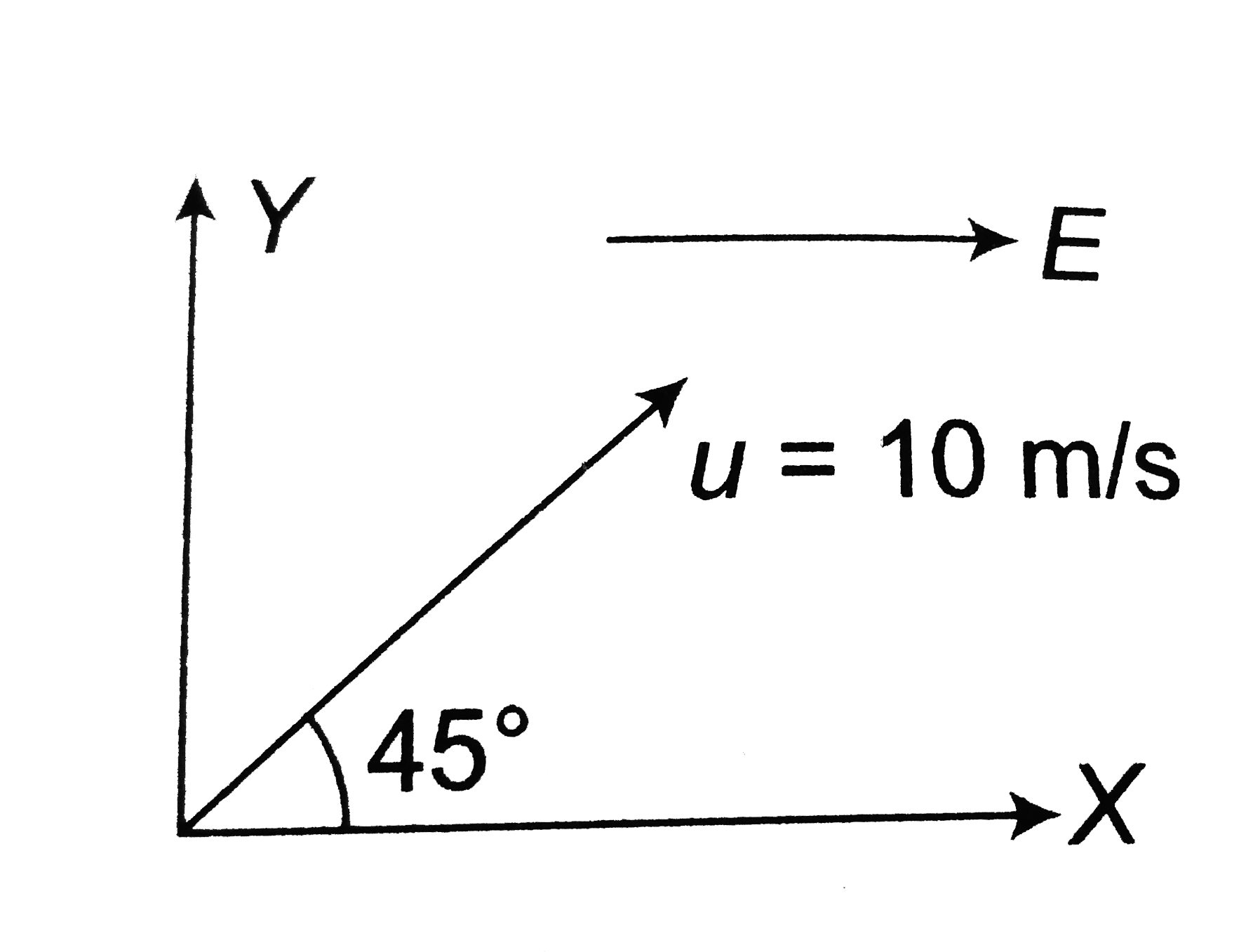 A5mB30mC10mD20m
A5mB30mC10mD20mA charged particle of mass m = 2 kg and charge 1μC is projected from a horizontal ground at an angle θ=45∘ with speed 10ms−1. In space, a horizontal electric field towards the direction of projection E=2×107NC−1 exists. The range of the projectile is
A20 mB60 mC200 mD180 mA particle is projected at an angle θ from ground with speed u (g = 10 m/s2 )
Aif u = 10 m/s and θ=30∘, then time of flight will be 1 secBIf u=10√3m/sandθ=60∘, then time of flight will be 3 sec.Cif u=10√3m/sandθ=60∘, then after 2 sec velocity becomes perpendicular to initial velocity.Dif u = 10 m/s and θ=30∘, then velocity never becomes perpendicular to initial velocity during its flight.
A particle having mass m and charge q is projected at an angle of 75° to the horizontal with a speed u. A uniform electric field E=mgq exists in horizontal direction. Find the time after projection when the velocity of particle makes an angle of 45° with horizontal.
View SolutionA simple pendulum with a bob of mass m=1 kg, charge q=5μC and string length imis given a horizontal velocity a in a uniform electric field E=2×106Vm at its bottommost point A, as shown in figure. It is given that the speed a is such that the particle leaves the circle at point C. Find the speed a (Take g=10ms2)
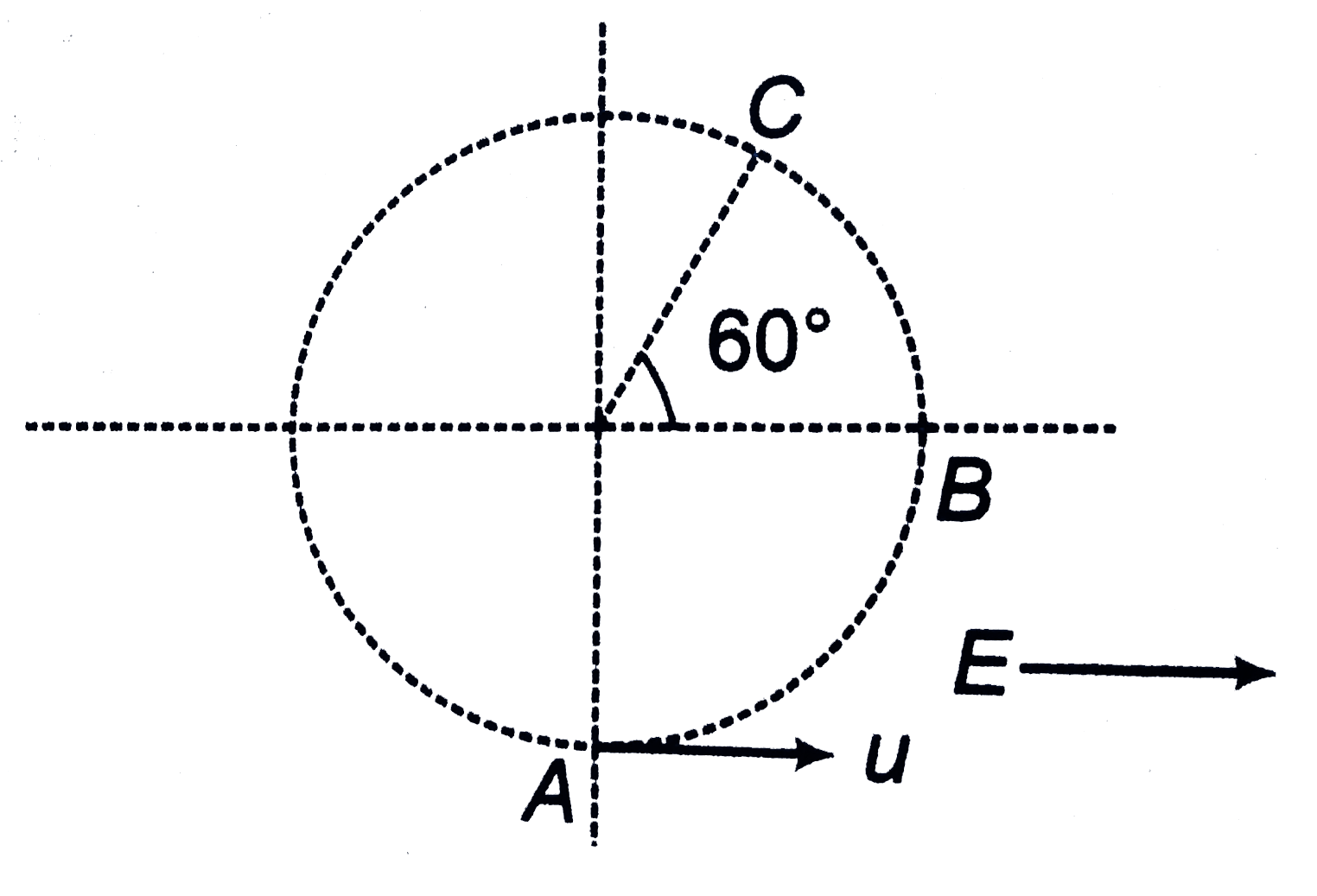
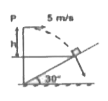
View SolutionA particle of mass m is thrown horizontally from point P , as shown in the figure, with speed 5m/s. It strikes an inclined plane perpendicularly as shown. The inclination of the plane is 30∘ with the horizontal. Then, the height h shown in the figure is ( Take g=10m/s2 ) .
View SolutionA particle of mass m and charge q is thrown in the vertical direction with a velocity v0 in a uniform horizontal electric field E. Assuming the gravity force to be negligible ,the equation of the path followed by the particle will be
View SolutionA projectile is thrown with velocity u making an angle θ with the horizontal. Its time of flight on the horizontal ground is 4 second. The projectile is moving at angle of 45∘ with the horizontal just one second after the projection. Hence the angle θ is (take g=10m/s2)
View Solution
