A cone made of insulating material has a total charge Q spread uniformly over its sloping surface. Calculate the work done in bringing a small test charge q from infinity to the apex of the cone. The cone has a slope length L.
The correct Answer is:B

W=−△U=Uapex−U∞
W=qVapex ………..i
asU∝=0
Vapexrx=RL
∴r=(RL)x
Surface charge density
σ=QπRl
dq=(σ)(dA)
=(QπRL)(2πr)(RXx)dx
=(θπRL)(2π)(RLx)dx
=(2QL2)xdx
Now,dV=(14πε0)(dqx)
=(2Q4πεL0^(2))dx
∴V=∫L0dV=Q2πε0L
substituting in eqn i we have
W=Qq2πε0L
Topper's Solved these Questions
- ELECTROSTATICSBOOK - DC PANDEYCHAPTER - ELECTROSTATICSEXERCISE - Level 1 Assertion And Reason
19 Videos
- ELECTROSTATICSBOOK - DC PANDEYCHAPTER - ELECTROSTATICSEXERCISE - Level 1 Objective
82 Videos
- ELECTROSTATICSBOOK - DC PANDEYCHAPTER - ELECTROSTATICSEXERCISE - Exercise 24.6
2 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITORSBOOK - DC PANDEYCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - (C) Chapter exercises
50 Videos
- GRAVITATIONBOOK - DC PANDEYCHAPTER - GRAVITATIONEXERCISE - All Questions
120 Videos
Similar Questions
A cone made of insulating material has a total charge Q spread uniformly over its sloping surface. Calculate the energy required to take a test charge q from infinity to apex A of cone. The slant length is L.
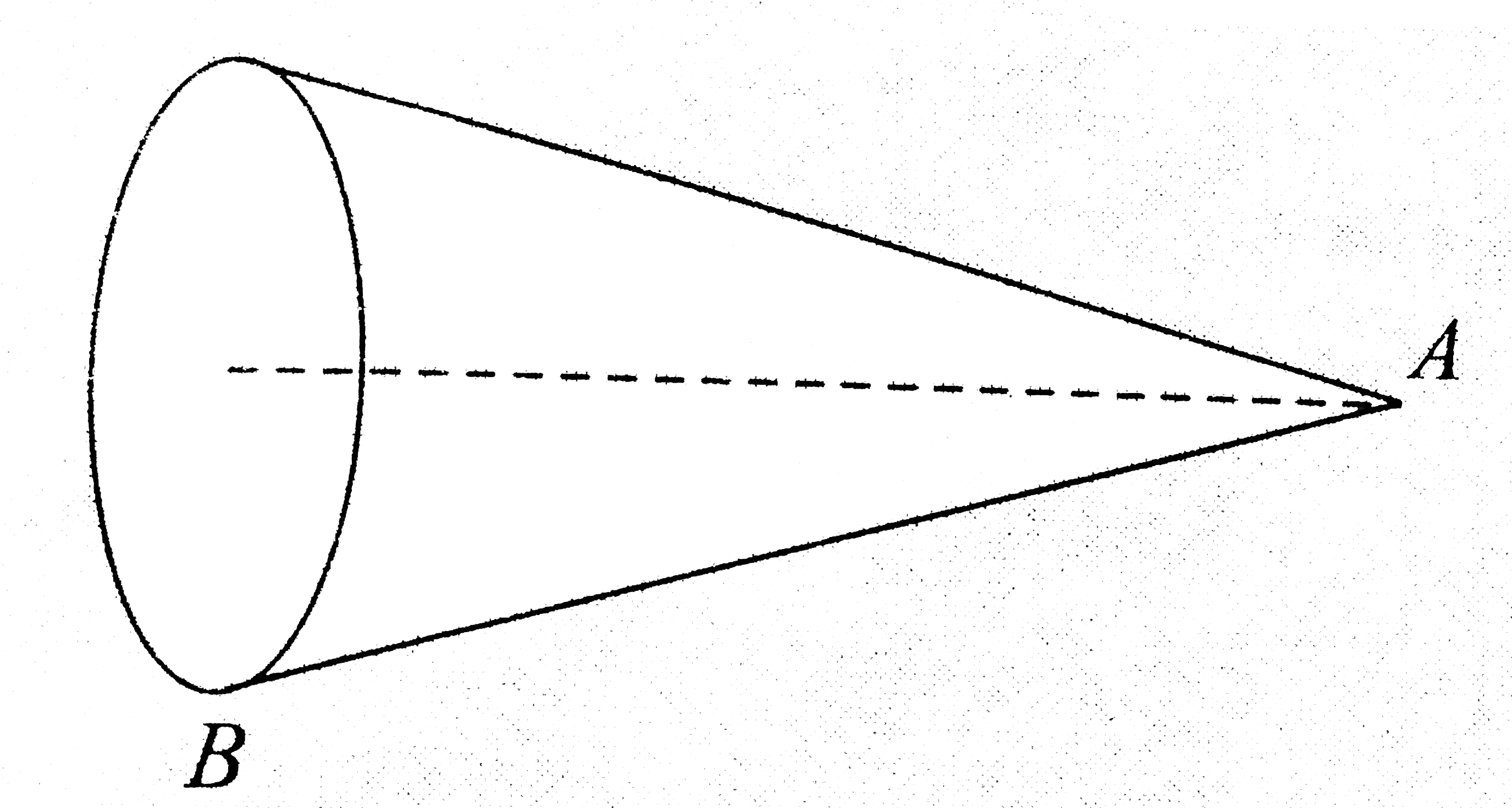 .
.
View SolutionFour identical charges q are kept on each vertices of a square of side a. Calculate the extra amount of work done in bringing a Q charge from infinity to the centre of the square.
View Solution
Knowledge Check
If 6W is the work done in bringing a infinitesimal small test charge 6g from infinity to a point P, then potential at point P is
AδWδqB−δWδqC−δW.δqDNone of theseThree equal charges Q are placed at the three corners of an equilateral triangle of side length a. Work done in shifting a charge q from infinity to the centroid of the triangle is
A3Qq4π∈0aB3Qq4π∈0√3aC3√3Qq4π∈0aD√3Qq4π∈03aFour equal charges Q are placed at the four corners of a square of each side is 'a'. Work done in removing a charge −Q from its centre to infinity is
AzeroB√2q2π∈0aC√2qπ∈0aDq2π∈0a
Over a thin ring of radius R a charge q is distributed none-uniformly. Calculate the work done of the force field in displacing a point charge q' from centre of the ring to infinity.
View SolutionA thin disc of dielectric material, with a total charge +q distributed uniformly over its surface rotates n times per second about an axis perpendicualr to the surface of the disc and passing through its center. Find the magnetic induction at the center of the disc. The radius of the disc is equal to r.
View SolutionDetermine the work which has to be done to bring an electric charge e2=1 from infinity to a unit distance from a charge e1.
View SolutionThe electirc field strength at a distance r from the centre of a charged sphere of radius r is E. If r>R, how much work will be done in bringing a test charge q0 from infinity to that point, is
View SolutionA charge Q is placed at the origin. The electric potential due to this charge at a given point in space is v. The work done by an external force in bringing another charge q from infinity up to the point is
View Solution
