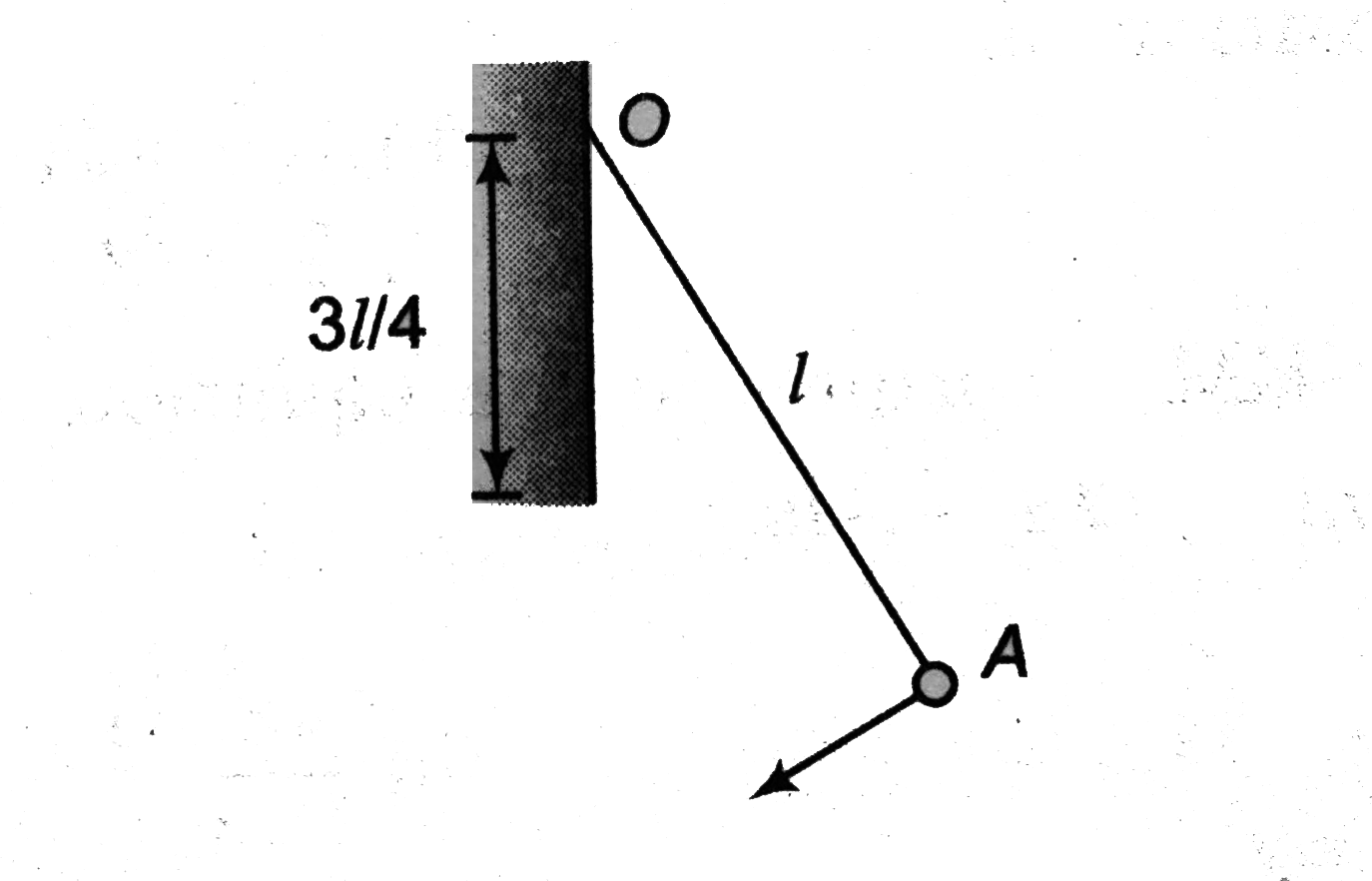
A small bob attached to a light inextensible thread of length l has a periodic time T when allowed to vibrate as a simple pendulum. The thread is now suspended from a fixed end O of a vertical rigid rod of length 3l/4. If now the pendulum performs periodic oscillations in this arrangement, the periodic time will be
A
3T/4
B
4T/5
C
2T/3
D
5T/6
Video Solution
Text Solution
The correct Answer is:A
Half of the oscillation is completed with length l and rest half with l/4.
∴ Time period =T12+T22
=12[2π√lg+2π√l/4g]
=34[2π√lg]=34T
|
Topper's Solved these Questions
- SIMPLE HARMONIC MOTIONBOOK - DC PANDEYCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Level 2 More Than One Correct
8 Videos
- SIMPLE HARMONIC MOTIONBOOK - DC PANDEYCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Level 2 Comprehension
2 Videos
- SIMPLE HARMONIC MOTIONBOOK - DC PANDEYCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Level 1 Subjective
39 Videos
- SEMICONDUCTORS AND ELECTRONIC DEVICESBOOK - DC PANDEYCHAPTER - SEMICONDUCTORS AND ELECTRONIC DEVICESEXERCISE - More than One Option is Correct
3 Videos
- SOLVD PAPERS 2017 NEET, AIIMS & JIPMERBOOK - DC PANDEYCHAPTER - SOLVD PAPERS 2017 NEET, AIIMS & JIPMEREXERCISE - Solved paper 2018(JIPMER)
38 Videos
Similar Questions
Knowledge Check
A small bob attached to a light inextensible thread of length l has a periodic time T when
allowed to vibrate as a simple pendulum. The thread is now suspended from a fixed end O of a vertical rigid rod of length 3l4 (as in figure). If now the pendulum performs periodic oscillations in this arrangement, the periodic time will be-
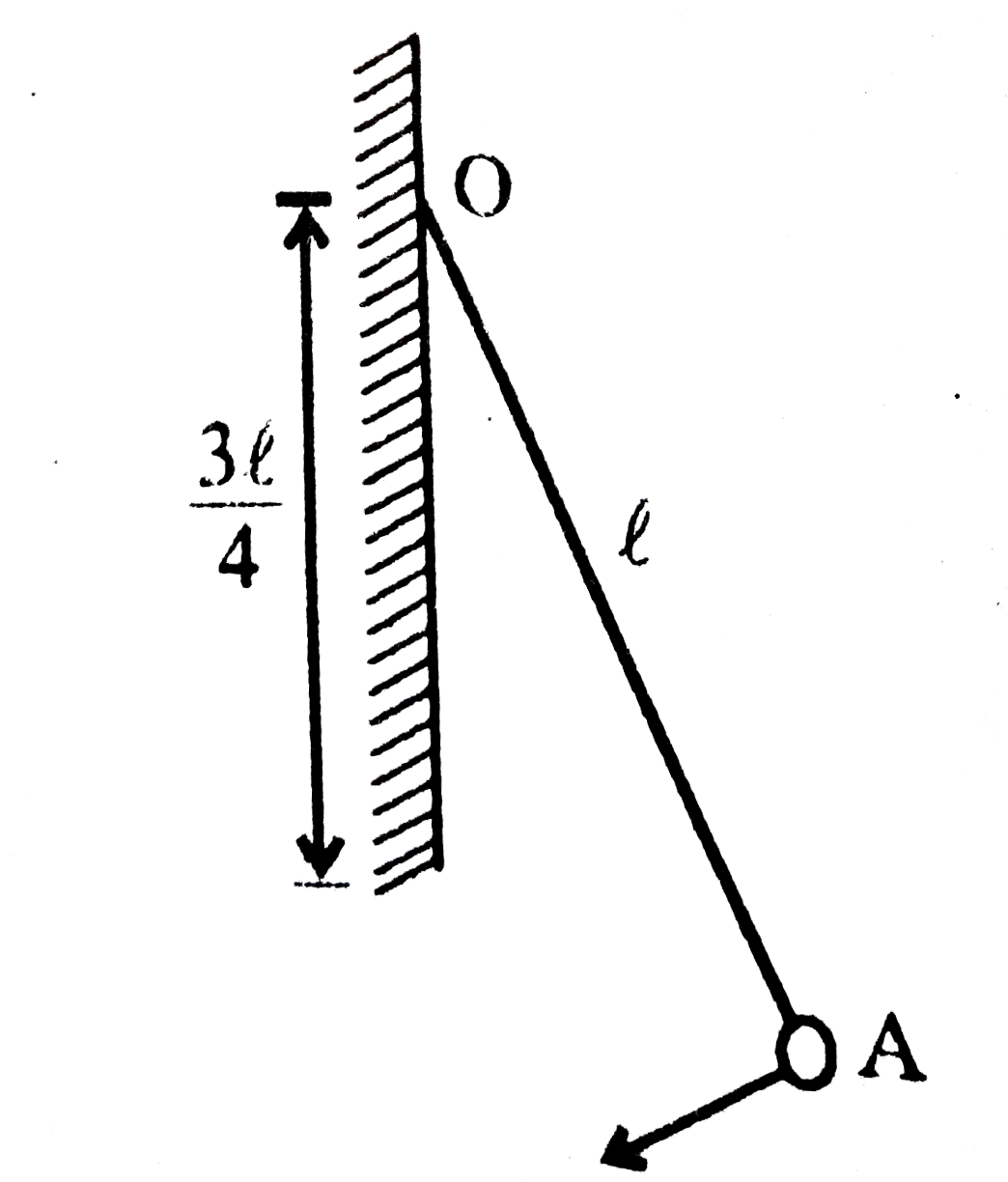 A3T4BT2CTD2T
A3T4BT2CTD2TIn a simple pendulum the period of oscillation (T) is related to the length of the pendulum (L) as
AL/T=ConstantBL2/T2= ConstantCL/T2=ConstantDLT=ConstantA simple pendulum has time period T. A uniform rod, whose length is the same as that of the pendulum, undergoes small oscillations about its upper end. Its time period of oscillation will be -
A<TBTC>TDmay be (A), (B) or (C) depending on whether T is < equal to or >2seconds
The time period of a simple pendulum in air is T. Now the pendulum is submerged in a liquid of density ρ16 where ρ is density of the bob of the pendulum. The new time period of oscillation is.
View SolutionA trolley moves in horizontal direction with an acceleration a. A simple pendulum of length l is suspended from the roof of the trolley. The time period of the pendulum will be
View SolutionThe graph of time period (T) of simple pendulum versus its length (l) is
View SolutionThe graph between time period (T) and length (l) of a simple pendulum is
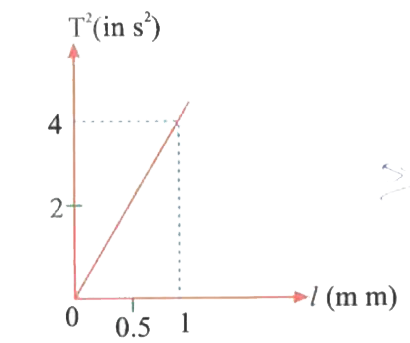
View SolutionThe l−T2 graph of a simple pendulum is an shown in the figure. The time period of the pendulum of length 0.5 mm is
View Solution