Derive Laplace's law for a spherical membrane.

Video Solution

Text Solution

Verified by Experts

Consider a spherical liquid and let the outside pressure be $P_{0}$ and inside presure be $P_{i}$ , such that the excess pressure is $P_{i} - P_{0} .$

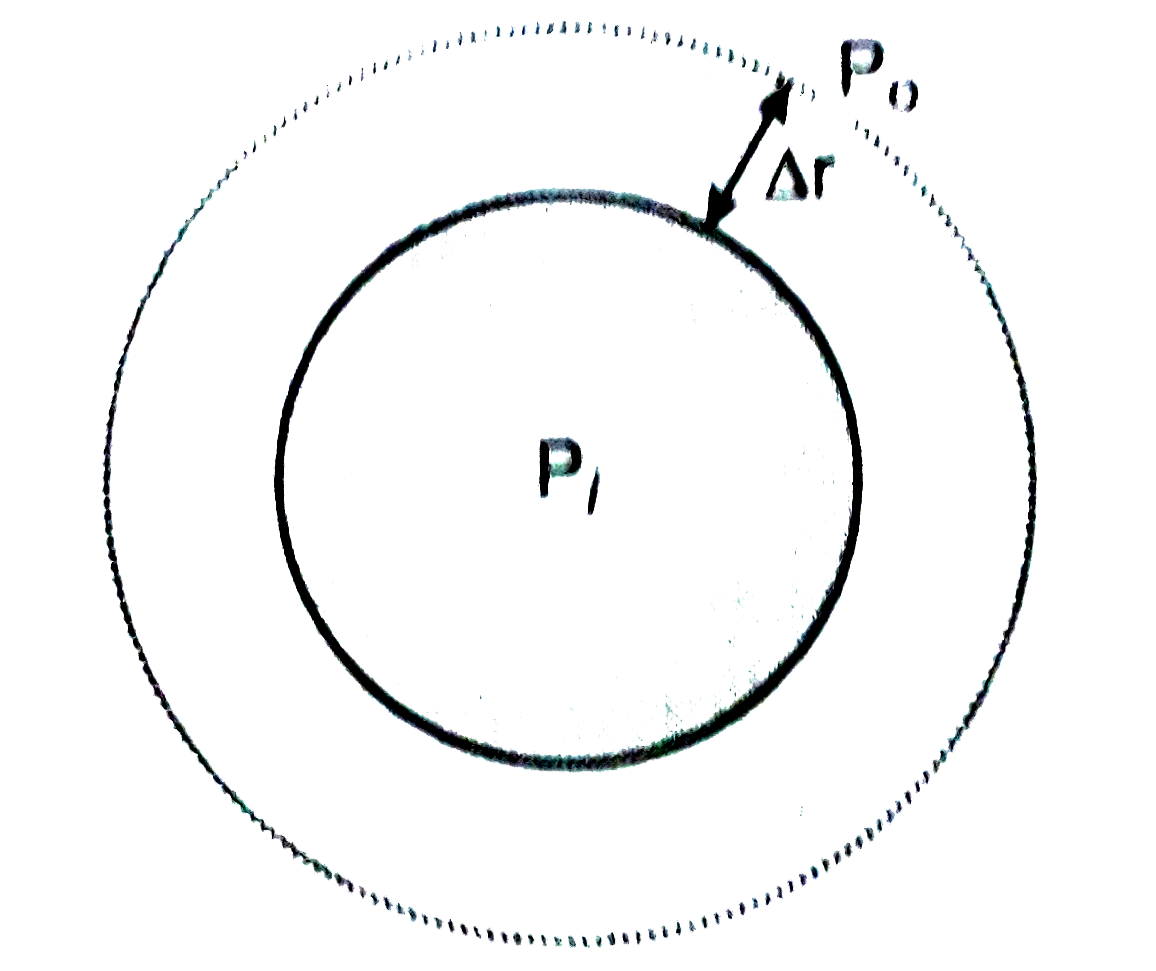
Let, the radius of the drop increase from r to $r = Δ r$ , where $Δ r$ is very small, so that the pressure inside the drop remains almost constant.
Initial surface area $(A_{1}) = 4 π r^{2}$
Final surface area $(A_{2}) = 4 π {(r + Δ r)}^{2}$
$= 4 π (r^{2} + 2 r Δ r + Δ r^{2})$
$= 4 π r^{2} + 8 π r Δ r + 4 π Δ r^{2}$
As $Δ r$ is very small, $Δ r^{2}$ is neglected (i.e., $4 π Δ r^{2} = 0$ )
Increase in surface area (dA)
$= A_{2} - A_{1} = 4 π r^{2} + 8 π r Δ r - 4 π r^{2}$
Increase in surface area (dA)
$= 8 π r Δ r$
Work done to increase the surface area $8 π r Δ r$ is extra surface energy.
$∴ d W = T d A$
$∴ d W = T \times 8 π r Δ r$ ...(i)
This work done is equal to the product of the force and the distace $Δ r .$
Excess Force = Excess Pressure $\times$ Area
$d F = (P_{1} - P_{o}) 4 π r^{2}$
The increase in the radius of the bubble is $Δ r$ .
$d W = d F Δ r = (P_{i} - P_{o}) 4 π r^{2} \times Δ r$ ...(ii)
Comparing equations (i) and (ii), we get
$(P_{i} - P_{o}) 4 π r^{2} \times Δ r = T \times 8 π r Δ r$
$∴ (P_{i} - P_{o}) = 2 T / r$
This is called the Laplace's law of spherical membrane.

Updated on:21/07/2023