Fig. 24.8 shows three events A, B and C. Probabilities of different events are shown in the figure. For instance,
P(A∩B'∩C')=0.18 , P(A'∩B∩C')= 0.06 etc.
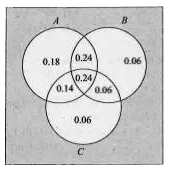
Which of the following is not true ?
A and B are independent
B and C are independent
A and C are independent
A and B∩ C are independent
The correct Answer is:C
Step by step video, text & image solution for Fig. 24.8 shows three events A, B and C. Probabilities of different events are shown in the figure. For instance, P(A nn B' nn C')=0.18 , P(A' nn B nn C')= 0.06 etc. Which of the following is not true ? by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- PROBABILITYBOOK - MCGROW HILL PUBLICATIONCHAPTER - PROBABILITYEXERCISE - Solved Examples (Level 2 straight Objective Correct )
14 Videos
- PROBABILITYBOOK - MCGROW HILL PUBLICATIONCHAPTER - PROBABILITYEXERCISE - Solved Examples (Numerical Answer )
21 Videos
- PROBABILITYBOOK - MCGROW HILL PUBLICATIONCHAPTER - PROBABILITYEXERCISE - Previous Years B-Architecture Entrance Examination Papers
21 Videos
- PERMUTATIONS AND COMBINATIONS BOOK - MCGROW HILL PUBLICATIONCHAPTER - PERMUTATIONS AND COMBINATIONS EXERCISE - Questions from Previous Years. B-Architecture Entrance Examination Papers
17 Videos
- PROGRESSIONSBOOK - MCGROW HILL PUBLICATIONCHAPTER - PROGRESSIONSEXERCISE - Questions from Previous Years. B-Architecture Entrance Examination Papers
25 Videos
Similar Questions
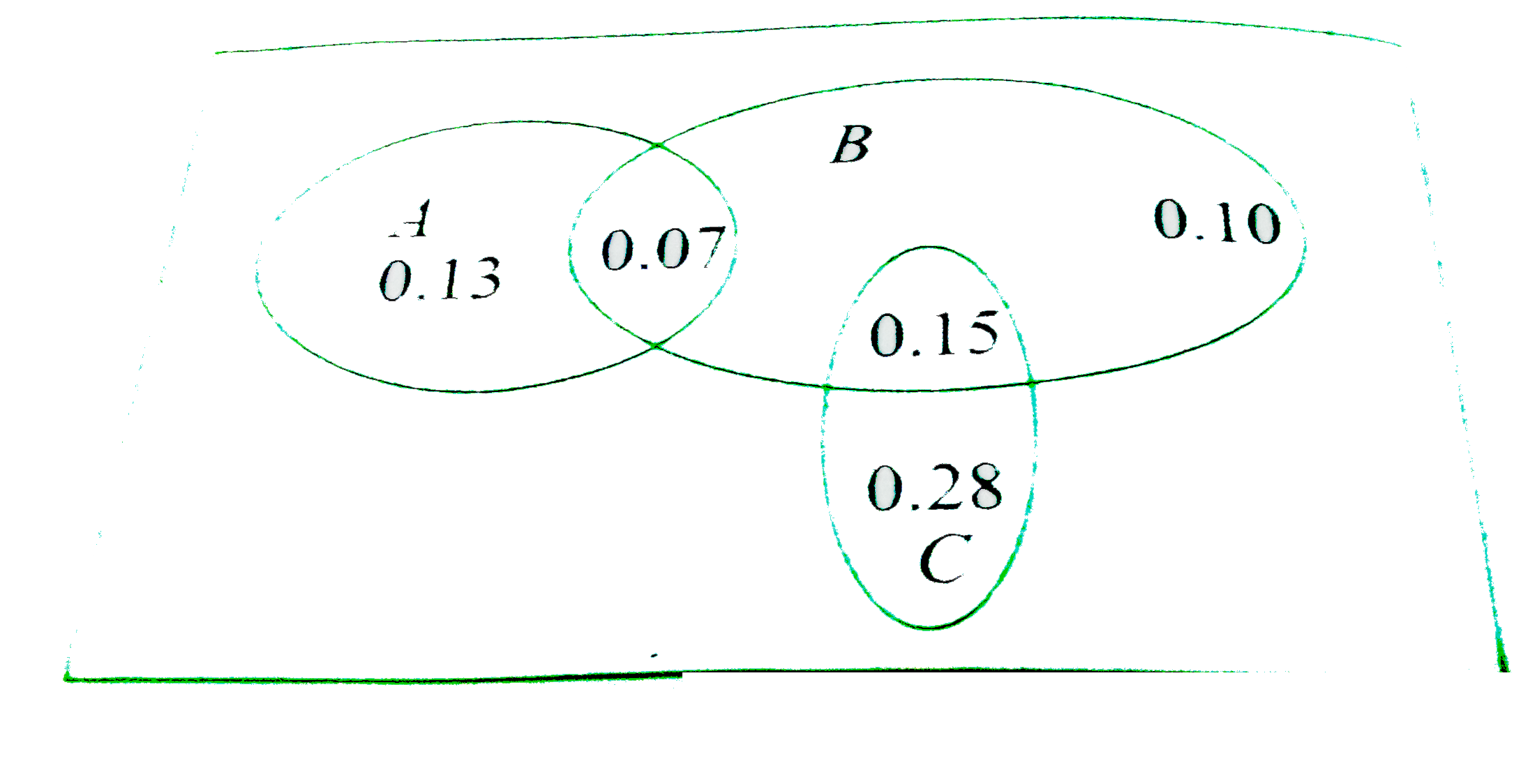
The following Venn diagram shows three events, A, B, and C, and also the probabilities of the various intersections.
Determine
(a) P(A)
(b) P(B∩¯¯¯C)
(c ) P(A∪B)
(d) P(A∩¯¯¯B)
(e) P(B∩C)
(f) Probability of the event that exactly one of A, B, and C occurs.
View SolutionProve the following A∩(B−C)=(A∩B)−(A∩C)
View Solution
Knowledge Check
Fig. 24.8 shows three events A, B and C. Probabilities of different events are shown in the figure. For instance,
P(A∩B'∩C')=0.18 , P(A'∩B∩C')= 0.06 etc.

Which of the following is not true ?AA and B are independentBB and C are independentCA and C are independentDA and B∩C are independentLet A,B and C be sets such that A∩B⊆C and A∩B≠ϕ. Then which of the following statements is not true?
AB∩C≠ϕBIf (A−B)⊆C, then A⊆CCC(∪A)∩(C∪B)=CDIf (A−C)⊆B, then A⊆BFor two given events A and B P(A∩B)=
ANot less than P(A) + P(B) -1BNot greater than P(A) + P(B)CEqual to P(A)+P(B)−P(A∪B)DAll of the above
Event A and B are such that P(A)=0.5,P(B)=0.25 and P(A∩B)=0.125. Find P(A'∩B').
View SolutionIf A, B and C be sets. Then, show that A∩(B∪C)=(A∩B)∪(A∩C).
View SolutionIf P(A) = 0.7, P(B) = 0.4, P(A∩B) = 0.3, then P(A∩B')=
View SolutionIf events are independents and P(A)=13,P(B)=13 and P(C)=14, then P(A'∩B'∩C') is equal to
View SolutionIf A and B are events sucn that P(A)=0.3P(B)=0.2 and P(A∩B)=0.1 then P(¯¯¯A∩B)=?
View Solution

